A rod of length has a mass of . It is hinged at one end of the rod at a distance of below the water surface.
a.What must be the weight of a block that is attached to the other end of the rod so that of the rod's length is under water?
b. Find the magnitude and direction of the force exerted by the hinge on the rod. The specific gravity of the material of rod is
The rod makes angle with the vertical shape cose and sin Let .a. be the area of cross section of the rod.
weight of the rod mg (6) 500 g N, Force of buoyancy F = (5a) 1000 g N
IF W N be the weight at the end (required), then taking moments about the hinge and equating it to zero
for rotatory equilibrium),(5000ag)2.5 sin )-(3000 ag)3 sin -W (6 sin )=0
Since sin ,so cancelling ag sin throughtout.
12500-9000-
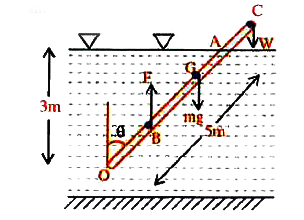
Now, the mass of the rod=12 kg
wt. or W=22.837 N
ii)Now, upthrust F= g=20 gN
IFR be the verical reaction (up) at the hinge, then for vertical equlibrium of the rod, R+F-mg - W=0
R=-5.67kg 2t.(or) -55.57N
The -Ve sign implies that ,the reaction is downwards of magnitude 55.57N
Similar Questions
A uniform thin rod of mass m and length R is placed normally on surface of earth as shown. The mass of earth is M and its radius R. Then the magnitude of gravitational force exerted by earth on the rod is

View SolutionA particle of mass m is situated at a distance d from one end of a rod of mass M and length L as shown in diagram. Find the magnitude of the gravitational force between them
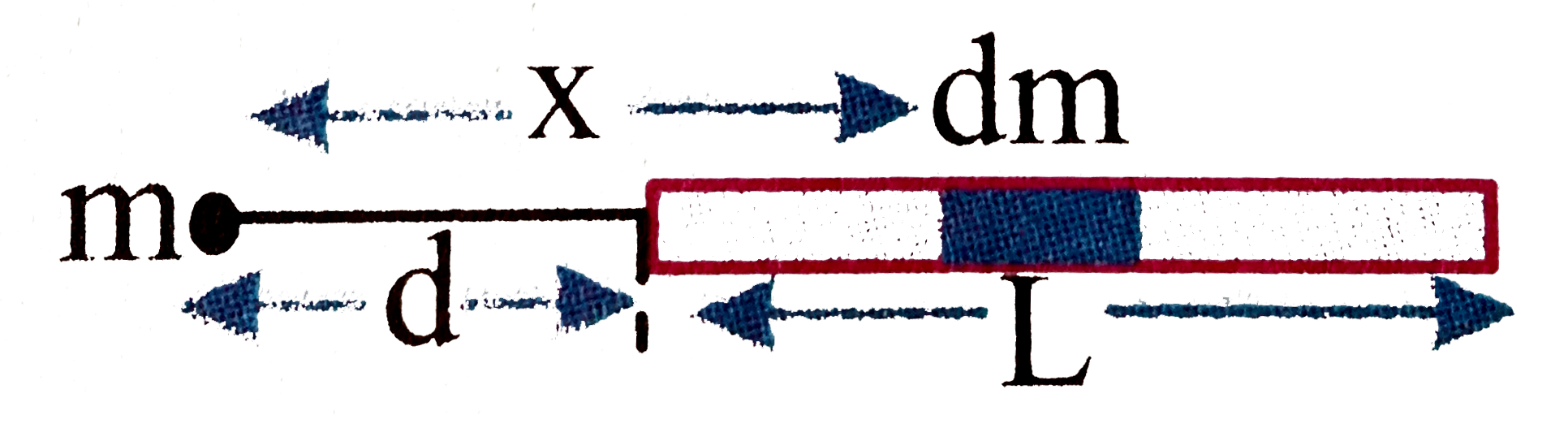
View SolutionA mass m is at a distance a from one end of a uniform rod of length l and mass M. Find the gravitational force on the mass due to the rod.

View SolutionA rod is of length 3 m and its mass acting per unit length is directly proportional to distance x from its one end. The centre of gravity of the rod from that end will be at
View SolutionA uniform rod ofmass M and length L is hanging from its one end free to rotate in a veritcal plane.A small ball of equal mass is attached of the lowe end as shown. Time period of small oscillations of the rod is

View SolutionA mass m is at a distance a from one end of a uniform rod of length l and mass M. Find the gravitational force on the mass due to tke rod.

View SolutionA rod AB of mass M and length L is shown in figure. End A of rod is hinged and end B is lying on the ground. Find the Horizontal component of the force applied by the hinge

View SolutionA rod of length 6m has specific gravity ρ(=25/36). One end of the rod is tied to a 5m long rope, which in turn is tied to the floor of a pool 10m deep, as shown. Find the length (in m) of the part of rod which is out of water.

View SolutionA ball of mass m is attached to one end of a light rod of length l, the other end of which is hinged. What minimum velocity v should be imparted to the ball downwards, so that it can complete the circle ?

View SolutionThere is a rod of length l and mass m. It is hinged at one end to the ceiling. The period of small oscillation is
View Solution