If the mass of the earth is M, its radius is R and gravitational constant G, then the speed required to be given to a projectile so that it does not return to earth is
√GMR
√2GM2
√GRM
√GRM
The correct Answer is:B
To find the speed required to be given to a projectile so that it does not return to Earth, we can use the principles of gravitational potential energy and kinetic energy. Here’s a step-by-step solution:
Step 1: Understand the Problem
We need to determine the speed (v) that a projectile must have to escape Earth's gravitational pull. This speed is often referred to as the escape velocity.
Step 2: Write Down the Given Data
- Mass of the Earth: M
- Radius of the Earth: R
- Gravitational constant: G
Step 3: Use the Conservation of Energy
The total mechanical energy at the surface of the Earth must equal the total mechanical energy when the projectile is at an infinite distance (where potential energy is zero).
The initial total energy (E_initial) is the sum of kinetic energy (KE) and gravitational potential energy (PE):
Einitial=KE+PE=12mv2−GMmR
Where:
- m is the mass of the projectile
- v is the speed of the projectile
The final total energy (E_final) when the projectile is far away (at infinity) is:
Efinal=0
Thus, we set up the equation:
12mv2−GMmR=0
Step 4: Rearrange the Equation
From the equation above, we can isolate the kinetic energy term:
12mv2=GMmR
Step 5: Cancel the Mass of the Projectile
Since m appears on both sides of the equation, we can cancel it out (assuming m≠0):
12v2=GMR
Step 6: Solve for v
Now, multiply both sides by 2 to isolate v2:
v2=2GMR
Taking the square root of both sides gives us the escape velocity:
v=√2GMR
Final Answer
The speed required to be given to a projectile so that it does not return to Earth is:
v=√2GMR
---
We need to determine the speed (v) that a projectile must have to escape Earth's gravitational pull. This speed is often referred to as the escape velocity.
- Mass of the Earth: M
- Radius of the Earth: R
- Gravitational constant: G
The total mechanical energy at the surface of the Earth must equal the total mechanical energy when the projectile is at an infinite distance (where potential energy is zero).
Einitial=KE+PE=12mv2−GMmR
Where:
- m is the mass of the projectile
- v is the speed of the projectile
Efinal=0
Thus, we set up the equation:
12mv2−GMmR=0
From the equation above, we can isolate the kinetic energy term:
12mv2=GMmR
Since m appears on both sides of the equation, we can cancel it out (assuming m≠0):
12v2=GMR
Now, multiply both sides by 2 to isolate v2:
v2=2GMR
Taking the square root of both sides gives us the escape velocity:
v=√2GMR
The speed required to be given to a projectile so that it does not return to Earth is:
v=√2GMR
Similar Questions
If M be the mass of the earth, R its radius (assumed spherical) and G gravitational constant, then the amount of work that must be done on a body of mass m, so that it completely escapes from the gravity of the earth of the earth is given by
View SolutionA particle of mass ‘m’ is kept at rest at a height 3R from the surface of earth, where ‘R’ is radius of earth and ‘M’ is mass of earth. The minimum speed with which it should be projected, so that it does not return back, is (g is acceleration due to gravity on the surface of earth)
View Solution
Knowledge Check
If M is the mass of the earth and R its radius, then ratio of the gravitational acceleration and the gravitational constant is
AR2EMEBMER2ECMER2EDMERE
If M is the mass of the earth and R its radius, the radio of the gravitational acceleration and the gravitational constant is
View SolutionA particle of mass m is thrown upwards from the surface of the earth, with a velocity u. The mass and the radius of the earth are, respectively, M and R. G is gravitational constant g is acceleration due to gravity on the surface of earth. The minimum value of u so that the particle does not return back to earth is
View SolutionA particle is kept at rest at a distance R (earth's radius) above the earth's surface. The minimum speed with which it should be projected so that is does not return is
View SolutionIf the earth were to spin faster, acceleration due to gravity at the poles :
View SolutionA uniform thin rod of mass m and length R is placed normally on surface of earth as shown. The mass of earth is M and its radius is R. If the magnitude of gravitational force exerted by earth on the rod is ηGMm12R2 then η is
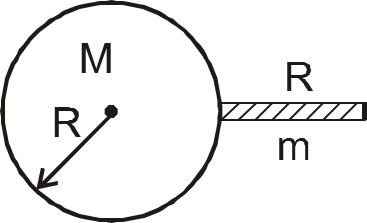
View SolutionA uniform thin rod of mass m and length R is placed normally on surface of earth as shown. The mass of earth is M and its radius is R. If the magnitude of gravitational force exerted by earth on the rod is ηGMm12R2 then η is

View SolutionA tuning is dug along the diameter of the earth. There is particle of mass m at the centre of the tunel. Find the minimum velocity given to the particle so that is just reaches to the surface of the earth. (R= radius of earth)
View Solution
