values of the acceleration ⋅⋅x of a particle moving in simple harmonic motion as a funcation of its displacement x are given in the table below.
∣∣∣..x(mm/s2)1680−8−16x(mm)−4−2024∣∣∣
The motion is
1πs
2πs
π2s
πs
The correct Answer is:D
Step by step video solution for values of the acceleration overset(* *)(x) of a particle moving in simple harmonic motion as a funcation of its displacement x are given in the table below. |(ddot(x)(mm//s^(2)),16,8,0,-8,-16),(x(mm),-4,-2,0,2,4)| The motion is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Similar Questions
Values of the acceleration A of a particle moving in simple harmonic motion as a function of its displacement x are given in the table below.
∣∣∣A(mms−2)1680−8−16x(mm)−4−2024∣∣∣
The pariod of the motion is
View SolutionThe total energy of a particle having a displacement x, executing simple harmonic motion is
View Solution
Knowledge Check
The displacement of a particle executing simple harmonic motion is given by y=4sin(2t+ϕ). The period of oscillation is
A2/πBπ/2CπD2π
The x−t graph of a particle undergoing simple harmonic motion is shown in figure. Acceleration of particle at t=4/3s is
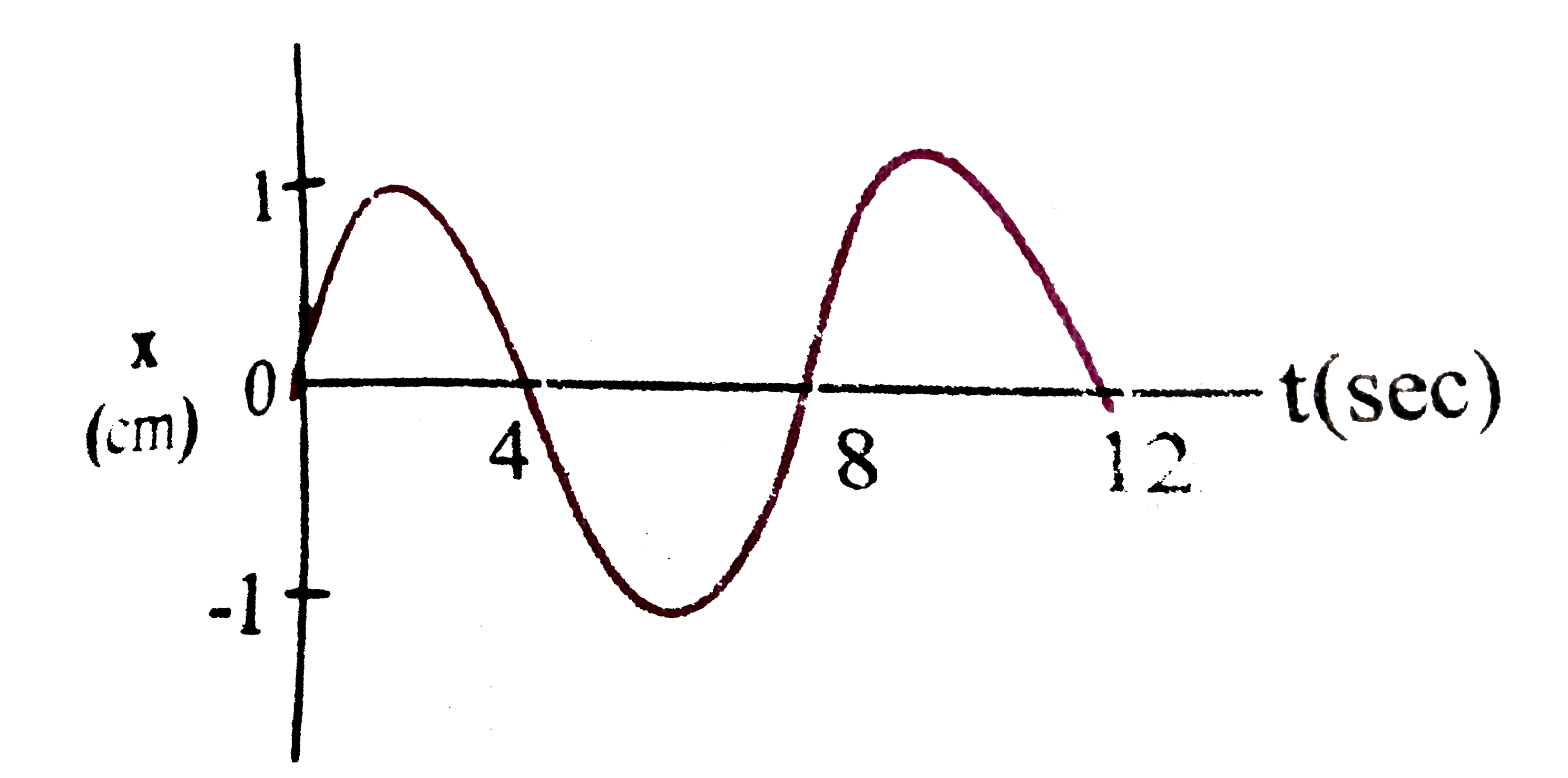
View SolutionThe x−t graph of a particle undergoing simple harmonic motion is shown in figure. Acceleration of particle at t=4/3s is

View SolutionThe acceleration-displacement (a−X) graph of a particle executing simple harmonic motion is shown in the figure. Find the frequency of oscillation.

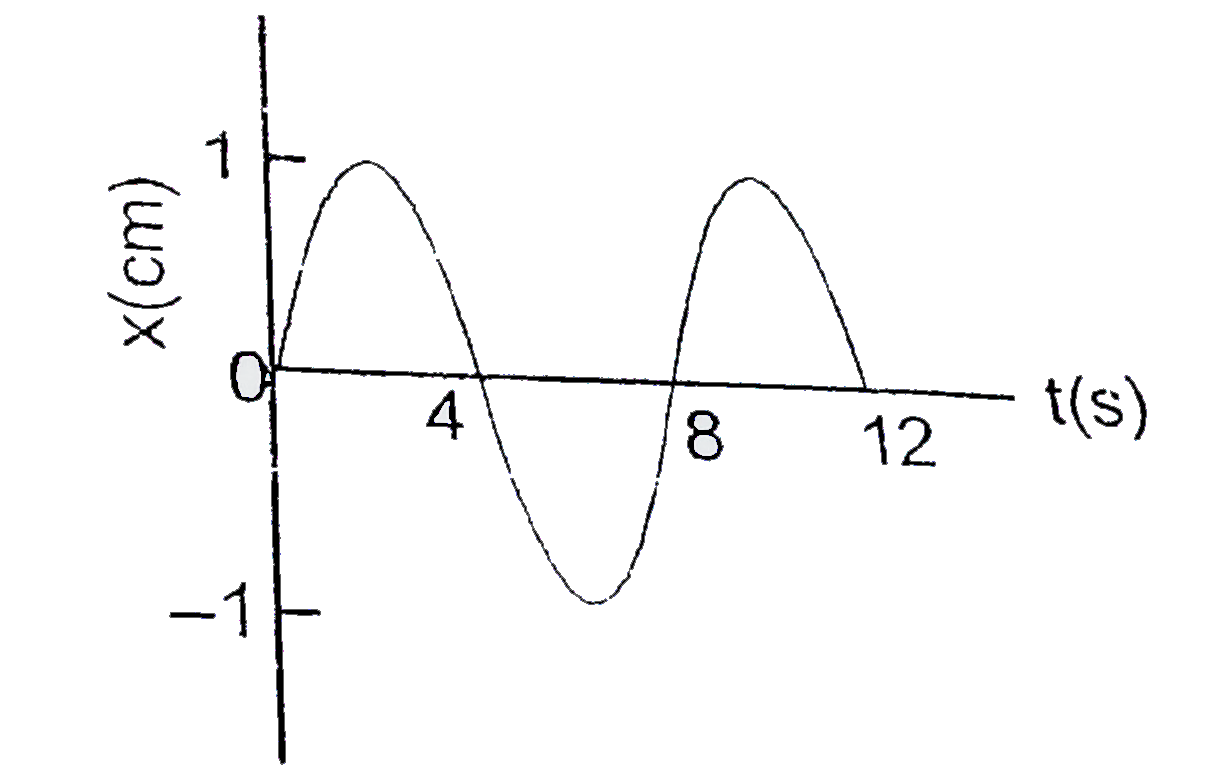
View SolutionThe x-t graph of a particle undergoing simple harmonic motion is shown below. The acceleration of the particle at t=23s is

View SolutionThe x−t graph of a particle undergoing simple harmonic motion is shown below. The accelertion of the particle at t=4/3s is
View SolutionThe maximum velocity of a particle, excuting simple harmonic motion with an amplitude 7mm, is 4.4m/s The period of oscillation is.
View SolutionA particle moving along the X-axis executes simple harmonic motion, then the force acting on it is given by
where, A and K are positive constants.
View Solution