The cross-section area and length of cylindrical conductor are A and l respectively. The specific conductivity varies as σ(x)=σ0l√x, where x is the distance along the axis of the cylinder from one of its ends. Compute the resistance of the system along the cylindrical axis.
3√l2Aσ0
2l3Aσ0
2√l3Aσ0
√lAσ0
The correct Answer is:C
To compute the resistance of a cylindrical conductor with varying specific conductivity, we will follow these steps:
Step 1: Understand the problem
We are given a cylindrical conductor with:
- Cross-sectional area A
- Length l
- Specific conductivity σ(x)=σ0l√x, where x is the distance from one end of the cylinder.
Step 2: Relate resistance to resistivity
The resistance R of a conductor can be expressed as:
R=ρlA
where ρ is the resistivity. Since resistivity is the inverse of conductivity, we have:
ρ(x)=1σ(x)=√xσ0l
Step 3: Consider a small element of the conductor
We will consider a small element of the conductor of length dx at a distance x from one end. The resistance dR of this small element is given by:
dR=ρ(x)dxA=√xσ0l⋅dxA
Step 4: Integrate to find total resistance
To find the total resistance R, we need to integrate dR from x=0 to x=l:
R=∫l0dR=∫l0√xσ0lAdx
Step 5: Solve the integral
The integral can be solved as follows:
R=1σ0lA∫l0√xdx
The integral ∫l0√xdx can be computed:
∫√xdx=23x3/2
Evaluating from 0 to l:
∫l0√xdx=23l3/2
Step 6: Substitute back into the equation for resistance
Substituting the result of the integral back into the equation for R:
R=1σ0lA⋅23l3/2
This simplifies to:
R=23⋅l3/2σ0A
Final Result
Thus, the resistance of the cylindrical conductor is:
R=23⋅l3/2σ0A
We are given a cylindrical conductor with:
- Cross-sectional area A
- Length l
- Specific conductivity σ(x)=σ0l√x, where x is the distance from one end of the cylinder.
The resistance R of a conductor can be expressed as:
R=ρlA
where ρ is the resistivity. Since resistivity is the inverse of conductivity, we have:
ρ(x)=1σ(x)=√xσ0l
We will consider a small element of the conductor of length dx at a distance x from one end. The resistance dR of this small element is given by:
dR=ρ(x)dxA=√xσ0l⋅dxA
To find the total resistance R, we need to integrate dR from x=0 to x=l:
R=∫l0dR=∫l0√xσ0lAdx
The integral can be solved as follows:
R=1σ0lA∫l0√xdx
The integral ∫l0√xdx can be computed:
∫√xdx=23x3/2
Evaluating from 0 to l:
∫l0√xdx=23l3/2
Substituting the result of the integral back into the equation for R:
R=1σ0lA⋅23l3/2
This simplifies to:
R=23⋅l3/2σ0A
Thus, the resistance of the cylindrical conductor is:
R=23⋅l3/2σ0A
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - LECTURE SHEET (EXERCISE- II LEVEL- II (ADVANCED) (Linked Comprehension Type Questions))
2 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - LECTURE SHEET (EXERCISE- II LEVEL- II (ADVANCED) (Matrix Matching Type Questions))
1 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - LECTURE SHEET (EXERCISE- II LEVEL-I (MAIN) (Straight Objective Type Questions))
14 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PROBLEMS (LEVEL-II)
27 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
42 Videos
Similar Questions
A current is flowing through a cylinderical conductor of radius R, such that current density at any cross-section is given by J=J0(1−rR), where r is radial distance from axis of the cylinder .Calculate the total current through the cross-section of the conductor.
View SolutionThe resistivity of cylindrical conductor carrying steady current along its length varies linearly with the distance from the current carrying end as given by ρ=ρ0(1+xl) where l is the length of the conductor and x is the distance from the current entry and. ρo is a positive constant.
View SolutionThe density of a rod of length L varies as ρ=A+Bx where x is the distance from the left end. Locate the centre of mass.

View SolutionA long cylindrical conductor of radius R having a cylindrical cavity of radius R/2 through out its length is lying with its axis parallel to x-axis. The distance between the axes of the cylinder and the cavity length in the positive x direction. The across sectional view of the cylinder is shown in the figure
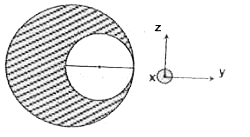
The magnetic induction at point on the cavity is
View SolutionA long cylindrical conductor of radius R carries a current i as shown in the figure. The current density J varies across the cross-section as J=kr2, where, k is a constant. Find an expression for the magnetic field B at a distance r(<R) from the axis

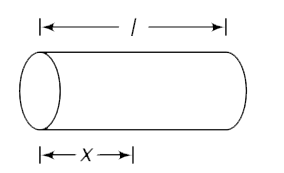
View SolutionA cylindrical conductor has length l and area of cross section A. Its conductivity changes with distance (x) from one of its ends as σ=σ0lx.[σ0is a constant]. Calculate electric field inside the conductor as a function of x, when a cell of emf V is connected across the ends.
View SolutionA long cylinder of uniform cross section and radius R is carrying a current i along its length and current density is uniform cross section and radius r in the cylinder parallel to its length. The axis of the cylinderical cavity is separated by a distance d from the axis of the cylinder. Find the magnetic field at the axis of cylinder.
View SolutionA conducting cylindrical rod of uniform cross-sectional area is kept between two large chambers which are at temperatures 100∘C and 0∘C, respectively. The cconductivety of the rod increases with x, where x is distance from 100∘C end. The temperature profile of the rod in steady -state will be as

View SolutionA cylinder of radius R and length l is made up of substance whose thermal conductivity K varies with the distance x from the axis as K=K1x+K2. Determine the the effective thermal conductivity between the flat faces of the cylinder.
View SolutionThe centre of mass of a non uniform rod of length L, whose mass per unit length varies as ρ=k.x2L where k is a constant and x is the distance of any point from one end is (from the same end)
View Solution
