The Electric field is given by , here the test charge should be
(a) Infinitesimally small and positive
(b) Infinitesimally small and negative
only a
only .b.
a (or )b
neither .a. or .b.
The correct Answer is:A
To solve the question regarding the nature of the test charge q0 in the expression for the electric field →E=→Fq0, we can follow these steps:
Step 1: Understanding the Electric Field
The electric field →E at a point in space is defined as the force →F experienced by a unit positive charge placed at that point. The formula →E=→Fq0 indicates that the electric field is derived from the force acting on a charge q0.
Step 2: Nature of the Test Charge
To accurately measure the electric field without influencing it, the test charge q0 must be:
- Infinitesimally small: This ensures that the test charge does not disturb the existing electric field created by other charges.
- Positive: By convention, the electric field is defined in terms of the force on a positive test charge. This means that the direction of the electric field is taken to be the direction of the force on a positive charge.
Step 3: Conclusion
Given the above understanding, the correct choice for the test charge q0 is:
- (a) Infinitesimally small and positive.
Thus, the answer to the question is that the test charge q0 should be infinitesimally small and positive.
The electric field →E at a point in space is defined as the force →F experienced by a unit positive charge placed at that point. The formula →E=→Fq0 indicates that the electric field is derived from the force acting on a charge q0.
To accurately measure the electric field without influencing it, the test charge q0 must be:
- Infinitesimally small: This ensures that the test charge does not disturb the existing electric field created by other charges.
- Positive: By convention, the electric field is defined in terms of the force on a positive test charge. This means that the direction of the electric field is taken to be the direction of the force on a positive charge.
Given the above understanding, the correct choice for the test charge q0 is:
- (a) Infinitesimally small and positive.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBook:AAKASH SERIESChapter:ELECTRIC CHARGES AND FIELDSExercise:Exercise-II
Explore 56 Videos
- ELECTRIC CHARGES AND FIELDSBook:AAKASH SERIESChapter:ELECTRIC CHARGES AND FIELDSExercise:Practice Exercise
Explore 57 Videos
- ELECTRIC CHARGES AND FIELDSBook:AAKASH SERIESChapter:ELECTRIC CHARGES AND FIELDSExercise:Assess Your Self
Explore 13 Videos
- DUAL NATURE OF RADIATION AND MATTERBook:AAKASH SERIESChapter:DUAL NATURE OF RADIATION AND MATTERExercise:PRACTICE EXERCISEX
Explore 42 Videos
- ELECTRIC FIELD AND POTENTIALBook:AAKASH SERIESChapter:ELECTRIC FIELD AND POTENTIALExercise:PROBLEMS (LEVEL-II)
Explore 26 Videos
Similar Questions
If electric field around a surface is given by ∣∣→E∣∣=Q∈ε0|A| where 'A' is the normal area of surface and Q∈ is the charge enclosed by the surface. This relation of gauss's law is valid when
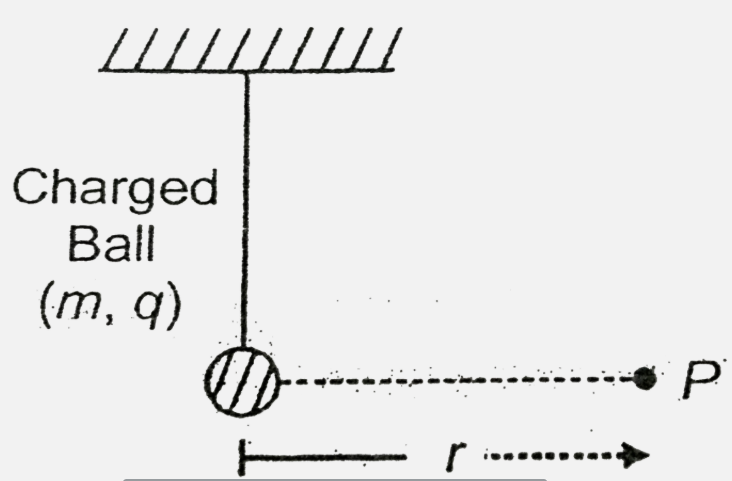
View SolutionThe electric field at point P due to a charged ball is given by
Ep=14πε0qr2
To measure 'E' at point P, A test charge q0 is placed at point P and measure electric force F upon the test charge. Check whether Fq0 is equal to 14πε0qr2 or not .
View SolutionAn electric field is defined in terms of q0 a small positive charge. If the definition was in terms of a small negative charge of the same magnitude, then compared to the original field the newly defined electric field.
(a) would point in the same direction and have the same magnitude.
(b) would point in the opposite direction and have the same magnitude.
(c ) would point in the same direction and have the different magnitude.
(d) would point in the opposite direction and have the different magnitude.
View SolutionThe electric field of a plane electromagnetic wave is given by
→E=E0ˆicos(kz)cos(ωt)
The corresponding magnetic field →B is then given by :
View SolutionMagnetic force on a charged particle is given by →Fm=q(→v×→B) and electrostatic force →Fe=q→E. A particle having charge q = 1C and mass 1 kg is released from rest at origin. There are electric and magnetic field given by →E=(10ˆi)N/Cforx=1.8m and →B=−(5ˆk)T for 1.8m≤x≤2.4m
A screen is placed parallel to y-z plane at x=3m. Neglect gravity forces.
The speed with which the particle will collide the screen is
View SolutionIn fiinding the electric field using Gauses law the formula ∣∣→E∣∣=qenc∈0|A| is applicable. In the Formula. ∈0 is permittivity of free space, A is the area of Gaussian surface and qenc is charge enclosed by the Gaussion surface. This equation can be used in which of the following situation?
View SolutionA uniform electric field E0 is directed along positive y-driection. Find the change in electric potential energy of a positive test charge q0 when it is displaced in this field from yi=a to yf=2a along the y-axis.
View SolutionA uniform electric field E0 is directed along positive y-driection. Find the change in electric potential energy of a positive test charge q0 when it is displaced in this field from yi=a to yf=2a along the y-axis.
View SolutionElectric field in space is given by −−→E(t)=E0i+j√2cos(ωt+Kz). A positively charged particle at (0,0,πK) is given velocity v0ˆk at t = 0. Direction of force acting on particle is
View SolutionMagnetic force on a charged particle is given by →Fm=q(→v×→B) and electrostatic force →Fe=q→E. A particle having charge q = 1C and mass 1 kg is released from rest at origin. There are electric and magnetic field given by →E=(10ˆi)N/Cforx=1.8m and →B=−(5ˆk)T for 1.8m≤x≤2.4m
A screen is placed parallel to y-z plane at x=3m. Neglect gravity forces.
y-coordinate of particle where it collides with screen (in meters) is
View Solution