The path of a charged particle projected into a uniform transverse electric field is
circle
hyperbola
parabola
ellipse
The correct Answer is:C
To solve the question regarding the path of a charged particle projected into a uniform transverse electric field, we can follow these steps:
Step 1: Understand the Forces Acting on the Charged Particle
When a charged particle is placed in an electric field, it experiences a force due to the electric field. The force F acting on a charged particle with charge Q in an electric field E is given by:
F=Q⋅E
This force acts in the direction of the electric field.
Step 2: Analyze the Motion of the Charged Particle
If the charged particle is projected into a uniform transverse electric field, it means that the initial velocity of the particle is perpendicular to the direction of the electric field. For example, if the electric field is directed vertically (upwards), the particle is projected horizontally.
Step 3: Break Down the Motion into Components
The motion of the charged particle can be analyzed in two dimensions:
- The horizontal motion (along the x-axis) where there is no force acting on the particle, so it moves with constant velocity.
- The vertical motion (along the y-axis) where the particle experiences a constant force due to the electric field, causing it to accelerate.
Step 4: Apply Kinematic Equations
In the horizontal direction (x-axis):
- The displacement x is given by:
x=Vx⋅t
where Vx is the initial horizontal velocity and t is the time.
In the vertical direction (y-axis):
- The displacement y is given by the equation of motion under constant acceleration:
y=12ayt2
where ay=Fm=Q⋅Em is the acceleration due to the electric field.
Step 5: Combine the Equations
Since y depends on t2 and x depends linearly on t, we can eliminate t from these equations. From the horizontal motion, we can express t as:
t=xVx
Substituting this into the vertical motion equation gives:
y=12ay(xVx)2
This equation represents a parabolic relationship between y and x.
Conclusion
The path of the charged particle projected into a uniform transverse electric field is a parabola.
Final Answer
The correct option is parabola.
---
When a charged particle is placed in an electric field, it experiences a force due to the electric field. The force F acting on a charged particle with charge Q in an electric field E is given by:
F=Q⋅E
This force acts in the direction of the electric field.
If the charged particle is projected into a uniform transverse electric field, it means that the initial velocity of the particle is perpendicular to the direction of the electric field. For example, if the electric field is directed vertically (upwards), the particle is projected horizontally.
The motion of the charged particle can be analyzed in two dimensions:
- The horizontal motion (along the x-axis) where there is no force acting on the particle, so it moves with constant velocity.
- The vertical motion (along the y-axis) where the particle experiences a constant force due to the electric field, causing it to accelerate.
In the horizontal direction (x-axis):
- The displacement x is given by:
x=Vx⋅t
where Vx is the initial horizontal velocity and t is the time.
- The displacement y is given by the equation of motion under constant acceleration:
y=12ayt2
where ay=Fm=Q⋅Em is the acceleration due to the electric field.
Since y depends on t2 and x depends linearly on t, we can eliminate t from these equations. From the horizontal motion, we can express t as:
t=xVx
Substituting this into the vertical motion equation gives:
y=12ay(xVx)2
This equation represents a parabolic relationship between y and x.
The path of the charged particle projected into a uniform transverse electric field is a parabola.
The correct option is parabola.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBook:AAKASH SERIESChapter:ELECTRIC CHARGES AND FIELDSExercise:Exercise-II
Explore 56 Videos
- ELECTRIC CHARGES AND FIELDSBook:AAKASH SERIESChapter:ELECTRIC CHARGES AND FIELDSExercise:Practice Exercise
Explore 57 Videos
- ELECTRIC CHARGES AND FIELDSBook:AAKASH SERIESChapter:ELECTRIC CHARGES AND FIELDSExercise:Assess Your Self
Explore 13 Videos
- DUAL NATURE OF RADIATION AND MATTERBook:AAKASH SERIESChapter:DUAL NATURE OF RADIATION AND MATTERExercise:PRACTICE EXERCISEX
Explore 42 Videos
- ELECTRIC FIELD AND POTENTIALBook:AAKASH SERIESChapter:ELECTRIC FIELD AND POTENTIALExercise:PROBLEMS (LEVEL-II)
Explore 26 Videos
Similar Questions
A proton and an α particle having equal kinetic energy are projected in a uniform transverse electric field as shown in figure
View SolutionAssertion If the path of a charged particle in a region of uniform electric and magnetic field is not a circle, then its kinetic energy may remain constant.
Reason In a combined electric and magnetic field region a moving charge experiences a net force
F=qE+q(V×B), where symbols have their usual meanings.
View Solution
Knowledge Check
When a charged particle is projected perpendicular to a uniform magnetic field, it describes a circular path in which :
Aits speed remains constant.Bits velocity remains constant.Cits momentum remains constant.Dits kinetic energy increases.
When a charged particle is projected perpendicular to a uniform magnetic field, it describes a circular path in which:
View SolutionThe path of a charged particle moving in a uniform steady magnetic field cannot be a
View SolutionA proton and an alpha particle projected with same velocity in uniform transverse magnetic field then
View SolutionSTATEMENT-1 : If a charge particle enters into a uniform electric field, then its path must be straight line.
and
STATEMENT -2 : Electrostatic force on a positive charge particle is always in the direction of electric field.
View SolutionThe path of a charged particle in a uniform magnetic field, when the velocity and the magnetic field are perpendicular to each other is a
View SolutionThe acceleration of a charged particle in a uniform electric field is
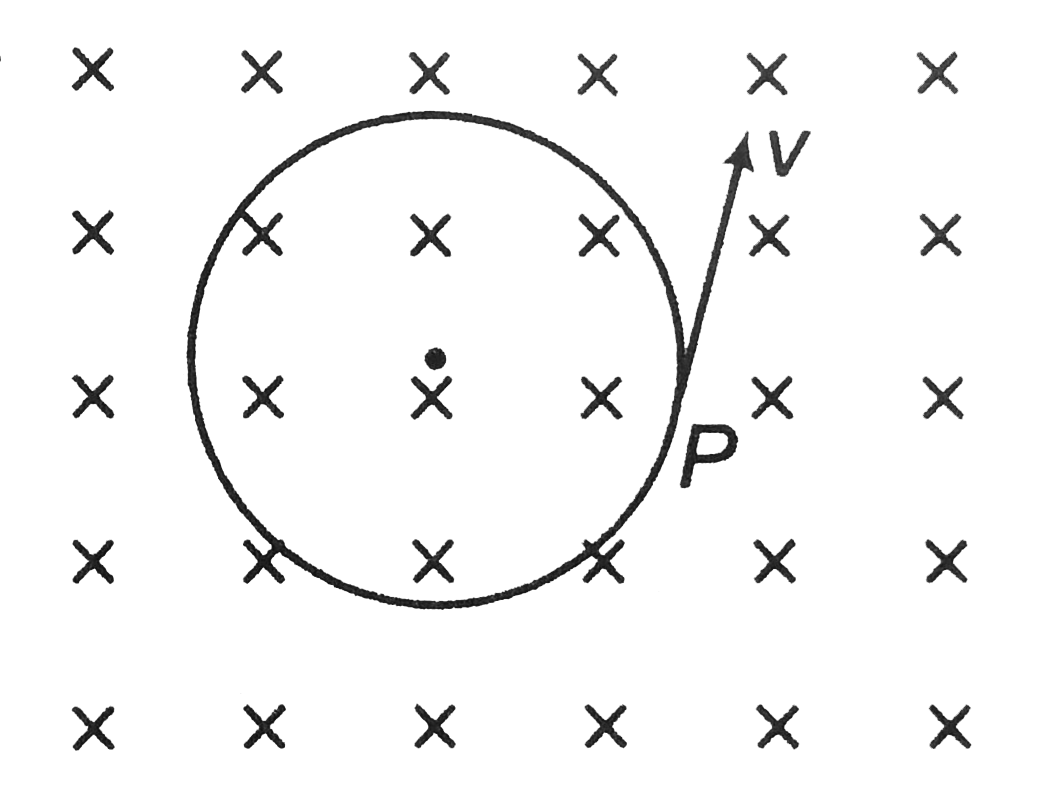
View SolutionA particle having charge of 20μC and mass 20μg moves along as circle of radius 5cm under the action of a magnetif field B=0.1 tesla. When the particle is at P, uniform transverse electric field is switched on an it is found that the particle continues along the tangent with a uniform velocity. Find the electric field
View Solution