A circular disc is rotating about its own axis at uniform angular velocity ω. The disc is subjected to uniform angular retardation by which its angular velocity is decreased to ω/2 during 120 rotations., The number of rotations further made by it before coming to rest is
120
60
40
20
The correct Answer is:C
To solve the problem step by step, we will follow the principles of angular motion.
1. Identify Given Values:
- Initial angular velocity, ω
- Final angular velocity after 120 rotations, ωf=ω2
- Number of rotations during the retardation, θ=120 rotations
2. Convert Rotations to Radians:
- Since we are dealing with angular motion, we need to convert rotations to radians.
- θ=120 rotations=120×2π radians=240π radians
3. Use the Angular Motion Equation:
- We can use the equation of motion for angular displacement:
ω2f=ω2i+2αθ
- Plugging in the values:
(ω2)2=ω2+2α(240π)
- This simplifies to:
ω24=ω2+480πα
4. Rearranging the Equation:
- Rearranging gives:
480πα=ω24−ω2
- This simplifies to:
480πα=−3ω24
- Therefore, we can express angular retardation α:
α=−3ω21920π
5. Finding Further Rotations Until Rest:
- Now, we need to find how many additional rotations the disc makes before coming to rest.
- Initial angular velocity for this phase is ωi=ω2 and final angular velocity ωf=0.
- Using the same angular motion equation:
0=(ω2)2+2αθ′
- Substituting α:
0=ω24+2(−3ω21920π)θ′
6. Rearranging to Find θ′:
- Rearranging gives:
2(−3ω21920π)θ′=−ω24
- This simplifies to:
θ′=ω246ω21920π=1920π24=80π
7. Convert Radians Back to Rotations:
- Converting θ′ back to rotations:
θ′=80π2π=40 rotations
Final Answer:
The number of additional rotations made by the disc before coming to rest is 40 rotations.
---
- Initial angular velocity, ω
- Final angular velocity after 120 rotations, ωf=ω2
- Number of rotations during the retardation, θ=120 rotations
- Since we are dealing with angular motion, we need to convert rotations to radians.
- θ=120 rotations=120×2π radians=240π radians
- We can use the equation of motion for angular displacement:
ω2f=ω2i+2αθ
- Plugging in the values:
(ω2)2=ω2+2α(240π)
- This simplifies to:
ω24=ω2+480πα
- Rearranging gives:
480πα=ω24−ω2
- This simplifies to:
480πα=−3ω24
- Therefore, we can express angular retardation α:
α=−3ω21920π
- Now, we need to find how many additional rotations the disc makes before coming to rest.
- Initial angular velocity for this phase is ωi=ω2 and final angular velocity ωf=0.
- Using the same angular motion equation:
0=(ω2)2+2αθ′
- Substituting α:
0=ω24+2(−3ω21920π)θ′
- Rearranging gives:
2(−3ω21920π)θ′=−ω24
- This simplifies to:
θ′=ω246ω21920π=1920π24=80π
- Converting θ′ back to rotations:
θ′=80π2π=40 rotations
The number of additional rotations made by the disc before coming to rest is 40 rotations.
Similar Questions
A circular disc is rotating about its own axis at an angular velocity ω. If P is exact midpoint of dise between axis and rim of disc then angular velocity of Pis
View SolutionA cylinder of water, is rotating about its own axis with uniform angular velocity ω. The shape of free surface of water will be
View Solution
Knowledge Check
When a disc rotates with uniform angular velocity, which of the following is not true ?
Aa. The sense of rotation remains same.Bb. The orientation of the axis of rotation remains same.Cc. The speed of rotation is non-zero and remains same.Dd. The angular acceleration is non-zero and remains same.
A disc is rotating with angular velocity ω. If a child sits on it, what is conserved?
View SolutionA fly wheel is rotating about its own axis at an angular velocity 11rads−1, its angular velocity in revolution per minute is
View SolutionA disc is rotaing with an angular velocity ω0. A constant retarding torque is applied on it to stop the disc. The angular velocity becomes ω02 after n rotations. How many more rotations will it make before coming to rest ?
View SolutionWhen a disc rotates with uniform angular velocity, which of the following is not true ?
View SolutionA solid sphere is rotating about a diameter at an angular velocity ω. If it cools so that its radius reduces to 1/n of its original value, its angular velocity becomes
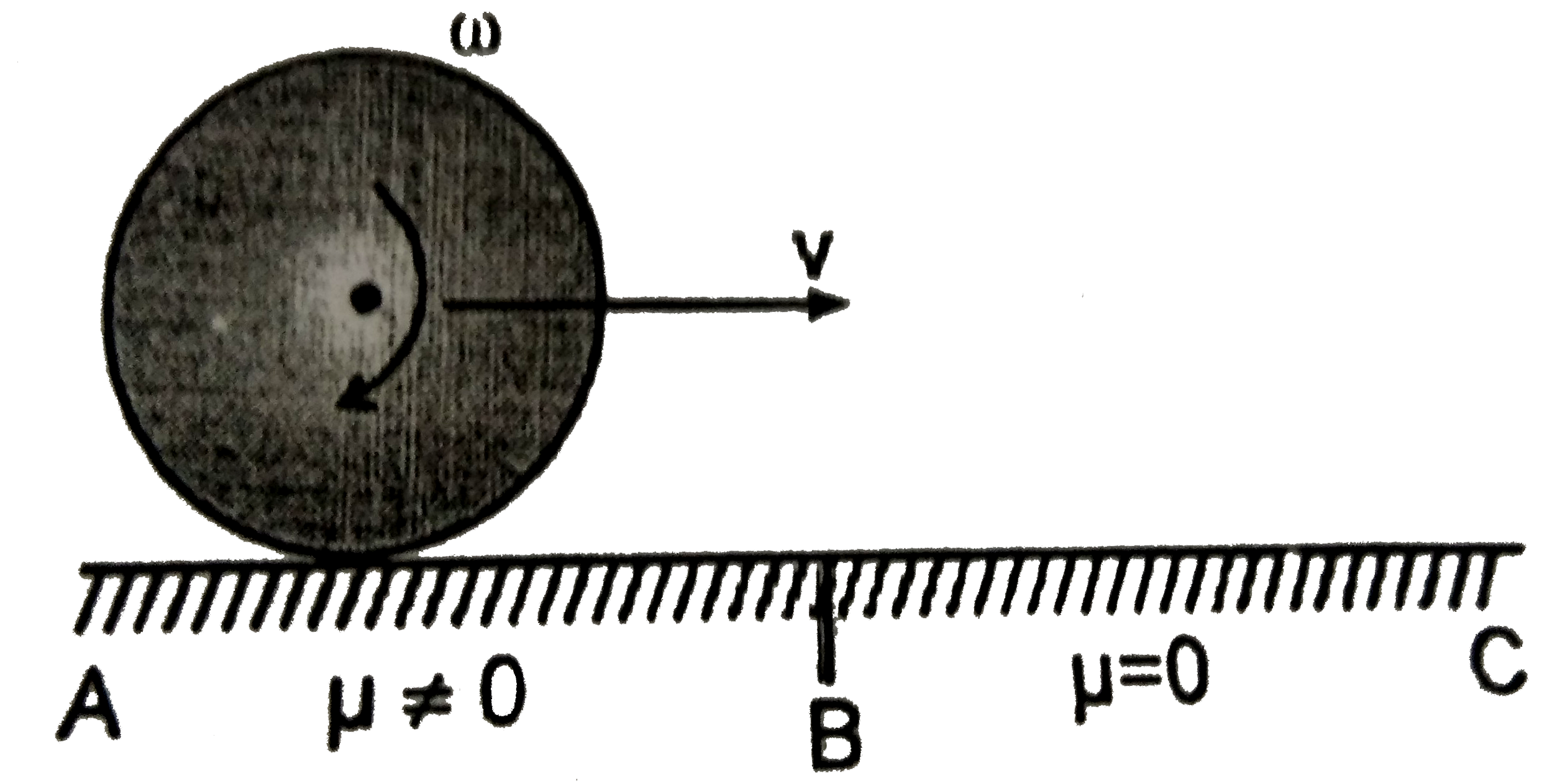
View SolutionAs shown in the figure, a disc of mass m is rolling without slipping a angular velocity ω. When it crosses point B disc will be in :
View SolutionA rod is rotating with angular velocity ω about axis AB. Find cosθ.

View Solution