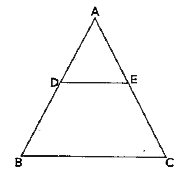
In ΔABC, DE || BC. If AD = 2x - 1, AE = 2x + 5, BD = x - 3 and CE = x - 1, then the value of x is:
8
9
10
11
The correct Answer is:A
To solve the problem, we will use the Basic Proportionality Theorem (also known as Thales' theorem), which states that if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally.
1. Set Up the Proportionality Equation:
Given that DE∥BC, we can set up the following proportion based on the segments created by the parallel line:
ADDB=AEEC
2. Substitute the Given Values:
We know:
- AD=2x−1
- DB=x−3
- AE=2x+5
- EC=x−1
Substituting these values into the proportion gives:
2x−1x−3=2x+5x−1
3. Cross-Multiply to Eliminate the Fractions:
Cross-multiplying leads to:
(2x−1)(x−1)=(2x+5)(x−3)
4. Expand Both Sides:
Expanding the left-hand side:
2x2−2x−x+1=2x2−3x+1
Expanding the right-hand side:
2x2−6x+5x−15=2x2−x−15
5. Set the Equation:
Now we have:
2x2−3x+1=2x2−x−15
6. Simplify the Equation:
Subtract 2x2 from both sides:
−3x+1=−x−15
Rearranging gives:
−3x+x=−15−1
Simplifying further:
−2x=−16
7. Solve for x:
Dividing both sides by -2:
x=8
Final Answer:
The value of x is 8.
---
Given that DE∥BC, we can set up the following proportion based on the segments created by the parallel line:
ADDB=AEEC
We know:
- AD=2x−1
- DB=x−3
- AE=2x+5
- EC=x−1
2x−1x−3=2x+5x−1
Cross-multiplying leads to:
(2x−1)(x−1)=(2x+5)(x−3)
Expanding the left-hand side:
2x2−2x−x+1=2x2−3x+1
Expanding the right-hand side:
2x2−6x+5x−15=2x2−x−15
Now we have:
2x2−3x+1=2x2−x−15
Subtract 2x2 from both sides:
−3x+1=−x−15
Rearranging gives:
−3x+x=−15−1
Simplifying further:
−2x=−16
Dividing both sides by -2:
x=8
The value of x is 8.
Topper's Solved these Questions
- SAMPLE PAPER 03Book:EDUCART PUBLICATIONChapter:SAMPLE PAPER 03Exercise:Section - C
Explore 10 Videos
- SAMPLE PAPER 03Book:EDUCART PUBLICATIONChapter:SAMPLE PAPER 03Exercise:Section - C
Explore 10 Videos
- SAMPLE PAPER 02Book:EDUCART PUBLICATIONChapter:SAMPLE PAPER 02Exercise:SECTION - D
Explore 9 Videos
- SAMPLE PAPER 03 (MATHEMATICS)Book:EDUCART PUBLICATIONChapter:SAMPLE PAPER 03 (MATHEMATICS)Exercise:SECTION D
Explore 12 Videos
Similar Questions
Knowledge Check
In ΔABC D and E are points on sides AB and AC, such that DE || BC. If AD = x,DB = x - 2, AE = x + 2 and EC = x-1 then the value of x is
A4B2C1D8If D and E are points on the sides AB and AC respectively of a triangle ABC such that DE || BC. If AD =x cm, DB =(x-3) cm, AE = ( x+ 3 ) cm and EC = ( x -2) cm, what is the value (in cm. ) of x ?
A3B3.5C4D4.5
In ΔABC,AD=BC. Find x.
View SolutionIn ΔABC,AD=BC. Find x.
View SolutionIn ΔABC , if DE||BC , AD =x , DB =x-2,AE=x+2andEC=x-1 , then the value of x is
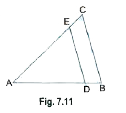
View SolutionIn Fig. 7.11, De||BC. If AD= x, DB= x- 2, AE = x + 2 and EC =x -1, find the value of x.
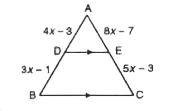
View SolutionIn the given figure in ΔABC,DE∣∣BC so that , AD=(4x−3)cm,AE=(8x−7)cm,BD=(3x−1)cmandCE=(5x−3)cm. Find the value of x.
View SolutionIn figure , DE∣∣BC.If AD=x+1 , DB = x-2 , AE =x+2andEC =x+3 , find the value of x .
View Solution