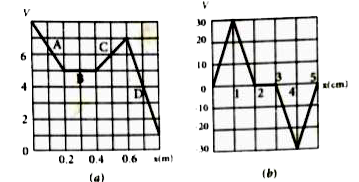
The electrostatic potential is given as a function of x in figure ( a) and (b) . Calculate the corresponding electric fields in regions A, B, C and D . Plot the electric field as a function of x for the figure (b).
 Solution in Tamil
Solution in Tamil
E=−dNdx
(i) Region A
dVdx=−30.2=−15Vi,E=dVdx
∴E=−(−15)=15Vm−1
(ii) Region B
dVdx=00.2=0
(iii) Region C
dVdx=20.2=10
E=−dVdx=(−10)Vm−1
(iv) Region D
dVdx=−60.2=−30
E=−dVdx=−(−30)=30Vm−1
(b) 
Similar Questions
Figure shows a plot of the conservative force F in a unidimensional field. The plot representing the function corresponding to the potential energy (U) in the field is
View SolutionA graph of the x-component of the electric field as a function of x in a region of space is shown in figure. The y−andz−compo≠nts of the electric field are zero in this region. If the electric potential is 10 V at the origin, then the potential at x = 2.0 m is

View SolutionThe variation of electric field on the y-axis as a function of 'y' is best represented by [for the given figure]: A. B C. D.
View SolutionA region in electric field is specified by the potential function V(x)= 4x^(2)
View SolutionA graph of the x-component of the electric field as a function of x in a region of space is shown in figure. The y- and z-components of the electric field are zero in this region. If the electric potential is 10 V at the origin, then the potential at x = 2.0 m is
View SolutionA graph of the x-component of the electric field as a function of x in a region of space is shown in the figure. They y and z-components of the electric field are zero in this region. The electric potential at the origin is 10 V. The value of x for which potential is zero is
View Solution(a) Electric dipole moment
(b) Electric field intensity
(c) Electric potential difference
(d) Electrostatic shielding
View SolutionFigure shows a plot of the conservative force F in a unidimensional field . The plot representing the function corresponding to the potential energy ( U) in the field is
View Solution