If D,E and F are the mid-points of the sides BC,CA and AB, respectively of a ΔABC and O is any point, show that
(i) AD+BE+CF=0
(ii) OE+OF+DO=OA+OB+OCSolution in Kannada
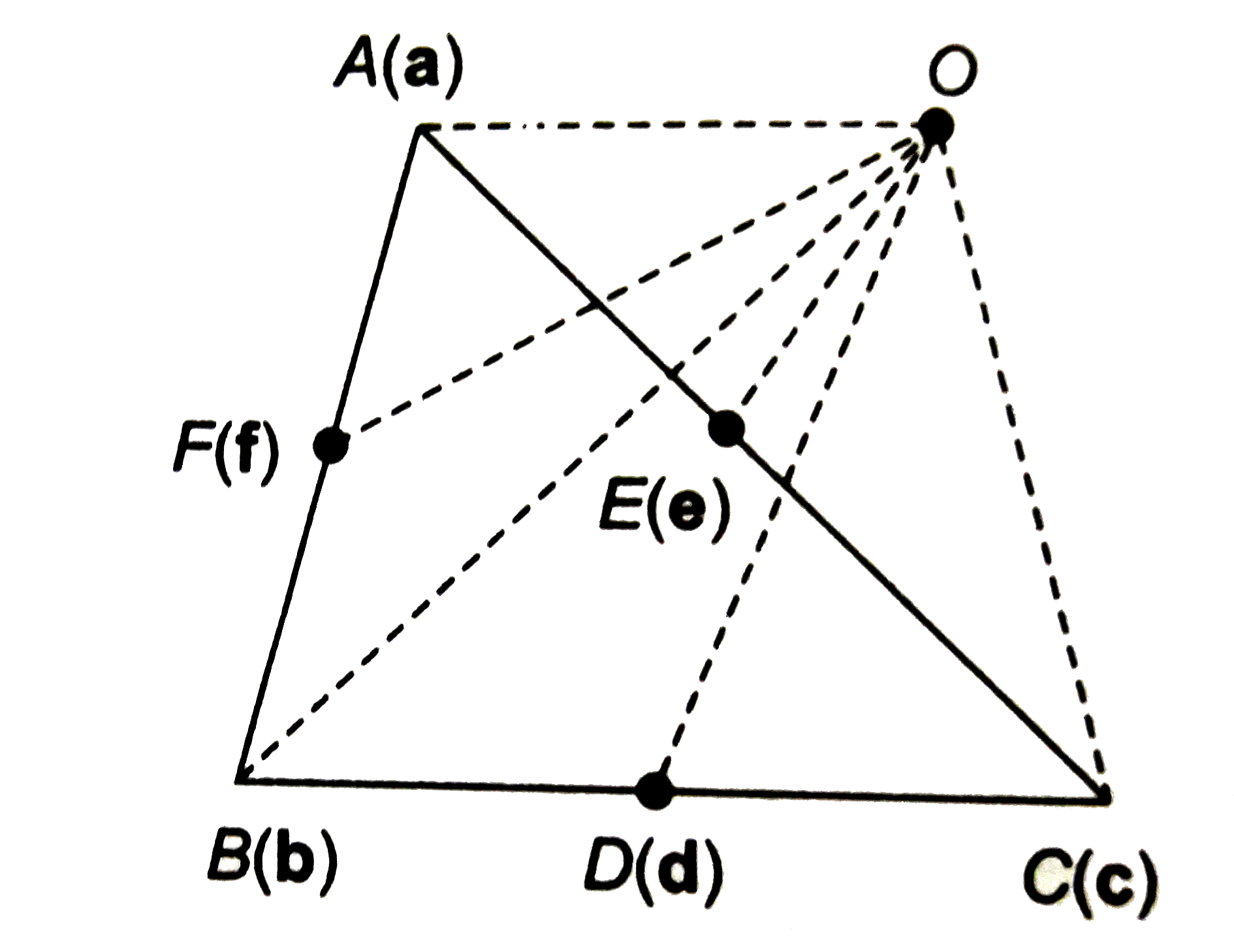
(i) AD+BE+CF==(d−a)+(e−b)+(f−c)
=(d+e+f)−(a+b+c)=0 [usingg eq. (i)]
⇒AD+BE+CF=0
(ii) Here, OE+OF+OD=e+f−d
=c+a2+a+b2−b+c2=a=OA
∴OE+OF+OD=OA
Similar Questions
Dand E are the mid-points of the sides AB and AC of ΔABC and O is any point on side BC. O is joined to A. If P and Q are the mid-points of OB and OC respectively, then DEQP is
View SolutionA point O lies inside a ΔABC. Show that OA+OB+OC>12(AB+BC+CA).
View SolutionIf D,E and F are the mid-points of the sides BC,CA and AB respectively of the ΔABC and O be any point, then prove that OA+OB+OC=OD+OE+OF
View SolutionIf D, E and F be the middle points of the sides BC,CA and AB of the ΔABC, then AD+BE+CF is
View SolutionD, E and F are the mid-points of the sides AB, BC and CA respectively of A ABC. AE meets DF at O. P and Q are the mid-points of OB and OC respectively. Prove that DPOF is a parallelogram.
View SolutionLet O be any point in the interior of ΔABC, prove that :
AB+BC+CA<2(OA+OB+OC)
View SolutionO is any point in the plane of the triangle ABC,AO,BO and CO meet the sides BC,CA nd AB in D,E,F respectively show that ODAD+OEBE+OFCF=1.
View SolutionIf D, E and F be the middle points of the sides BC,CA and AB of the ΔABC, then AD+BE+CF is
View Solution