A cylindrical object of outer diameter 10 cm, height 20 cm and density 8000 kgm−3 is supported by a vertical spring and is half dipped in water as shown in figure. a. Find the elongation of the spring in equilibrium condition. b. If the object is slightly depressed and released, find the time period of resulting oscillations of the object. The spring constant =500Nm−1.
 Solution in Tamil
Solution in Tamil
The correct Answer is:A, B, C
d=10cm
r=5cm
→h=20cm
ρb=8000kgm3=8gm
k=500Nm=500×x103dy≠cm
a. Here F+U=mg[wheeFkx]
→kx+Vρug=mg
→500×103×(x)+(πr2)×(h2)×1×1000
=πr2xh×ρb×1000<br>rarr 500xx10^3xx(x)br>=pir^2xxhxx1000(rho-1/2)<br>=pixx(5)^2xx20xx1000(rho_b-1/2)<br>rarr 50x=pixx25xx2xx(rho_b-1/2)<br>=x=pi(8-0.5)<br>orx=pixx7.5=23.5cm<br>b.IfX→displacementoftheblockformtheequilibriumposition,<br>Driv∈gforce<br>F=kX+Vrho_wxxg<br>rarr ma=kX+pir^2xx(X)xxrho_wxxg<br>=(k+pir^2xxrho_wxxg)X<br>rarr omega^2xx(X)=((k+pir^2+rho_wxxg))/m xx(X)<br>[because a=w^2XinSHM]<br>rarr T=2i m/(k+pir^2+rho_wxxg) ,<br>=2pi(pixx25xx20xx8)/(500+10^3+pix25xx1xx1000)<br>=0.935s.
Topper's Solved these Questions
Similar Questions
Find the accelerationof the moon with respect to the eath from the following data, Distance between the earth and the moon =3.85×105 km and the time taken by the moon to complete one revolution around the earth =27.3days
View SolutionA body slipping on a rough horizontal plane moves wioth a deceleationof 4.0ms. What is cofficient of kineic friction between the block and the plane?
View SolutionA cylindrical object of outer diameter 20 cm and mass 2 kg flots in wter with its axis vertical. If it si lightly depressed and then released, find the time peiod of the resulting simple harmonic motion of the object.
View SolutionA wooden block of mass 0.5 kg and density 800 kgm−3 is fastened to the free end of a vetical spring of spring cosntant 50 Nm−1 fixed at the bottom. If the entire system is completely immersed in water, find a. the elongation (or compresion) of the spring in equilibrium and b. the time period of vertical oscillations of het block when it is slightly depressed and released.
View SolutionAn object suspended from a spring exhibits oscillations of period T. Now ,the spring is cut in half and the two halves are used to support the same object, as shown in figure. Show that the new period of oscillation is T/2.
 .
.
View SolutionAn object suspended from a spring exhibits oscillations of period T. Now the spring is cut in two halves and the same object is suspsended with two halves as shown in figure. The new time period of oscillation will become

View SolutionAn object suspended from a spring exhibits oscillations of period T. Now the spring is cut in two halves and the same object is suspended with two halves as shown in figure. The new time period of oscillation will become
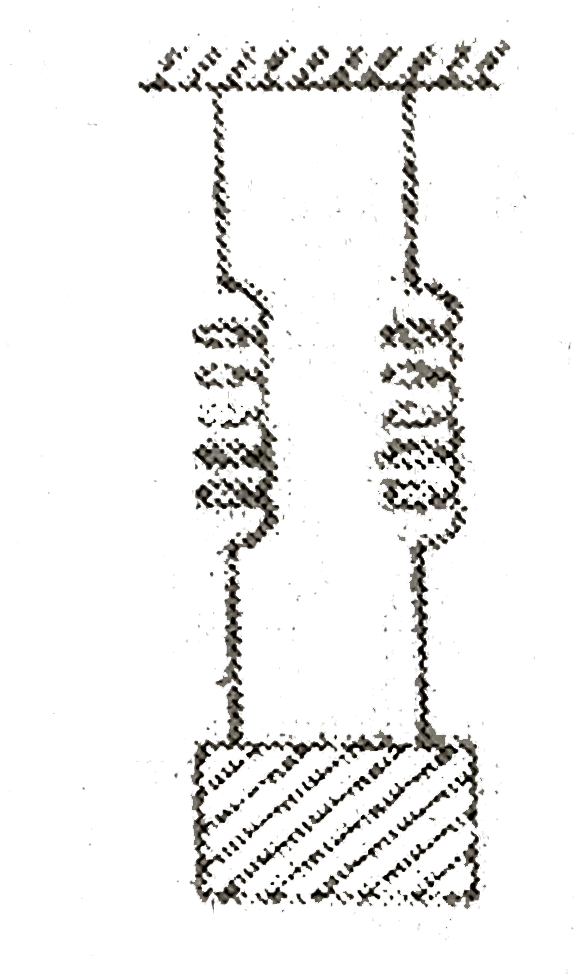
View Solution(i) 0.25 किग्रा द्रव्यमान की एक वस्तु जब किसी स्प्रिंग से लटकायी जाती है तो स्प्रिंग की लम्बाई सेमी बढ़ जाती है । इस स्प्रिंग का बल नियतांक एवं उसमे संचित ऊर्जा ज्ञात कीजिये ।
View Solutionएक वस्तु एक ऊर्ध्वाधर स्प्रिंग से लटकी हुई है और प्रतिसेकण्ड 10 दोलनम करती है दोलन के उच्चतम बिंदु पर स्प्रिंग की लम्बाई अपनी स्वाभाविक लम्बाई के बराबर है (a) वस्तु की अधिकतम चाल ज्ञात कीजिये (b) जब स्प्रिंग 0.20 cm खींचा हुआ है उस समय वस्तु की चाल ज्ञात कीजिये (g=π2m/s2 ले)
View Solutionचित्र 24.E6 में एक बेलनाकार वस्तु को स्प्रिंग से लटकता हुआ दिखाया गया है | वस्तु का कुछ भाग पानी में तथा कुछ भाग हवा में है | इस वस्तु का व्यास 10 cm, ऊँचाई 20 cm तथा घनत्व 8000kg/m3 है | स्प्रिंग का स्प्रिंग नियतांक 500N/m है | वस्तु का अक्ष सदा ऊर्ध्वाधर दिशा में रहता है |
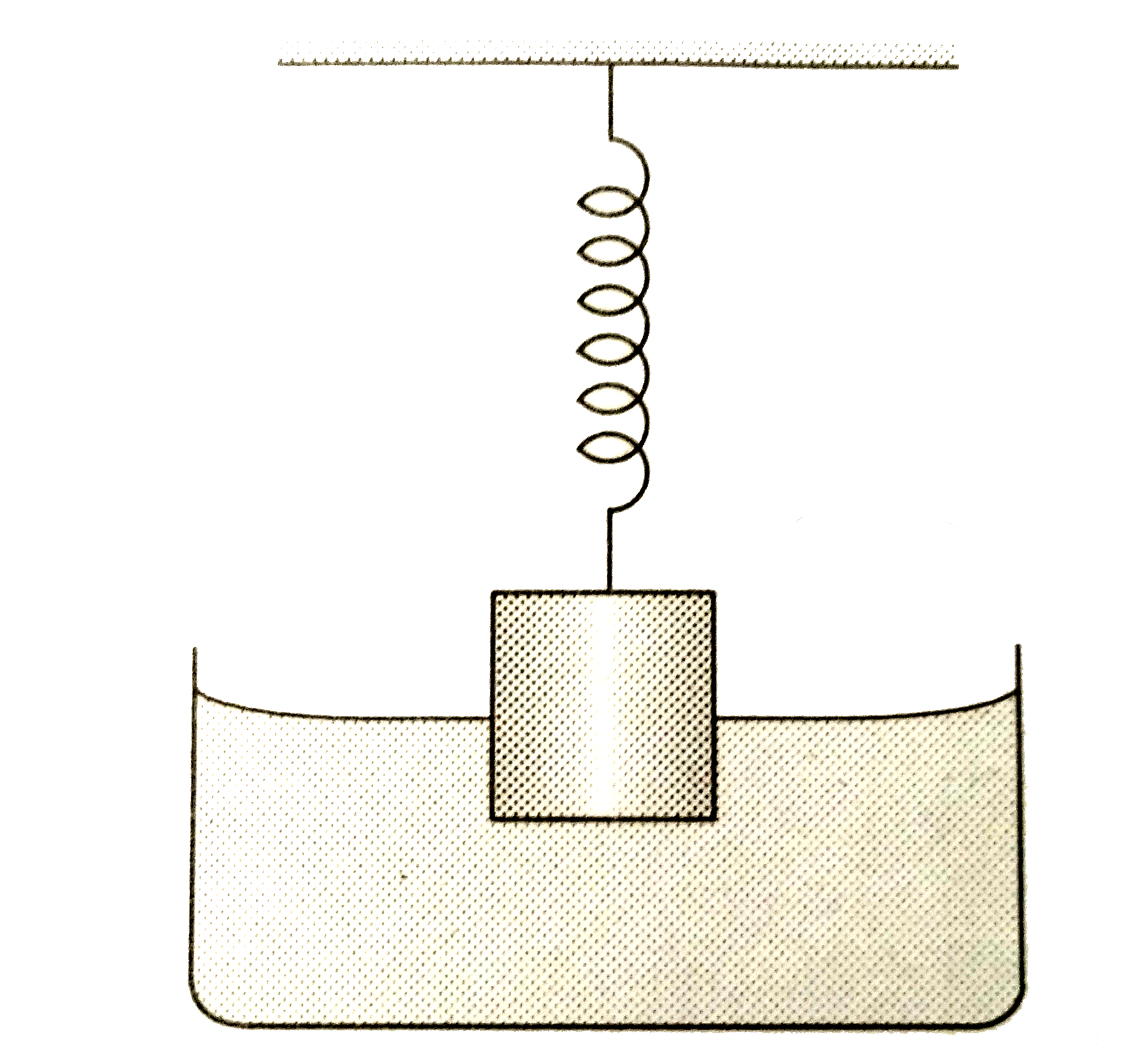
(a) संतुलन की स्थिति में स्प्रिंग की लंबाई में वृद्धि निकालें |
(b) यदि इस वस्तु को निचे की ओर थोड़ा दबाकर छोड़ दिया जाए, तो उसकी गति की आवृति निकालें |
View SolutionThe vertical extension in a light spring by a weight of 1 kg, in equilibrium is 9.8 cm. The period of oscillation of the spring, in seconds, will be…………….
View SolutionA 5kg collar is attached to a spring of spring constant 500 Nm^(-1) . It slides without friction over a horizontal rod. The collar is displaced from its equilibrium position by 10.0 cm and released. Calculate the period of oscillation.
View SolutionAn object of 'm' mass is suspended from a vertical spring of length L and oscillating for a period of time T. Tell periodic if (a) If the length of the spring is reduced to half. (b) The mass of the object should be reduced in half.
View SolutionA particle of mass m is attached with three springs A,B and C of equal force constancts k as shown in figure. The particle is pushed slightly against the spring C and released. Find the time period of oscillation.
 .
.
View SolutionA cylindrical object of outer diameter 20 cm and mass 2 kg floats in water with its axis vertical. If it is slightly depressed and then released, find the time period of the resulting simple harmonic motion of the object.
View SolutionA wooden block of mass 0.5 kg and density 800 kgm−3 is fastened to the free end of a vertical spring of spring constant 50 Nm−1 fixed at the bottom. If the entire system is completely immersed in water, find a. the elongation (or compression) of the spring in equilibrium and b. the time period of vertical oscillations of the block when it is slightly depressed and released.
View Solution
