In a rotor, a hollow vertical cylindrical structure rotates about its axis and a person rests against the inner wall. At a particular speed of the rotor, the floor below the person is removed and the person hangs resting against the wall without any floor. If the radius of the rotor is 2m and the coefficient of static friction between the wall and the person is 0.2, find the minimum speed at which the floor may be removed Take g=10ms2.Solution in Tamil
The situation is shown in ure.
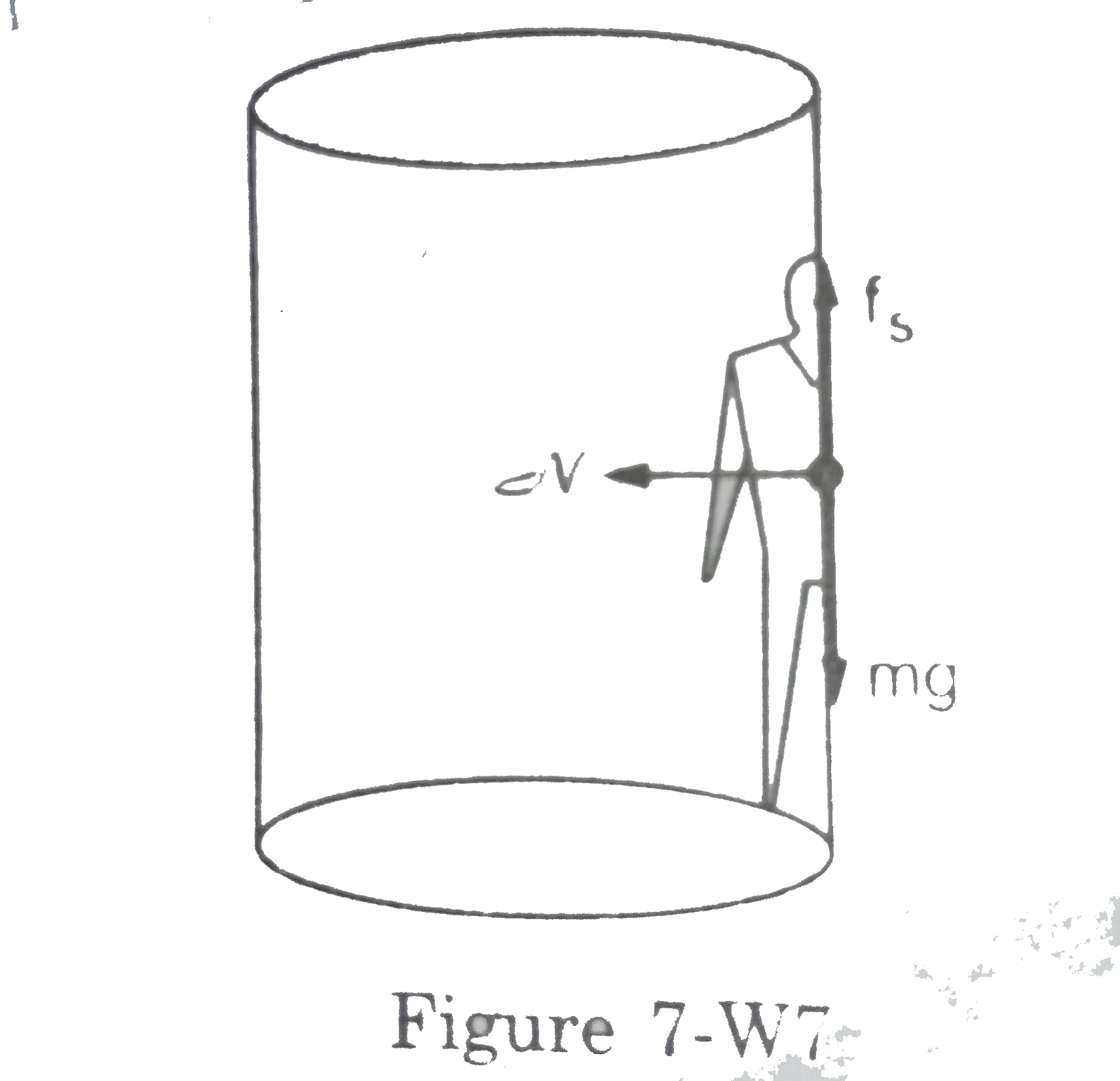
When the floor removed, the forces on the person are
a. weight mg downward
b. normal force N due to the wall, towards the centre
c. Frictional force fs parallel to the wall, upward.
The person is moving in a circle with as uniform speed, so its acceleration is v2r towards the centre.
Newton's law for the horizontal direction (2nd law) and for the vertical direction (1st law) give
N=mv2r........i
and fs=mg ...........ii
for the minimum speed when the floor may be removed, the friction is limiting one and so equals μsN.This gives
μsN=mg
or, μsmv2r=mg[using i]
or v=√rgμs=√2m×10ms20.2=10ms
Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - HC VERMACHAPTER - CIRCULAR MOTIONEXERCISE - Question for short Answer
10 Videos
- CIRCULAR MOTIONBOOK - HC VERMACHAPTER - CIRCULAR MOTIONEXERCISE - Objective -1
16 Videos
- CIRCULAR MOTIONBOOK - HC VERMACHAPTER - CIRCULAR MOTIONEXERCISE - Exercises
30 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMACHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Exercises
64 Videos
- FLUID MECHANICSBOOK - HC VERMACHAPTER - FLUID MECHANICSEXERCISE - All Questions
96 Videos
Similar Questions
You are driving a motorcycle ona horizontal raod. It is moving with a uniform velocity. Is it possibel to accelerate the motorcycle wilthout putting higher petrol input rate into the engine?
View SolutionWhen a particle moves in a circle with a uniform speed
View SolutionFind the accelerationof the moon with respect to the eath from the following data, Distance between the earth and the moon =3.85×105 km and the time taken by the moon to complete one revolution around the earth =27.3days
View SolutionThree particles of masses 1.0 kg 2.0 kg and 3.0 kg are placed at the corners A,B and C respectively of an equilateral triangle ABC of edge 1m. Locate the centre of mass of the system.
View SolutionWater is filled in a flask up to a height of 20 cm. The bottom of the flask is circular with radius 10 cm. If the atmospheric pressure is 1.01×105 Pa, find the force exerted by the water on the bottom. Take g=10ms−2 and density of water =1000 kgm^-3.
View SolutionA block is rotating in contact with vertical wall (rotor) as shown. Find the minimum value of ω so that the block does not slide down.

View Solution70 kg संहति का कोई व्यक्ति अपने ऊर्ध्वाधर अक्ष पर 200 rev/min कि चाल से घूर्णन करती 3m त्रिज्या कि किसी बेलनकार दीवार के साथ उसके संपर्क में खड़ा है। दीवार तथा उसके कपड़ो के बीच घर्षण गुणांक 0.15 है। दीवार कि वह न्यूनतम घूर्णन चाल ज्ञात कीजिए, जिससे फर्श को यकायक हटा लेने पर भी, वह व्यक्ति बिना गिरे दीवार से चिपके रह सके।
View Solutionरोटर नामक यंत्र में खोखली बेलनाकार संचरना अपने अक्ष में परित: घूमती है और एक मनुष्य उसकी भीतरी दीवार के सहारे संचरना के फर्श पर खड़ा होगा है। संचरना का कोणीय वेग धीरे-धीरे बढ़ाया जाता है और जब यह वेग एक खास मान तक पहुँच जाता है तो नीचे से फर्श हटा लिया जाता है। ऐसे में मनुष्य दीवार के सहारे, पर बिना किसी फर्श के टिका रहता है। यदि रोटर की त्रिज्या 2 m तथा मनुष्य और दीवार के बीच घर्षण गुणांक 0.2 हो तो रोटर का न्यूनतम कोणीय वेग ज्ञात करे जब फर्श हटाया जा सके। g का मान 10m/s2 है।
View SolutionA person stands in contact against a wall of cylindrical drum of radius 'r' rotating with an angular velocity omega . If mu is coefficient ofn static fiction between the wall and the person , then the minimum rotational speed which enables the person to remain struck to the wall will be
View SolutionA person stands in contact against the inner surface of a cylindrical drum of radius (R ) rotating with angular velocity ω . The coefficient of friction between the inner surface of the minumum rotational speed of the cylinder , which enables the person to ramain stuck to the wall , when the platform on which the person was standing is suddenly removed ?
View SolutionA person stands in contact against the inner wall of a rotor of radius r. The coefficient of friction between the wall and the clothing is mu and the rotor is rotating about vertical axis. The minimum angular speed of the rotor so that the person does not slip downward is
View SolutionA uniform ladder is standing against a vertical smooth wall, resting on a horizontal floor. The ladder makes an angle of 45^(@) with the floor when its lower end is about to slip. What is the value of the coefficient of friction between the ladder and the floor?
View SolutionA 70kg man stands in contact against the inner wall of a hollow cylindrical drum of radius 3m rotating about its vertical axis. The coefficient of friction between the wall and his clothing is 0.15 . What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed?
View SolutionA person stands in contact against a wall of cylindrical drum of radius r, rotating with an angular velocity ω. If μ is the coefficient of friction between the wall and the person, the minimum rotational speed which enables the person to remain stuck to the wall is
View SolutionA person is in contact with inner wall of a vertical hollow cylinder of radius 2m without floor under his feet, remains in equilibrium without slipping down when the cylinder is rotated about it's own vertical axis. If the coefficient of static friction between person and wall of cylinder is 0.4, the minimum angular velocity of cylinder should be
View SolutionA rotor of radius (r) is rotating about its own vertical axis and a person in contact with innerwall of rotor remains in equilibrium without slipping down. If 'w' is angular velocity of rotor and μ is minimum coefficient of friction between persona and the wall of rotor. Then following is correct
(A) μαw2 (B) μα1r (C) μα1w2 (D) μαr
View Solution
