A pilot in an aeroplane at an altitude of 200 metre observes two points lying on either side of a river .If the angles of depression of the two points be 45∘and60∘, then the width of the river is
(200+200√3)metre
(200−200√3)metre
400√3 metre
(400√3)metre
The correct Answer is:A
To solve the problem, we need to determine the width of the river based on the angles of depression observed by the pilot at an altitude of 200 meters.
1. Understanding the Angles of Depression:
- The angles of depression from the pilot to points P and Q on either side of the river are given as 45° and 60°, respectively.
2. Drawing the Diagram:
- Let's denote the altitude of the airplane as point O, which is 200 meters above the ground.
- Let point P be the point on one side of the river and point Q be the point on the other side.
- The angles of depression from point O to points P and Q create two right triangles: triangle OAP (for angle 45°) and triangle OBQ (for angle 60°).
3. Using Trigonometry for Triangle OAP:
- In triangle OAP, where the angle of depression is 45°, we know:
tan(45°)=oppositeadjacent=200d1
where d1 is the horizontal distance from point O to point P.
- Since tan(45°)=1:
1=200d1⟹d1=200 meters
4. Using Trigonometry for Triangle OBQ:
- In triangle OBQ, where the angle of depression is 60°, we have:
tan(60°)=oppositeadjacent=200d2
where d2 is the horizontal distance from point O to point Q.
- Since tan(60°)=√3:
√3=200d2⟹d2=200√3 meters
5. Calculating the Width of the River:
- The total width of the river (W) is the sum of distances d1 and d2:
W=d1+d2=200+200√3
6. Simplifying the Expression:
- To express the width in a single fraction:
W=200+200√3=200(1+1√3)
- To combine the terms:
W=200(√3√3+1√3)=200(√3+1√3)
Final Answer:
The width of the river is:
W=200(√3+1)√3 meters
- The angles of depression from the pilot to points P and Q on either side of the river are given as 45° and 60°, respectively.
- Let's denote the altitude of the airplane as point O, which is 200 meters above the ground.
- Let point P be the point on one side of the river and point Q be the point on the other side.
- The angles of depression from point O to points P and Q create two right triangles: triangle OAP (for angle 45°) and triangle OBQ (for angle 60°).
- In triangle OAP, where the angle of depression is 45°, we know:
tan(45°)=oppositeadjacent=200d1
where d1 is the horizontal distance from point O to point P.
- Since tan(45°)=1:
1=200d1⟹d1=200 meters
- In triangle OBQ, where the angle of depression is 60°, we have:
tan(60°)=oppositeadjacent=200d2
where d2 is the horizontal distance from point O to point Q.
- Since tan(60°)=√3:
√3=200d2⟹d2=200√3 meters
- The total width of the river (W) is the sum of distances d1 and d2:
W=d1+d2=200+200√3
- To express the width in a single fraction:
W=200+200√3=200(1+1√3)
- To combine the terms:
W=200(√3√3+1√3)=200(√3+1√3)
The width of the river is:
W=200(√3+1)√3 meters
Topper's Solved these Questions
- TRIGONOMETRY Book:KIRAN PUBLICATIONChapter:TRIGONOMETRY Exercise:TYPE -V
Explore 45 Videos
- TRIGONOMETRY Book:KIRAN PUBLICATIONChapter:TRIGONOMETRY Exercise:TEST YOURSELF
Explore 25 Videos
- TRIGONOMETRY Book:KIRAN PUBLICATIONChapter:TRIGONOMETRY Exercise:TYPE -III
Explore 90 Videos
- TIME AND WORKBook:KIRAN PUBLICATIONChapter:TIME AND WORKExercise:TEST YOURSELF
Explore 25 Videos
Similar Questions
An aeroplane at an altitude of 200 metres observes the angles of depression of opposite points on the two banks of a river to be 450and600. Find the width of the river.
View SolutionThe pilot of an aeroplane at an altitutde of 200 m observes the angle of depression of opposite points on the two banks of a river to be 45∘ and 60∘. Find the width of the river.
View Solution
Knowledge Check
From the top of a building 60 metre high ,the angles of depression of the top and bottom of a tower are observed to be 30∘and60∘ respectively.The height of the tower in metre is :
Aa.40Bb.45Cc.50Dd.55If from point 100 m above the ground the angles of depression of two objects due south on the ground are 60∘and45∘, then the distance between the objects is :
A50(3−√3)3mB50(3+√3)3mC100(3+√3)3mD100(3−√3)3m
An aeroplane at an altitude of 200 m observes the angles od depression of opposite points on the two banks of a river to be 45∘and60∘. Find the width of the river.
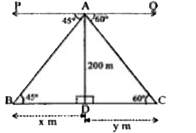
View SolutionAn aeroplane at an altitude of 200 m observes angles of depression of opposite points on the two banks of the river to be 45∘ and 60∘, find the width of the river.
View SolutionFrom a point on a bridge across a river, the angles of depression of the banks on opposite sides of the river are 30oand 45o, respectively. If the bridge is at a height of 3 m from the banks, find the width of the river.
View SolutionAn aeroplane is flying over two houses which are at a distance of 300 m from each other. If at some moment from the aeroplane the angle of depression of two houses on the same side are 45∘ and 60∘, at what height the plane is flying ?
View SolutionAn aeroplane at an altitude of 1200 metres finds that two ships are sailing towards it in the same direction. The angles of depression of the ships as observed from the aeroplane are 60o and 30o respectively. Find the distance between the two ships.
View SolutionThe two palm trees are of equal heights and are standing opposite each other on either side of the river, which is 80 m wide. From a point O between them on the river the angles of elevation of the top of the trees are 60∘ and 30∘, respectively. Find the height of the trees and the distances of the point O from the trees.
OR
The angles of depression of the top and bottom of a building 50 meters high as observed from the top of a tower are 30∘ and 60∘ respectively. Find the height of the tower, and also the horizontal distance between the building and the tower.
View Solution