Question

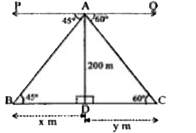
An aeroplane at an altitude of 200 m observes angles of depression of opposite points on the two banks of the river to be ${45}^{\circ }$ and ${60}^{\circ }$, find the width of the river.

Answer

315.8 m

Explanation

To find the width of the river based on the angles of depression observed from an airplane, we can follow these steps:

Step 1: Understand the Problem
We have an airplane flying at an altitude of 200 meters. It observes two points on opposite banks of a river with angles of depression of 45° and 60°.

Step 2: Draw a Diagram
Draw a vertical line representing the altitude of the airplane (200 m). Mark the point where the airplane is located as point A. Draw horizontal lines from point A to the points on the banks of the river, marking the angles of depression. Let the points on the banks be B (for 45°) and C (for 60°).

Step 3: Identify the Right Triangles
From point A, draw perpendicular lines down to the riverbank at points B and C. This creates two right triangles:
- Triangle ADB (where D is the point directly below A on the riverbank)
- Triangle AEC (where E is the point directly below A on the riverbank)

Step 4: Use Tangent to Find Distances
For triangle ADB:
- The angle of depression is 45°, so the angle at point D is also 45° (alternate angles).
- Using the tangent function:
\( \tan(45°) = \frac{\text{opposite}}{\text{adjacent}} = \frac{200}{x} \)
Since \(\tan(45°) = 1\), we have:
\( 1 = \frac{200}{x} \implies x = 200 \text{ m} \)

For triangle AEC:
- The angle of depression is 60°, so the angle at point E is also 60°.
- Using the tangent function:
\( \tan(60°) = \frac{\text{opposite}}{\text{adjacent}} = \frac{200}{y} \)
Since \(\tan(60°) = \sqrt{3}\), we have:
\( \sqrt{3} = \frac{200}{y} \implies y = \frac{200}{\sqrt{3}} \text{ m} \)

Step 5: Calculate the Width of the River
The width of the river is the sum of distances x and y:
\( \text{Width of the river} = x + y = 200 + \frac{200}{\sqrt{3}} \)

Step 6: Simplify the Expression
To simplify:
\( \text{Width of the river} = 200 \left(1 + \frac{1}{\sqrt{3}}\right) \)
We can approximate \(\sqrt{3} \approx 1.73\):
\( \frac{1}{\sqrt{3}} \approx \frac{1}{1.73} \approx 0.577 \)
Thus,
\( \text{Width of the river} \approx 200 \left(1 + 0.577\right) = 200 \times 1.577 \approx 315.4 \text{ m} \)

Final Answer
The width of the river is approximately 315.4 meters.

---