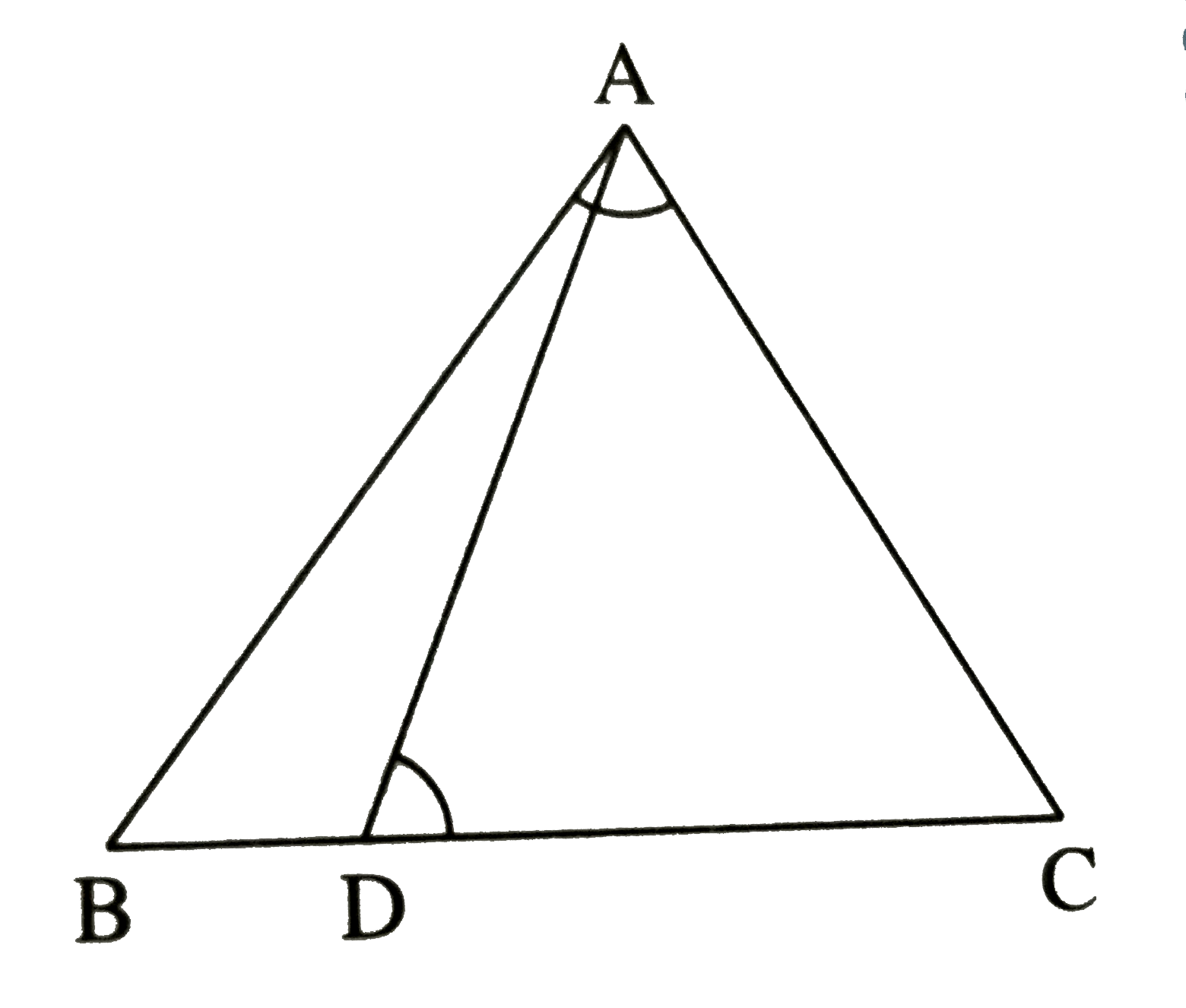
In the given figure , D is a point on the side BC of Δ A B C such that ∠ A D C = ∠ B A C . Prove that C A 2 = C B × C D .
In the given figure , D is a point on the side BC of Delta ABC such that angle ADC=angle BAC. Prove that CA^(2)=CBxxCD.

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is: ⇒ C A 2 = C B × C D

Updated on:21/07/2023
|
Answer

Step by step video, text & image solution for In the given figure , D is a point on the side BC of Delta ABC such that angle ADC=angle BAC. Prove that CA^(2)=CBxxCD. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.

Updated on:21/07/2023
Class 10MATHSTRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • D is a point on the side BC of a ΔABC such that AD bisects ∠BAC . Then

    ABD=CD
    BBA>BD
    CBD>BA
    DCD>CA

  • D is a point on the side BC of △ABC such that ∠ADC=∠BAC. If CA = 12 cm and BC = 16 cm, then the length of CD is :

    A12 cm
    B6 cm
    C8 cm
    D9 cm

  • In ΔABC, D is a point on side BC such that ∠ADC=∠BAC, If CA = 12 cm, CB = 8 cm then CD is equal to :

    A18 cm
    B12 cm
    C16 cm
    D15 cm