A chord that passes through the centre of a circle is___
Diameter
Segment
Radius
Centre
The correct Answer is:a
Step by step video, text & image solution for A chord that passes through the centre of a circle is___ by Maths experts to help you in doubts & scoring excellent marks in Class 6 exams.
Updated on:21/07/2023Topper's Solved these Questions
- BASIC GEOMETRICAL IDEASBOOK - MTG IIT JEE FOUNDATIONCHAPTER - BASIC GEOMETRICAL IDEASEXERCISE - EXERCISE(MCQ)LEVEL-2
10 Videos
- BASIC GEOMETRICAL IDEASBOOK - MTG IIT JEE FOUNDATIONCHAPTER - BASIC GEOMETRICAL IDEASEXERCISE - MATCH THE FOLLOWING
2 Videos
- BASIC GEOMETRICAL IDEASBOOK - MTG IIT JEE FOUNDATIONCHAPTER - BASIC GEOMETRICAL IDEASEXERCISE - NCERT SECTION(EXERCISE 4.6)
20 Videos
- ALGEBRABOOK - MTG IIT JEE FOUNDATIONCHAPTER - ALGEBRAEXERCISE - OLYMPIAD/HOTS CORNER
20 Videos
- DATA HANDLING BOOK - MTG IIT JEE FOUNDATIONCHAPTER - DATA HANDLING EXERCISE - Olympiad/HOTS Corner
10 Videos
Similar Questions
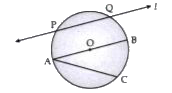
The chord, which passes through the centre of the circle, is called a ........... of the circle.
View SolutionDIAMETER A chord passing through the centre of a circle is known as its diameter. Note that a circle has many diameters and a diameter of a given circle is one of the largest chords of the circle. Also all diameters are of the same length.
View Solution
Knowledge Check
In the given figure, TB is a chord which passes through the centre of the circle . PT is a tangent to the circle at the point T on the circle . If PT=10cm, PA=5cm and AB=xcm, then the radius of the circle is :
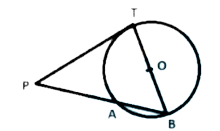 A5√3cmB6√5cmC3√5cmD10√3cm
A5√3cmB6√5cmC3√5cmD10√3cmTwo circles of radii 7cm and 5 cm intersect each other at A and B, the distance between their centres is 10 cm. The length (in cm) of the common chord AB is:
दो वृत्त, जिनकी त्रिज्याएँ 7 सेमी तथा 5 सेमी हैं, एक-दूसरे को A और B पर प्रतिच्छेद करते हैं | उनके केंद्रों के बीच की दूरी 10 सेमी है | उभयनिष्ठ जीवा AB की लंबाई ( सेमी में ) कितनी है ?A√2r unitsB√3r unitsC√5r unitsDrunitsTwo equal circles of radius 4 cm intersect each other such that each passes through the centre of the other. The length of the common chord is :
A2√3 cmB4√3 cmC2√2 cmD8 cm
In the given figure, TB is a chord which passes through the centre of the circle. PT is a tangent to the circle at the point T on the circle. If PT = 10 cm, PA = 5 cm and AB = x cm. then the radius of the circle is:
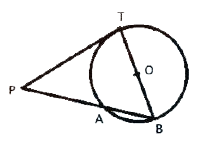
View SolutionIf a line segment joining mid-points of two chords of a circle passes through the centre of the circle, prove that the two chords are parallel.
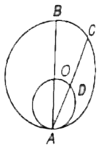
View SolutionA smaller circle touches internally to a larger circle at A and passes through the centre of the larger circle. O is the centre of the larger circle and BA, OA are of the diameters of the larger and smaller circles respectively. Chord AC intersects the smaller circle at a point D. If AC=12cm, then AD is :
View SolutionTwo equal circles intersect such that each passes through the centre of the other. If the length of the common chord of the circles is 10√3 cm, then what is the diameter of the circle?
View SolutionIn the given figure, a straight line l passing through the centre O of the circle bisects the chord AB and CD. Prove that AB||CD.
View Solution
