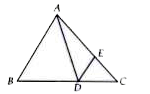
Assertion : In the given fig ure, the point D divides the side BC of $△ A B C$ in the ratio $m : n$ , then ratio of $a r (A B D)$ and $a r (A D C)$ is $m : n$
$Assertion : In the given fig ure, the point D divides the side BC of /\ABC in the ratio m : n, then ratio of ar(ABD) and ar(ADC) is m:n Reason : Area of triangle = 1/2 xx Base xx Height$
Reason : Area of triangle = 1/2 $\times$ Base $\times$ Height

If both assertion and reason are tme and reason is the correct explanation of assertion.

If both assertion and reason are true but reason is not the correct explanation of assertion.

If assertion is true but reason is false.

If assertion is false but reason is trne.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Answer

Step by step video, text & image solution for Assertion : In the given fig ure, the point D divides the side BC of /_\ABC in the ratio m : n, then ratio of ar(ABD) and ar(ADC) is m:n Reason : Area of triangle = 1/2 xx Base xx Height by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.

Updated on:21/07/2023

Class 9 MATHS AREA OF PARALLELOGRAMS AND TRIANGLES

Topper's Solved these Questions

Knowledge Check

Question 1 - Select One
ABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of $Δ A B C and Δ D E F$ is :
A $4 : 1$
B $5 : 1$
C $3 : 1$
Dcan't be determined
Question 2 - Select One
ABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of $Δ A B C and Δ D E F$ is :
A4:1
B5:1
C3:1
Dcan't be determind
Question 3 - Select One
In $△ A B C,$ D divides BC in the ratio l:m, G divides AD in the ratio (l+m):n then the position vector of G is
A $\frac{m ¯ c + n ¯ b + l ¯ a}{m + n + l}$
B $\frac{l ¯ a + m ¯ b + n ¯ c}{m + n + l}$
C $\frac{n ¯ c + l ¯ b + m ¯ a}{m + n + l}$
D $\frac{l ¯ c + m ¯ b + n ¯ a}{m + n + l}$

MTG IIT JEE FOUNDATION-AREAS OF PARALLELOGRAMS AND TRIANGLES-EXERCISE (Assertion & Reason Type )

Home
Ask Doubt
Profile

Assertion : In the given fig ure, the point D divides the side BC of △ABC in the ratio m:n, then ratio of ar(ABD) and ar(ADC) is m:n Reason : Area of triangle = 1/2 × Base × Height

The correct Answer is:A

Topper's Solved these Questions

Similar Questions

Knowledge Check

ABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :

ABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of ΔABCandΔDEF is :

In △ABC, D divides BC in the ratio l:m, G divides AD in the ratio (l+m):n then the position vector of G is

MTG IIT JEE FOUNDATION-AREAS OF PARALLELOGRAMS AND TRIANGLES-EXERCISE (Assertion & Reason Type )

ABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of $Δ A B C and Δ D E F$ is :

ABC is a triangle and D,E,F are the mid-points of the sides BC,CA, AB respectively . The ratio of the areas of $Δ A B C and Δ D E F$ is :

In $△ A B C,$ D divides BC in the ratio l:m, G divides AD in the ratio (l+m):n then the position vector of G is