The angle in a semi-circle is
a) a reflex angle
b) an obtuse angle
c) an acute angle
d) a right angle
The correct Answer is:D
To find the angle in a semicircle, we can follow these steps:
1. Draw a Circle: Start by drawing a circle with a diameter. Label the endpoints of the diameter as points A and B.
2. Identify the Center: Mark the center of the circle as point O, which is the midpoint of the diameter AB.
3. Draw a Triangle: Choose any point C on the circumference of the circle. This point will help us form triangle ABC, where AB is the diameter.
4. Understand the Angles: According to the properties of circles, the angle subtended by the diameter at any point on the circle (in this case, angle ACB) is always a right angle (90 degrees).
5. Apply the Angle Property: We know that the angle subtended at the center (angle AOB) is twice the angle subtended at the circumference (angle ACB). Since AB is a straight line, angle AOB is 180 degrees.
6. Calculate the Angle: Using the property mentioned, we can express the relationship as:
Angle ACB=Angle AOB2=180∘2=90∘
7. Conclusion: Therefore, the angle in a semicircle (angle ACB) is 90 degrees.
Final Answer:
The angle in a semicircle is 90 degrees (a right angle).
---
Angle ACB=Angle AOB2=180∘2=90∘
The angle in a semicircle is 90 degrees (a right angle).
Topper's Solved these Questions
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-V)
Explore 30 Videos
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-VI)
Explore 8 Videos
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-III)
Explore 109 Videos
- DISCOUNTBook:KIRAN PUBLICATIONChapter:DISCOUNTExercise:Test Yourself
Explore 10 Videos
- LCM AND HCF Book:KIRAN PUBLICATIONChapter:LCM AND HCF Exercise:Test Yourself
Explore 18 Videos
Similar Questions
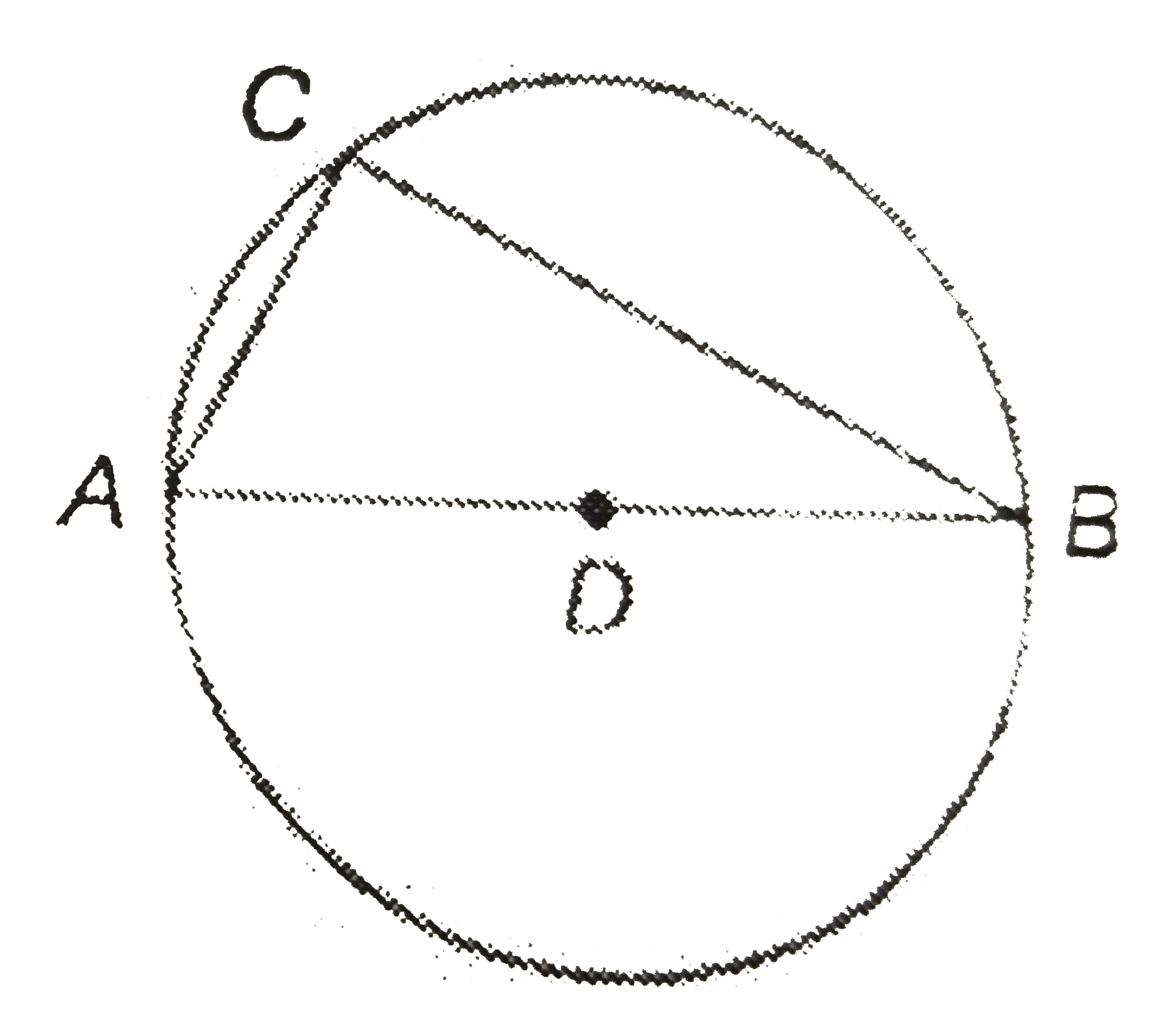
Show that any angle in a semi-circle is a right angle.
The following arc the steps involved in showing the above result. Arrange them in sequential order.
A) ∴∠ACB=180∘2=90∘
B) The angle subtended by an arc at the center is double of the angle subtended by the same arc at any point on the remaining part of the circle.
c) Let AB be a diameter of a circle with center D and C be any point on the circle. Join AC and BC.
D) ∴∠AD=2×∠ACB
180∘=2∠ACB(∴∠ADB=180∘)
View SolutionUsing vector method, prove that the angel in a semi circle is a right angle.
View Solution
Knowledge Check
If the area of a semi-circle is 1925cm2, then find its radius (in cm).
A70B31C62D35If the area of a semi-circle is 693cm2, then find its radius (in cm).
A42B27C21D54If the area of a semi-circle is 308 cm2, then find its radius (in cm).
A28B10C20D14
Angle subtend by an arc at center is double the angle subtend b it at any point on remaining part OF circle||Angle is a semi circle is a 90 ||Example ||Congurent arc|| Equal arc||Cyclic quadrilateral
View SolutionProve that an angle inscribed in a semi-circle is a right angle using vector method.
View SolutionProve that the angle in a segment greater than a semi-circle is less than a right angle.
View SolutionSemi-Circles
View SolutionIf the area of a semi-circle is 308 cm2, then find its radius (in cm).
View Solution