Show that any angle in a semi-circle is a right angle.
The following arc the steps involved in showing the above result. Arrange them in sequential order.
A) ∴∠ACB=180∘2=90∘
B) The angle subtended by an arc at the center is double of the angle subtended by the same arc at any point on the remaining part of the circle.
c) Let AB be a diameter of a circle with center D and C be any point on the circle. Join AC and BC.
D) ∴∠AD=2×∠ACB
180∘=2∠ACB(∴∠ADB=180∘)
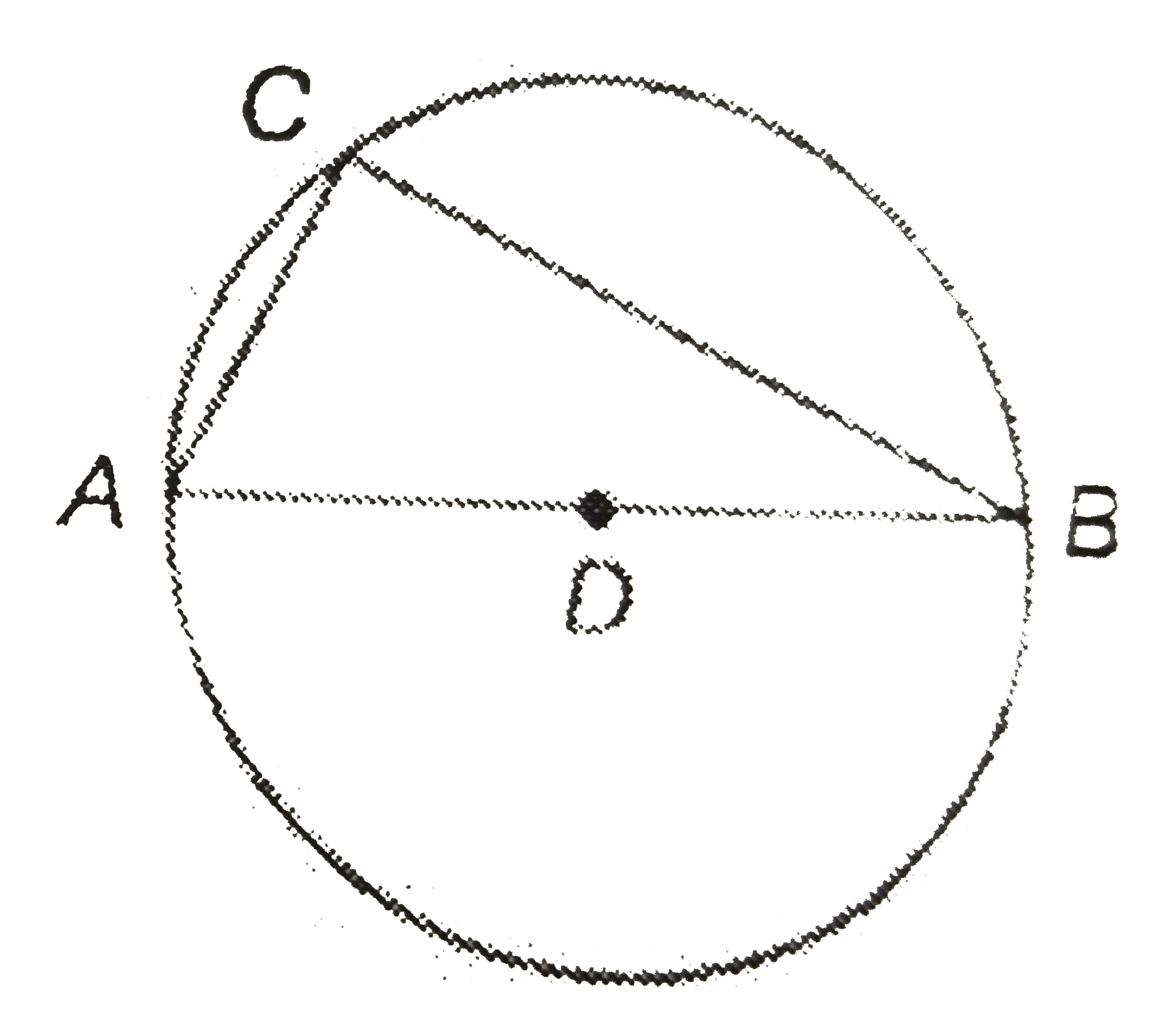
DBAC
DBCA
CBAD
CBDA
The correct Answer is:D
CBDA is the required sequential order.
Topper's Solved these Questions
- GEOMETRYBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - GEOMETRYEXERCISE - SHORT ANSWER TYPE QUESTIONS
18 Videos
- COORDINATE GEOMETRYBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - COORDINATE GEOMETRYEXERCISE - Level 3
9 Videos
- LINEAR EQUATIONS AND INEQUATIONSBOOK - PEARSON IIT JEE FOUNDATIONCHAPTER - LINEAR EQUATIONS AND INEQUATIONSEXERCISE - level 3
10 Videos
Similar Questions
Theorem 10.8 : The angle subtended by an arc at the centre is double the angle subtended by it at any point on the remaining part of the circle.
View SolutionThe angle subtended by an arc of a circle at the centre is double the angle subtended by it any point on the remaining part of the circle.
View Solution
Knowledge Check
The angle subtended by a minor arc in its alternate segment is…………….
AacuteBobtuseC90∘Dreflex angle.Calculate the angle subtended by the chord at the point on the major arc if the radius and the chord of the circle are equal in length
AA) 30∘BB) 120∘CC) 200∘DD) 300∘A chord of a circle is equal to its radius. The angle subtended by this chord at a point on the circumference is
A75∘B60∘C150∘D120∘
Angle subtend by an arc at center is double the angle subtend b it at any point on remaining part OF circle||Angle is a semi circle is a 90 ||Example ||Congurent arc|| Equal arc||Cyclic quadrilateral
View SolutionTheorem:-5 (i) The degree measure of an arc or angle subtended by an arc at the centre is double the angle subtended by it at any point of alternate segment. (ii) Angle in the same segment of a circle are equal (iii) The angle in the semi circle is right angle.
View SolutionAny angle subtended by a minor arc in the alternate segment is acute and any angle subtended by a major arc in the alternate segment is obtuse.
View SolutionConsider the following statements
1. the opposite angles of a cyclic quadrilateral are supplementary.
2. Angle subtended by an arc at the centre is doubled the angle subtended by it any point on the remaining part of the circle.
Which one of the following is correct in respect of the above statements
View SolutionIf the chord of a circle is equal to the radius of the circle, then the angle subtended by the chord at a point on the minor arc is:
View Solution
