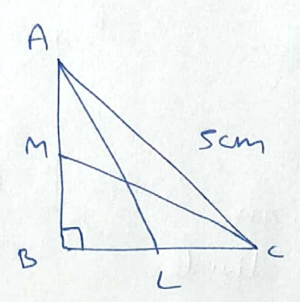
In a Δ right angled at B . AC = 5 cm , median AL=3√52 find the length of second median CM in cm ?
2√5 cm
5√2 cm
10√2 cm
4√5 cm
The correct Answer is:A
Step by step video, text & image solution for In a Delta right angled at B . AC = 5 cm , median AL = (3 sqrt(5))/(2) find the length of second median CM in cm ? by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-V)
Explore 30 Videos
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-VI)
Explore 8 Videos
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-III)
Explore 109 Videos
- DISCOUNTBook:KIRAN PUBLICATIONChapter:DISCOUNTExercise:Test Yourself
Explore 10 Videos
- LCM AND HCF Book:KIRAN PUBLICATIONChapter:LCM AND HCF Exercise:Test Yourself
Explore 18 Videos
Similar Questions
Knowledge Check
In a Δ right angled at B . AC = 5 cm , median AL=3√52 find the length of second median CM in cm ?
 A2√5 cmB5√2 cmC10√2 cmD4√5 cm
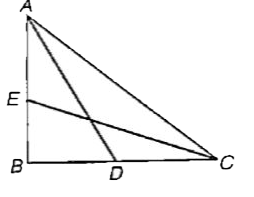
A2√5 cmB5√2 cmC10√2 cmD4√5 cmIn figure, ABC is a right triangle, right angled at B. AD and CE are the two medians drawn from A and C respectively. If AC = 5 cm and AD=3√52cm, find the length of CE:
 A2√5cmB2.5cmC5cmD4√2cm
A2√5cmB2.5cmC5cmD4√2cmΔABC is right-angled at C. If AC=5cmandBC=12cm find the length of AB.
A17B7C13D14
In Fig. 4.192, ABC is a right triangle right-angled at B . AD and CE are the two medians drawn from A and C respectively. If AC=5cm and AD=3√52cm , find the length of CE . (FIGURE)
View SolutionIn triangle ABC, angle B is right angled, AC=2 and A(2,2),B(1,3) then the length of the median AD is
View SolutionIn △ABC, AB = 6 cm, AC = 8 cm, and BC = 9 cm. The length of median AD is:
View SolutionIn the given figure,ABC in a right-angled triangle,right-angled atB.IfBC=5cmandAC-AB=1cm,f∈dthevalueofcosecAandcos A
View SolutionIn a △ABC,AB = 10 cm, BC = 12 cm and AC = 14 cm. Find the length of median AD. If G is the centroid, find length of GA.
View Solution