A thin uniform rod AB of mass m and length l is hinged at one end to the level floor and stands vertically. If it s allowed to fall, with what angular velocity will it strike the floor?
√mgl
√3gl
√5mgl
√2mgl
The correct Answer is:B
To find the angular velocity of a thin uniform rod of mass m and length l when it strikes the floor after being allowed to fall from a vertical position, we can use the principle of conservation of mechanical energy. Here's a step-by-step solution:
Step 1: Identify the Initial Potential Energy
When the rod is in the vertical position, the center of mass of the rod is at a height of l2 from the floor. The initial potential energy (PE_initial) of the rod can be calculated using the formula:
PEinitial=mgh
where h=l2. Thus,
PEinitial=mg(l2)=mgl2
Step 2: Identify the Final Potential Energy
When the rod falls and becomes horizontal, its center of mass is at the level of the floor, meaning its height is now 0. Therefore, the final potential energy (PE_final) is:
PEfinal=0
Step 3: Identify the Final Kinetic Energy
When the rod is horizontal, all the initial potential energy has been converted into rotational kinetic energy (KE_final). The rotational kinetic energy can be expressed as:
KEfinal=12Iω2
where I is the moment of inertia of the rod about the hinge point. For a thin rod hinged at one end, the moment of inertia is given by:
I=13ml2
Step 4: Apply Conservation of Energy
According to the conservation of mechanical energy, the initial potential energy equals the final kinetic energy:
PEinitial=KEfinal
Substituting the values we have:
mgl2=12(13ml2)ω2
Step 5: Simplify the Equation
We can simplify the equation:
mgl2=ml26ω2
Cancel m from both sides (assuming m≠0):
gl2=l26ω2
Step 6: Solve for Angular Velocity ω
Now, we can solve for ω2:
ω2=gl2⋅6l2=3gl
Taking the square root gives us:
ω=√3gl
Final Answer
Thus, the angular velocity ω when the rod strikes the floor is:
ω=√3gl
When the rod is in the vertical position, the center of mass of the rod is at a height of l2 from the floor. The initial potential energy (PE_initial) of the rod can be calculated using the formula:
PEinitial=mgh
where h=l2. Thus,
PEinitial=mg(l2)=mgl2
When the rod falls and becomes horizontal, its center of mass is at the level of the floor, meaning its height is now 0. Therefore, the final potential energy (PE_final) is:
PEfinal=0
When the rod is horizontal, all the initial potential energy has been converted into rotational kinetic energy (KE_final). The rotational kinetic energy can be expressed as:
KEfinal=12Iω2
where I is the moment of inertia of the rod about the hinge point. For a thin rod hinged at one end, the moment of inertia is given by:
I=13ml2
According to the conservation of mechanical energy, the initial potential energy equals the final kinetic energy:
PEinitial=KEfinal
Substituting the values we have:
mgl2=12(13ml2)ω2
We can simplify the equation:
mgl2=ml26ω2
Cancel m from both sides (assuming m≠0):
gl2=l26ω2
Now, we can solve for ω2:
ω2=gl2⋅6l2=3gl
Taking the square root gives us:
ω=√3gl
Thus, the angular velocity ω when the rod strikes the floor is:
ω=√3gl
Similar Questions
A thin rod of mass m and length l is hinged at the lower end to a level floor and stands vertically. Then its upper end will strike the floor with a velocity given by:
View SolutionA uniform rod of mass m and length / is hinged at upper end. Rod is free to rotate in vertical plane. A bail of mass m moving horizontally with velocity vo collides at lower end of rod perpendicular to it and sticks to it. The minimum velocity of the ball such that combined system just completes the vertical circle will be
View SolutionA uniform rod of mass m and length l is suspended about its end. Time period of small angular oscillations is

View SolutionA uniform rod of mass m and length L is hinged about one end and can freely rotate in a vertical plane. The angular velocity of the rod, when it falls from position P to Q. through an angle α starting from rest, is

View SolutionA uniform rod ofmass M and length L is hanging from its one end free to rotate in a veritcal plane.A small ball of equal mass is attached of the lowe end as shown. Time period of small oscillations of the rod is

View SolutionA slender uniform rod of mass M and length l is pivoted at one end so that it can rotate in a vertical plane, Fig. There is negligible friction at the pivot. The free end is held vertically above the pivot and then released. The angular acceleration of the rod when it makes an angle θ with the vertical is
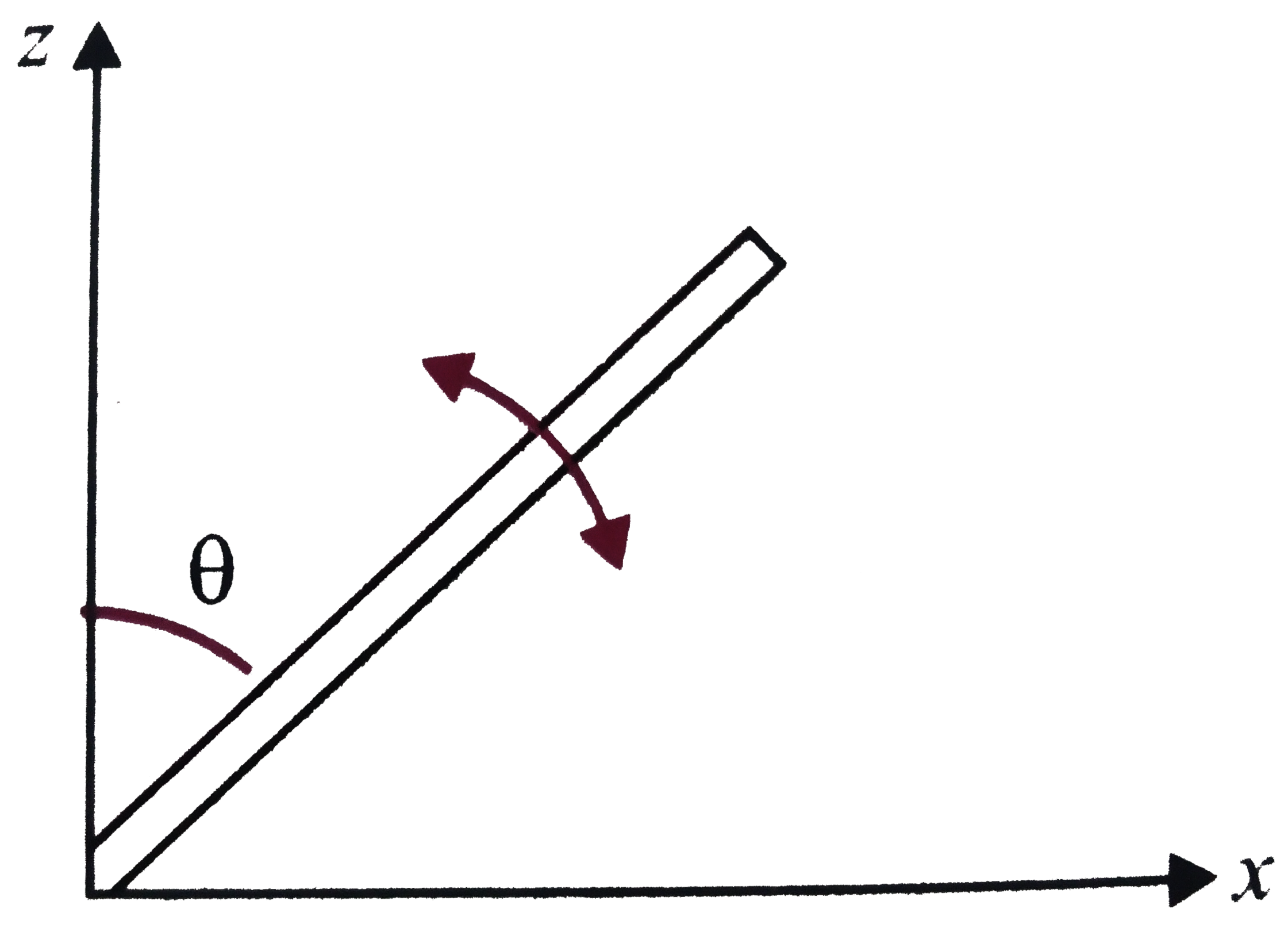
View SolutionA thin and uniform rod of mass M and length L is held vertical on a floor with large friction. The rod is released from rest so that it falls by rotating about its contact-point with the floor without slipping. Which of the following statement(s) is(are) correct, when the rod makes an angle 60° with vertical? [g is the acceleration due to gravity]
View SolutionA uniform rod of mass m and length l is pivoted smoothly at O. A horizontal force acts at the bottom of the rod.
a. Find the angular velocity of the rod as the function of angle of rotation θ.
b.What is the maximum angular displacement of the rod?
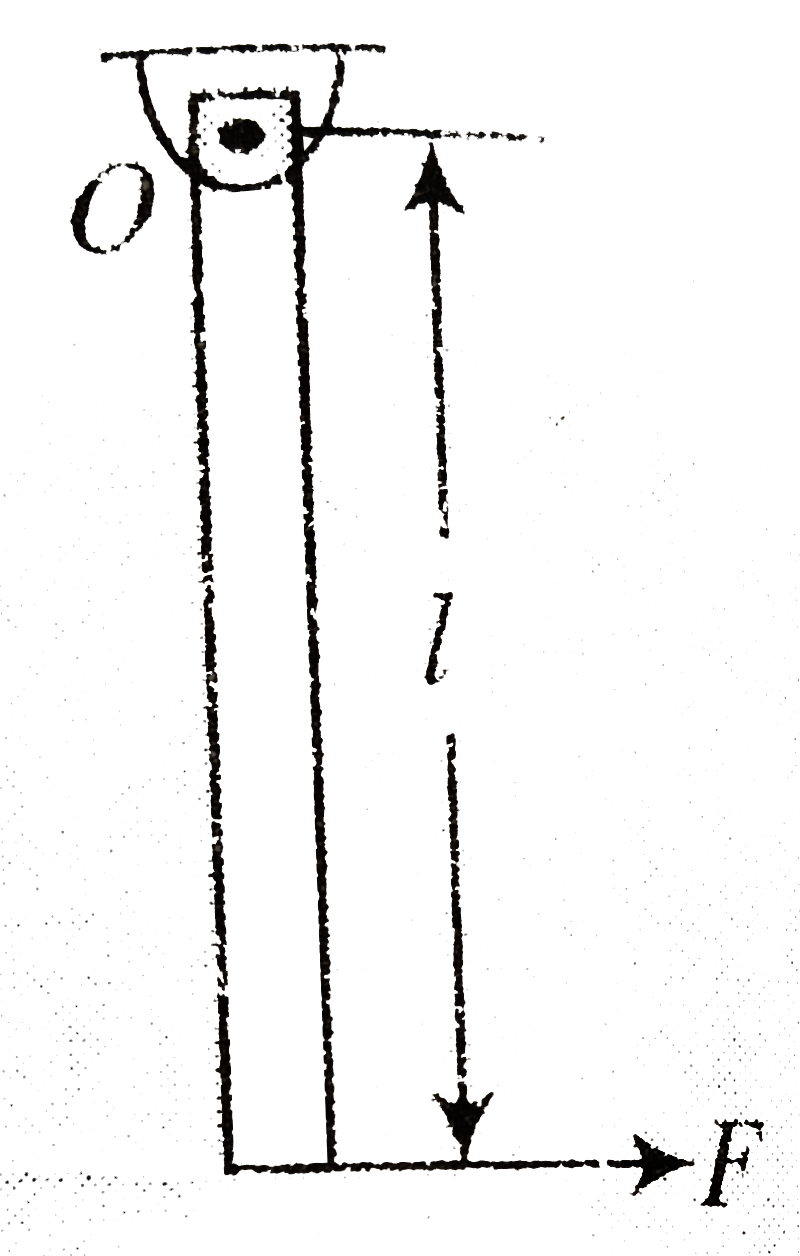
View SolutionA rod of mass m and length l is lying on a horizontal table. Work done in making it stand on one end will be
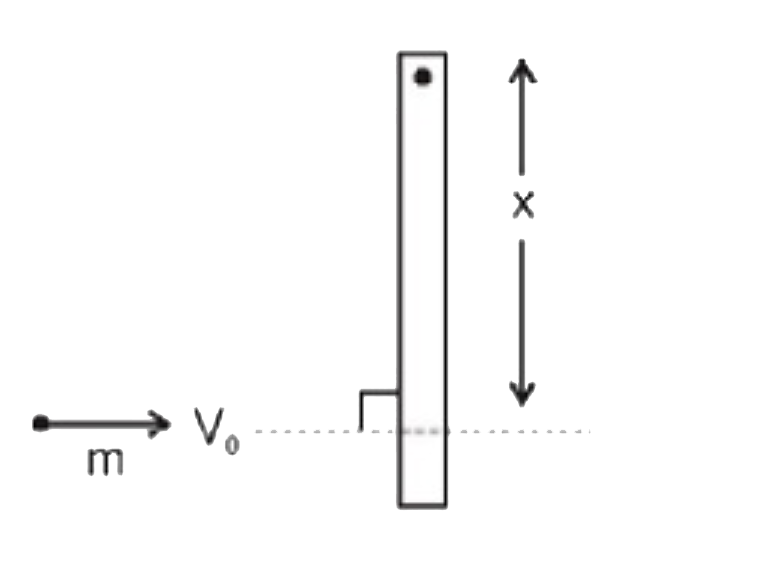
View SolutionA uniform rod of mass m and length L is hinged at one end and free to rotate in the horizontal plane. All the surface are smooth. A particle of same mass m collides with the rod with a speed V0. The coefficient of restitution for the collision is e=12. If hinge reaction during the collision is zero then the value of x is :
View Solution