A ball is thrown from ground level so as to just clear a wall 4 m high at a distance of 4 m and fall at a distance of 14 m from the wall. Find the magnitude and direction of the velocity of the ball.
To solve the problem, we need to find the magnitude and direction of the velocity of a ball thrown from ground level that just clears a wall 4 m high at a distance of 4 m, and then lands 14 m away from the wall.
1. Understand the Problem:
- The wall height (h) = 4 m
- Distance from the launch point to the wall (x1) = 4 m
- Distance from the wall to the landing point (x2) = 14 m
- Total horizontal range (R) = x1 + x2 = 4 m + 14 m = 18 m
2. Use the Range Formula:
The range R of a projectile is given by the formula:
R=V20sin(2α)g
where V0 is the initial velocity, α is the angle of projection, and g is the acceleration due to gravity (approximately 10m/s2).
Substituting the known values:
18=V20sin(2α)10
Rearranging gives:
V20sin(2α)=180(1)
3. Use the Equation of Trajectory:
The height y of the projectile at a horizontal distance x is given by:
y=xtan(α)−gx22V20cos2(α)
For the wall at x=4 m, y=4 m:
4=4tan(α)−10⋅422V20cos2(α)
Simplifying gives:
4=4tan(α)−80V20cos2(α)(2)
4. Relate tan(α) and cos(α):
From (1), we have:
sin(2α)=2sin(α)cos(α)
Thus, we can express tan(α) in terms of sin(α) and cos(α):
tan(α)=sin(α)cos(α)
5. Substituting Values:
From the trajectory equation (2), we can express tan(α) as:
4=4sin(α)cos(α)−80V20cos2(α)
This gives us a relationship between sin(α) and cos(α).
6. Finding tan(α):
From the previous steps, we can derive:
tan(α)=97⇒α=tan−1(97)
7. Finding sin(α) and cos(α):
Using the triangle formed by 9 and 7:
sin(α)=9√130,cos(α)=7√130
8. Substituting Back to Find V0:
Substitute sin(α) and cos(α) back into the range equation (1):
V20⋅2⋅9√130⋅7√130=180
Simplifying gives:
V20=180⋅130126⇒V0=√23400126=√13007
9. Final Result:
The magnitude of the initial velocity V0 is:
V0=√13007 m/s
The angle of projection α is:
α=tan−1(97)
- The wall height (h) = 4 m
- Distance from the launch point to the wall (x1) = 4 m
- Distance from the wall to the landing point (x2) = 14 m
- Total horizontal range (R) = x1 + x2 = 4 m + 14 m = 18 m
The range R of a projectile is given by the formula:
R=V20sin(2α)g
where V0 is the initial velocity, α is the angle of projection, and g is the acceleration due to gravity (approximately 10m/s2).
Substituting the known values:
18=V20sin(2α)10
Rearranging gives:
V20sin(2α)=180(1)
The height y of the projectile at a horizontal distance x is given by:
y=xtan(α)−gx22V20cos2(α)
For the wall at x=4 m, y=4 m:
4=4tan(α)−10⋅422V20cos2(α)
Simplifying gives:
4=4tan(α)−80V20cos2(α)(2)
From (1), we have:
sin(2α)=2sin(α)cos(α)
Thus, we can express tan(α) in terms of sin(α) and cos(α):
tan(α)=sin(α)cos(α)
From the trajectory equation (2), we can express tan(α) as:
4=4sin(α)cos(α)−80V20cos2(α)
This gives us a relationship between sin(α) and cos(α).
From the previous steps, we can derive:
tan(α)=97⇒α=tan−1(97)
Using the triangle formed by 9 and 7:
sin(α)=9√130,cos(α)=7√130
Substitute sin(α) and cos(α) back into the range equation (1):
V20⋅2⋅9√130⋅7√130=180
Simplifying gives:
V20=180⋅130126⇒V0=√23400126=√13007
The magnitude of the initial velocity V0 is:
V0=√13007 m/s
The angle of projection α is:
α=tan−1(97)
Similar Questions
A ball is thrown from the ground so that it just crosses a wall 5m high at a distance of 10m and falls at a distance of 10m ahead from the wall. Find the speed and the direction of projection of ball. Assume, g=10 ms–2
View SolutionA ball is thrown from the ground to clear a wall 3 m high at a distance of 6 m and falls 18 m away from the wall. Find the angle of projection of ball.
View SolutionA ball is thrown from the ground to clear a wall 3 m high at a distance of 6 m and falls 18 m away from the wall. Find the angle of projection of ball.
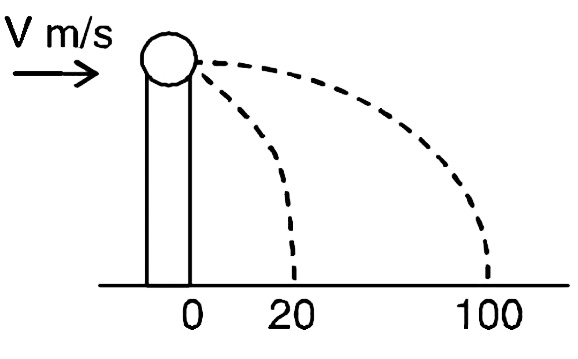
View SolutionA ball of mass 0.2 kg rests on a vertical post of height 5 m. A bullet of mass 0.01 kg, travelling with a velocity Vm/s in a horizontal direction, hits the centre of the ball. After the collision, the ball and bullet travel independently. The ball hits the ground at a distance of 20 m and the bullet at a distance of 100 m from the foot of the post. The velocity V of the bullet is
View SolutionA ladder is placed against a wall such that its foot is at a distance of 2.5 m from the wall and its top reaches a window 6 m above the ground. Find the length of the ladder.
View SolutionA ball is thrown from a point in level with velocity u and at a horizontal distance r from the top os a tower of height h.
At what horizontal distance x from the foot of the tower does the ball hit the ground ?
View SolutionA ball is projected from the ground with speed u at an angle α with horizontal. It collides with a wall at some distance from the point of projection and returns on the same level. Coefficient of restitution between the ball and the wall is e, then time taken in path AB and maximum height attained by the ball is

View SolutionA ball is projected from ground with a speed 70m/s at an angle 45∘ with the vertical so that it strikes a vertical wall at horizontal distance 4903m from the point of projection. If the ball returns back to the poin tof projection without any collision with ground then find the coefficient of restitution between the ball and wall.
View SolutionA ball is projected form ground with a speed of 20ms−1 at an angle of 45∘ with horizontal.There is a wall of 25m height at a distance of 10m from the projection point. The ball will hit the wall at a height.
View SolutionA 15 m long ladder reached a window 12 m highfrom the ground on placing it against a wall at a distance a. Find the distance of the foot of theladder from the wall.
View Solution
