A parallel plate capacitor has a dielectric (of dielectric constant k) between the plates. The plates are charged to a surface charge density σ0. What is the density of the polarized charge that appear on the surface of the dielectric.
To find the density of the polarized charge that appears on the surface of the dielectric in a parallel plate capacitor, we can follow these steps:
1. Understand the System: We have a parallel plate capacitor with plates charged to a surface charge density σ0 and a dielectric material with dielectric constant k placed between the plates.
2. Electric Field in the Dielectric: The electric field E inside the dielectric can be expressed as:
E=σ0kϵ0
where ϵ0 is the permittivity of free space.
3. Electric Field due to Free Charge: The electric field E0 due to the free charge on the plates (without the dielectric) is given by:
E0=σ0ϵ0
4. Electric Field due to Polarized Charge: Let σ′ represent the surface charge density of the polarized charge on the dielectric. The electric field E′ due to this polarized charge can be expressed as:
E′=σ′ϵ0
5. Net Electric Field: The net electric field E in the dielectric is the difference between the electric field due to the free charge and the electric field due to the polarized charge:
E=E0−E′
6. Substituting the Values: By substituting the expressions for E, E0, and E′ into the equation, we have:
σ0kϵ0=σ0ϵ0−σ′ϵ0
7. Rearranging the Equation: Multiplying through by ϵ0 to eliminate the denominator gives:
σ0k=σ0−σ′
8. Solving for Polarized Charge Density: Rearranging the equation to solve for σ′:
σ′=σ0−σ0k
σ′=σ0(1−1k)
9. Final Expression: This can be rewritten as:
σ′=σ0k−1k
Final Answer:
The density of the polarized charge that appears on the surface of the dielectric is:
σ′=σ0k−1k
E=σ0kϵ0
where ϵ0 is the permittivity of free space.
E0=σ0ϵ0
E′=σ′ϵ0
E=E0−E′
σ0kϵ0=σ0ϵ0−σ′ϵ0
σ0k=σ0−σ′
σ′=σ0−σ0k
σ′=σ0(1−1k)
σ′=σ0k−1k
The density of the polarized charge that appears on the surface of the dielectric is:
σ′=σ0k−1k
Similar Questions
Parallel Plate Capacitors with and without Dielectric
View SolutionA parallel plate capacitor has a dielectric slab of dielectric constant K between its plates that covers 1/3 of the area of its plates, as shown in the figure. The total capacitance of the capacitor is C while that of the portion with dielectric in between is C1. When the capacitor is charged, the plate area covered by the dielectric gets charge Q1 and the rest of the area gets charge Q2. The electric field in the dielectric is E1 and that in the other portion is E2. Choose the correct option/options, ignoring edge effects.
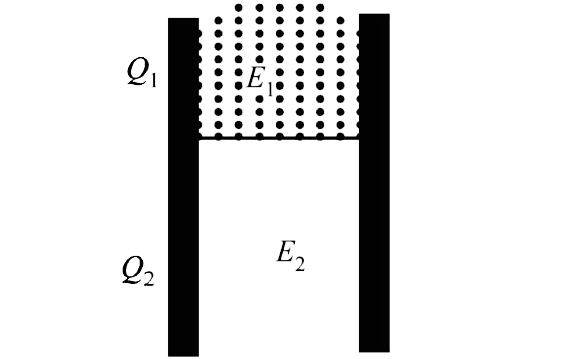
View Solution
Knowledge Check
A parallel plate condenser with a dielectric of dielectric constant K between the plates has a capacity C and is charged to a potential V volt. The dielectric slab is slowly removed from between the plates and then reinserted. The net work done by the system in this process is
AzeroB12(K−1)CV2CCV2(K−1)KD(K−1)CV2
An uncharged parallel plate capacitor having a dielectric of dielectric constant K is connected to a similar air core parallel plate capacitor charged to a potential V0. The two share the charge, and the common potential becomes V. The dielectric constant K is
View SolutionOn placing dielectric slab between the plates of an isolated charged condenser its
View SolutionA dielectric slab is inserted between the plates of a capacitor. The charge on the capacitor is Q and the magnitude of the induced charge on each surface of the dielectric is Q'.
View SolutionA parallel plate capacitor is half filled with a dielectric slab of dielectric constant (K) and mass M. Capacitor is connected to a cell of e.m.f. E. Plates are held fixed on horizontal surface. A bullet of mass M hits the dielectric elastically and it is found that the dielectric slab just leaves out the capacitor. Find the speed of the bullet?
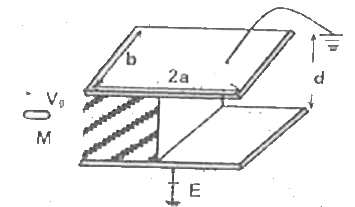
View SolutionAfter charging a capacitor the battery is removed. Now by placing a dielectric slab between the plates
View SolutionFind an expression for the capacitance of a parallel plate capacitor when a dielectric slab of dielectric constant K and thickness t=d2 but the same area on the plates is inserted between the the capacitors plate. (d=separation between the plates)
View SolutionA parallel plate capacitor with air as dielectric is charged to a potential .v. using a battery . Removing the battery. The charged capacitor is then connected across an identical uncharged parallel plate capacitor filled with was of dielectric constant k. The common potential of both the capacitor is
View Solution
