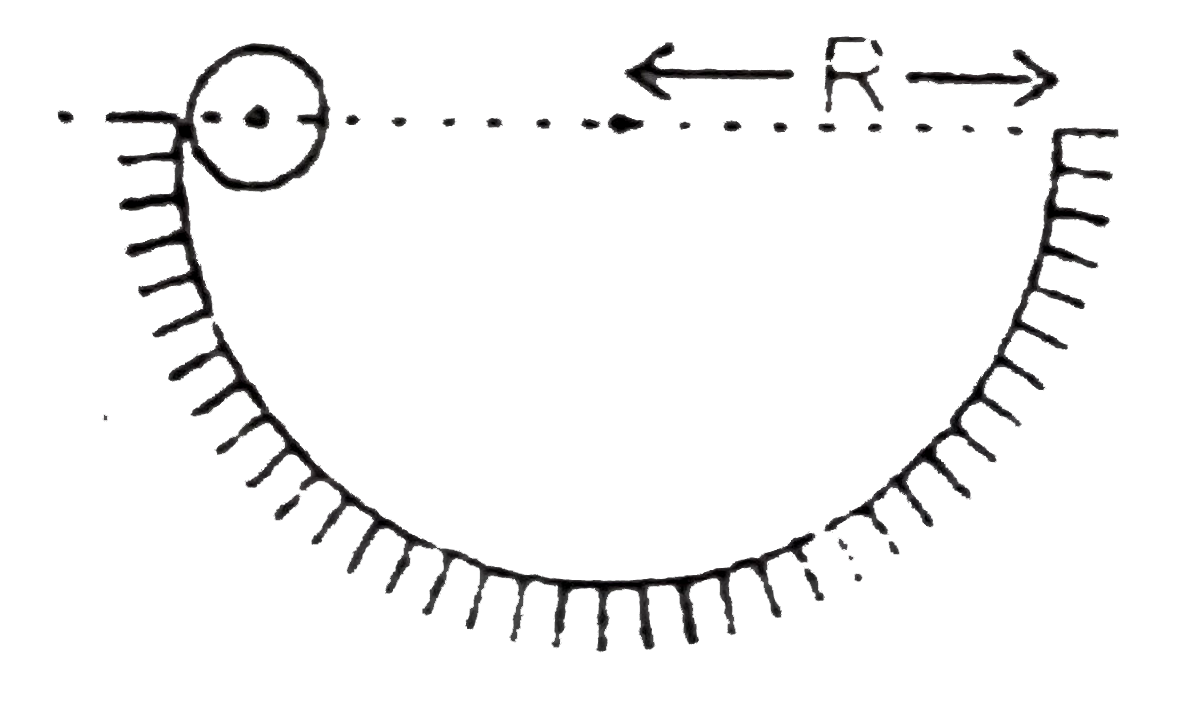
A circular tube of mass M placed veticlly on a horizontal surface as shown in the figure. Two small spheres, each of mass m , just fit in the tube, are released from the top. If θ gives the angle between radius vector of eirther ball with the vertical, obtain the value of the ratio M/m if the tube breacks its contact with ground when θ=60∘ . Neglect any friction.

Using conservation of energy principle, if v be the speed of either ball when its radius vector makes angle θ with vertically upward direction.
mgR[1−cosθ]=12mv2
⇒mv2R=2mg[1−cosθ]
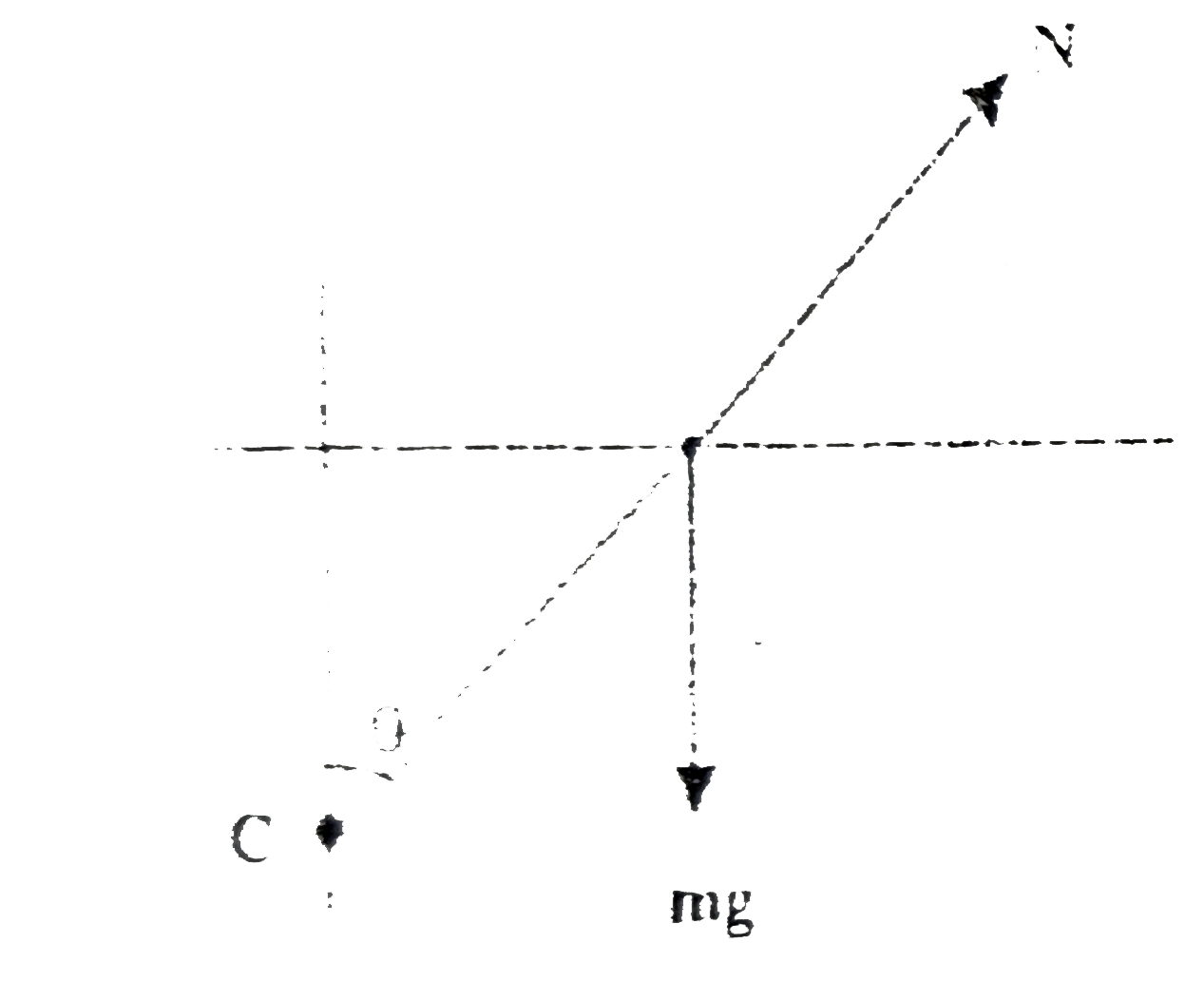
From F.B.D (i)
N=mgcosθ−mv2R
=mgcosθ−2mg[1−cosθ]
From F.B.D. (ii)
N'=2Ncosθ+Mg
At the instant tube breaks its contact with ground
N'=0
⇒Mg+[mgcosθ−2mg(1−cosθ)]2cosθ=0
for θ=60∘, we get mM=2.
Similar Questions
Two identical ladders, each of mass M and length L are resting on the rough horizontal surface as shown in the figure. A block of mass m hangs from P. if the system is in equilibrium, find the magnitude and the direction of frictional force at A and B.
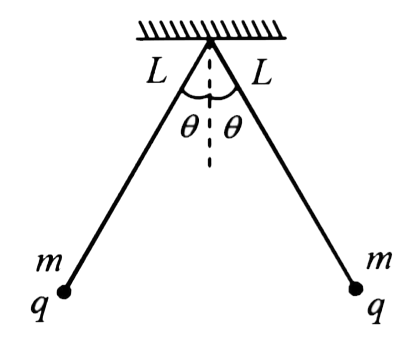
View SolutionTwo identical small charged spheres, each having a mass m, hang in equilibrium the length of each string is l, and the angle made by any string with the vertical is θ. Find the magnitude of the charge on each sphere.
View SolutionTwo small spheres each of mass m and charge q are tied from the same rigid support with the help of silk threads of length L. They make angle θ with the vertical as shown in the fig. If length L is decreased, then angle θ with the vertical.
View SolutionA block of mass M is placed on the top of a bigger block of mass 10 M as shown in figure. All the surfaces are frictionless. The system is released from rest, then the distance moved by the bigger block at the instant the smaller block reaches the ground :
View SolutionIn the figure shown, a small ball of mass 'm' can move witgout sliding in a fixed semicircular track of radius R in vertical planet. It is released from the top. The resultant force on the ball at the lowest of the track is
View SolutionA force F is applied at the top of a ring of mass M and radius R placed on a rough horizontal surface as shown in figure. Friction is sufficient to prevent slipping. The friction force acting on the ring is:

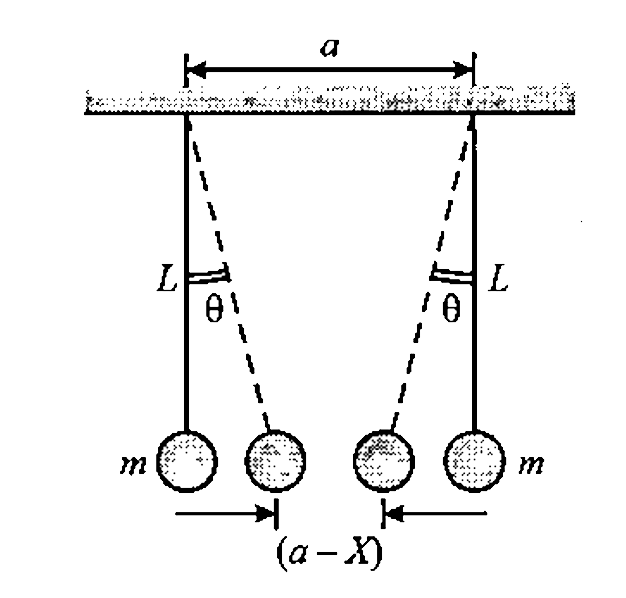
View SolutionTwo small balls of mass m each are suspended side by side by two equal threds to length L. If the distance between the upper ends of the threads be a, the angle θ that the threads will make with the vertical due to attraction between the balls is :
View SolutionA solid sphere of mass m and radius R is released from top of an incline having co-efficent of friction μ and making an angle of 45∘ with the horizontal. Choose the correct alternative (s)
View SolutionA small ball of mass m is released in a smooth circular tube as shown in figure. The minimum height h such that bead can complete the circle is
View SolutionFigure shows a flat car of mass M on a frictionless road. A small massless wedge is fitted on it as shown. A small ball of mass m is released from the top of the wedge, it slides over it and falls in the hole at distance l from the initial position of the ball. Find the distance the flat car moves till the ball gets into the hole.

View Solution