A person wants to drive on the vertical suirface of a large cylindrical wooden well commonly known as deathwell in a circus. The radius of the well 2 meter, and the coefficient of friction between the tyers of the motorcycle and the wall of the well is 0.2 the minimum speed the motorcyclist must have in order to prevent slipping should be
10m/s
15m/s
20m/s
25m/s
To solve the problem of determining the minimum speed a motorcyclist must have to prevent slipping while driving on the vertical surface of a cylindrical well, we can follow these steps:
Step 1: Identify the Forces Acting on the Motorcyclist
When the motorcyclist is riding on the vertical wall of the well, two main forces act on him:
- The gravitational force (Fg=mg), acting downward.
- The normal force (Fn), acting perpendicular to the wall of the well.
Step 2: Understand the Role of Friction
The frictional force (Ff) that prevents slipping is given by:
Ff=μFn
where μ is the coefficient of friction. In this case, μ=0.2.
Step 3: Apply Centripetal Force Concept
For the motorcyclist to move in a circular path, the centripetal force required is provided by the normal force. The centripetal force (Fc) is given by:
Fc=mv2r
where v is the speed of the motorcycle and r is the radius of the well (2 meters).
Step 4: Set Up the Equations
Since the frictional force must balance the gravitational force for the motorcyclist not to slip, we can write:
Ff=mg
Substituting the expression for friction:
μFn=mg
From the centripetal force equation, we know:
Fn=mv2r
Step 5: Equate the Forces
Substituting Fn into the friction equation gives:
μ(mv2r)=mg
Step 6: Simplify the Equation
We can cancel m from both sides (assuming m≠0):
μv2r=g
Step 7: Solve for Speed v
Rearranging the equation to solve for v:
v2=grμ
Taking the square root:
v=√grμ
Step 8: Substitute Known Values
Now, substituting the known values:
- g=10m/s2
- r=2m
- μ=0.2
We get:
v=√10×20.2
v=√200.2
v=√100
v=10m/s
Final Answer
The minimum speed the motorcyclist must have to prevent slipping is 10 m/s.
---
When the motorcyclist is riding on the vertical wall of the well, two main forces act on him:
- The gravitational force (Fg=mg), acting downward.
- The normal force (Fn), acting perpendicular to the wall of the well.
The frictional force (Ff) that prevents slipping is given by:
Ff=μFn
where μ is the coefficient of friction. In this case, μ=0.2.
For the motorcyclist to move in a circular path, the centripetal force required is provided by the normal force. The centripetal force (Fc) is given by:
Fc=mv2r
where v is the speed of the motorcycle and r is the radius of the well (2 meters).
Since the frictional force must balance the gravitational force for the motorcyclist not to slip, we can write:
Ff=mg
Substituting the expression for friction:
μFn=mg
Fn=mv2r
Substituting Fn into the friction equation gives:
μ(mv2r)=mg
We can cancel m from both sides (assuming m≠0):
μv2r=g
Rearranging the equation to solve for v:
v2=grμ
Taking the square root:
v=√grμ
Now, substituting the known values:
- g=10m/s2
- r=2m
- μ=0.2
v=√10×20.2
v=√200.2
v=√100
v=10m/s
The minimum speed the motorcyclist must have to prevent slipping is 10 m/s.
Similar Questions
A person wants to drive on the vertical surface of a large cylindrical wooden 'well' commonly known as 'death well' in a circus. The radius of the well is R and the coefficient of friction between the tyres of the motorcycle and the wall of the well is u. The minimum speed the motor cyclist must have in order to prevent slipping should be -
View SolutionA motercyclist wants to drive on the vertical surface of wooden 'well' of radius 5 m, with a minimum speed of 5√5ms−1. The minimum value of coefficient of friction between the tyres and the well must be (take, g = 10ms−2)
View SolutionA person stands in contact against the inner wall of a rotor of radius r. The coefficient of friction between the wall and the clothing is μ and the rotor is rotating about vertical axis. The minimum angular speed of the rotor so that the person does not slip downward is
View SolutionA horizontal force of 10N is necessary to just hold a block stationary against as well. The coefficient of friction between the block and the wall is 0.2. The weight of the block is
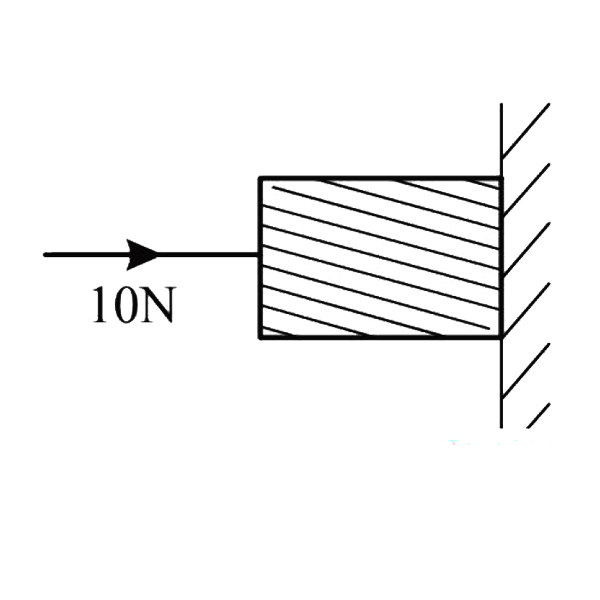
View SolutionA horizontal force of 10N is necessary to just hold a block stationary against as well. The coefficient of friction between the block and the wall is 0.2. The weight of the block is

View SolutionIn a rotor, a hollow vertical cylindrical structure rotates about its axis and a person rests against the inner wall. At a particular speed of the rotor, the floor below the person is removed and the person hangs resting against the wall without any floor. If the radius of the rotor is 2m and the coefficient of static friction between the wall and the person is 0.2, find the minimum speed at which the floor may be removed Take g=10ms2.
View SolutionIN a rotor, a hollow verticla cylindrical structure rotates about its axis and a person rests asgainst he inner wall. At a particular speed of the rotor, the floor below the person is removed and the person hangs resting against the wall without any floor. If the radius of the roter is 2m and the coefficient of static frictioin between the wall and theperson is 0.2, find the minimum speed at which the floor may be removed Take g=10ms2.
View SolutionIn the motorcycle stunt called " the well of death" the track is vertical cylindrical surface of 18 m radius.take the motorcyle to be a point mass and μ=0.8. The minimum angular speed of the motorcycle to prevent him from sliding down should be
View SolutionA 70kg man stands in contact against the inner wall of a hollow cylindrical drum of radius 3m rotating about its vertical axis. The coefficient of friction between the wall and his clothing is 0.15. What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed?
View SolutionA 70 kg man stands in contact against the wall of a cylindrical drum of radius 3 m rotating about its vertical axis with 200 rev/min. The coefficient of friction between the wall and his clothing is 0.15. What is the minimum rotational speed of the cylinder to enable the man to remain stuck to the wall (without falling) when the floor is suddenly removed ?
View Solution