A particle P is to be projected from a fixed point A on the ground with a fixed speed u. Another particle Q is also to be projected from a point B which is directly above the point A. The trajectory of the particle Q touches all possible trajectories (for different angle of projection) of the particle P in the vertical x−y plane. Find the equation of trajectory of the particle Q. (Take the point A as origin, horizontal axis x− axis and vertical axis y−axis Consider the figure.)
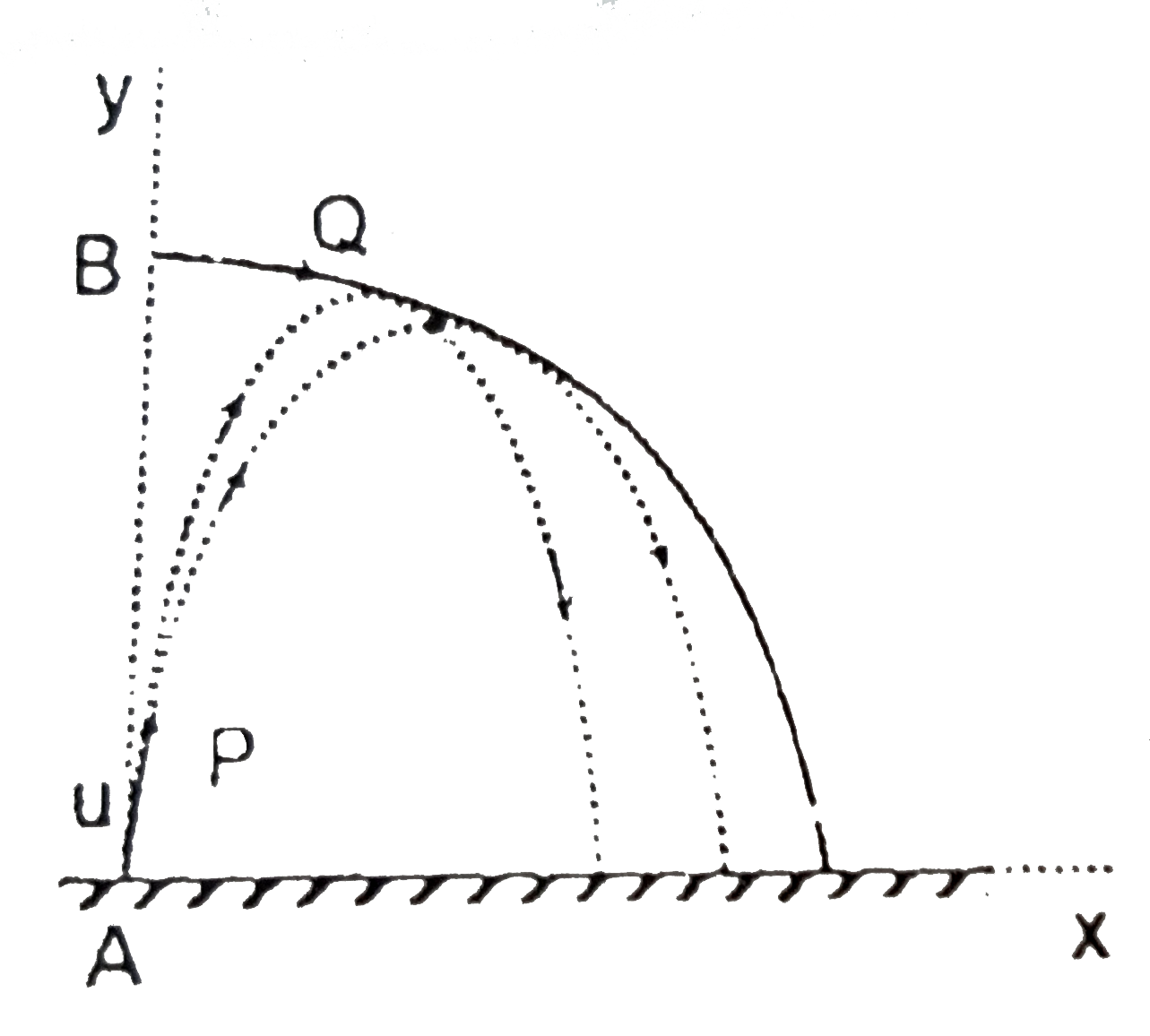
In order for the trahectory of Q to touch that of P when the latter is projected vertically upward, Q must be projected horizontally from the highest point. To visualize this, consider the trajectories of P as the angle of projection of P(θ,say), approaches 90∘ (i.e. for vertical projection).Let Q be projected horizontally from B with a speed v, where A=u32g. The point of impact of Q on the horizontal plane represents the point of maximum range for P along the horizontal.
OC=u2g=v√2hg, where h=u22g
i.e., v=u
The trajectory of Q is
u22g−y=12gt2. where t=x/v(v≡u)

Similar Questions
The ratio of the speed of a projectile at the point of projection to the speed at the top of its trajectory is x. The angle of projection with the horizontal is
View SolutionTwo particles P and Q are projected from two points in the same plane as shown. Find the condition for collision of two paricles.
View SolutionA particle is projected vertically upward with velocity u from a point A, when it returns to the point of projection .
View SolutionA particle of mass m is projected from the ground with an initial speed u at an angle α. Find the magnitude of its angular momentum at the highest point of its trajector about the point of projection.
View SolutionA particle of mass m is projected from the ground with an initial speed u at an angle α. Find the magnitude of its angular momentum at the highest point of its trajector about the point of projection.
View Solution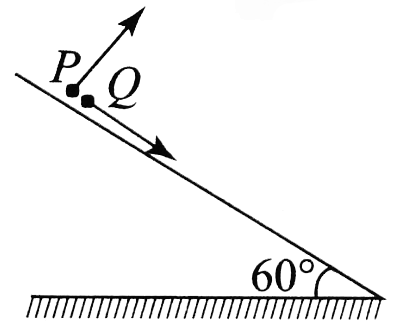
A particle P is projected from a point on the surface of smooth inclined plane (see figure). Simultaneously another particle Q is released on the smooth inclined plane from the same position. P and Q collide aftert=4. The speed of projection of P is
View Solution
A particle P is projected from a point on the surface of smooth inclined plane (see figure). Simultaneously another particle Q is released on the smooth inclined plane from the same position. P and Q collide aftert=4. The speed of projection of P is
View SolutionA particle is projected from a point on the level ground and its height is h when at horizontal distances aand2a from its point of projection. Find the velocity of projection.
View SolutionA particle is projected at angle θ with horizontal from ground. The slop (m) of the trajectory of the particle varies with time (t) as
View SolutionA particle of mass m is projected with a velocity v at an angle of θ with horizontal. The angular momentum of the particle at the highest point of its trajectory is equal to :
View Solution