Consider a wave sent down a string in the positive direction whose equation is given is
y=y0sin[ω(t−xv)]
The wave is propagated along because each string segment pulls upward and downward on the segment adjacent to it a slightly larger value of x and, as a result does work upon the string segment to which wave is travelling.
For example, the portion of string at point A is going upward, and will pull the portion at point B upward as well. In fact, at any point along the string, each segment of the string is pulling on the segment just adjacent and to its right, causing the wagve to propagate. It is by this process that the energy is sent along the string.
Now we try to calculate how much energy is propagated down the string per second
Ty=tsinθ≈Ttanθ⇒Ty=−T∂y∂x
(The negative sign appears because as shown in the figure II, the slope is negative) the force will act through a distance
dy=vydt=∂y∂tdt
Therefore work done by force in time dt is
dW=Tydy=−T(∂y∂x)(∂y∂t)dt⇒dW=ω2y20Tvcos2[ω(t−xV)]dt.....(A)
![Consider a wave sent down a string in the positive direction whose equation is given is y=y(0)sin[omega(t-x/v)] The wave is propagated along because each string segment pulls upward and downward on the segment adjacent to it a slightly larger value of x and, as a result does work upon the string segment to which wave is travelling. For example, the portion of string at point A is going upward, and will pull the portion at point B upward as well. In fact, at any point along the string, each segment of the string is pulling on the segment just adjacent and to its right, causing the wagve to propagate. It is by this process that the energy is sent along the string. Now we try to calculate how much energy is propagated down the string per second T(y)=tsintheta~~TtanthetaimpliesT(y)=-T(dely)/(delx) (The negative sign appears because as shown in the figure II, the slope is negative) the force will act through a distance dy=v(y)dt=(dely)/(delt) dt Therefore work done by force in time dt is dW=T(y)dy=-T((dely)/(delx))((dely)/(delt))dtimpliesdW=(omega^(2)y(0)^(2)T)/vcos^(2)[omega(t-x/V)]dt.....(A) How much average power is transmitted dawn a string having density mu=5xx10^(-4)kg//m and T=5N by a 200Hz vibration of amplitude of 0.20cm](https://d10lpgp6xz60nq.cloudfront.net/physics_images/FIT_JEE_PT3_P2_E01_316_Q01.png)
How much average power is transmitted dawn a string having density μ=5×10−4kg/m and T=5N by a 200Hz vibration of amplitude of 0.20cm
0.08 Watt
0.16 Watt
0.32 Watt
0.64 Watt
The correct Answer is:B
Since the work done will be same at any place along the string in one cycle so choose x=0, for convenience. Then work done in a cycle =ω2y20Tv∫2π/ω0cos2ωtdt=πωy20Tv
Average power = work done per unit time =work done in a cycleTime period=(πω20T).v2πω=ω2y20T2V
Average power =2π2f2y20T√μT=2π2f2y20√μT
=2×10×200×200×0.2×0.2×10−4√5×10−4×5=160×10−3 Watt =0.16 Watt.
Similar Questions
Consider a wave sent down a string in the positive direction whose equation is given is
y=y0sin[ω(t−xv)]
The wave is propagated along because each string segment pulls upward and downward on the segment adjacent to it a slightly larger value of x and, as a result does work upon the string segment to which wave is travelling.
For example, the portion of string at point A is going upward, and will pull the portion at point B upward as well. In fact, at any point along the string, each segment of the string is pulling on the segment just adjacent and to its right, causing the wagve to propagate. It is by this process that the energy is sent along the string.
Now we try to calculate how much energy is propagated down the stirng per second
Ty=tsinθ≈Ttanθ⇒Ty=−T∂y∂x
(The negative sign appears because as shown in the figure II, the slope is negative) the force will act through a distance
dy=vydt=∂y∂tdt
Therefore work done by force in time dt is
dW=Tydy=−T(∂y∂x)(∂y∂t)dt⇒dW=ω2y20Tvcos2[ω(t−xV)]dt.....(A)
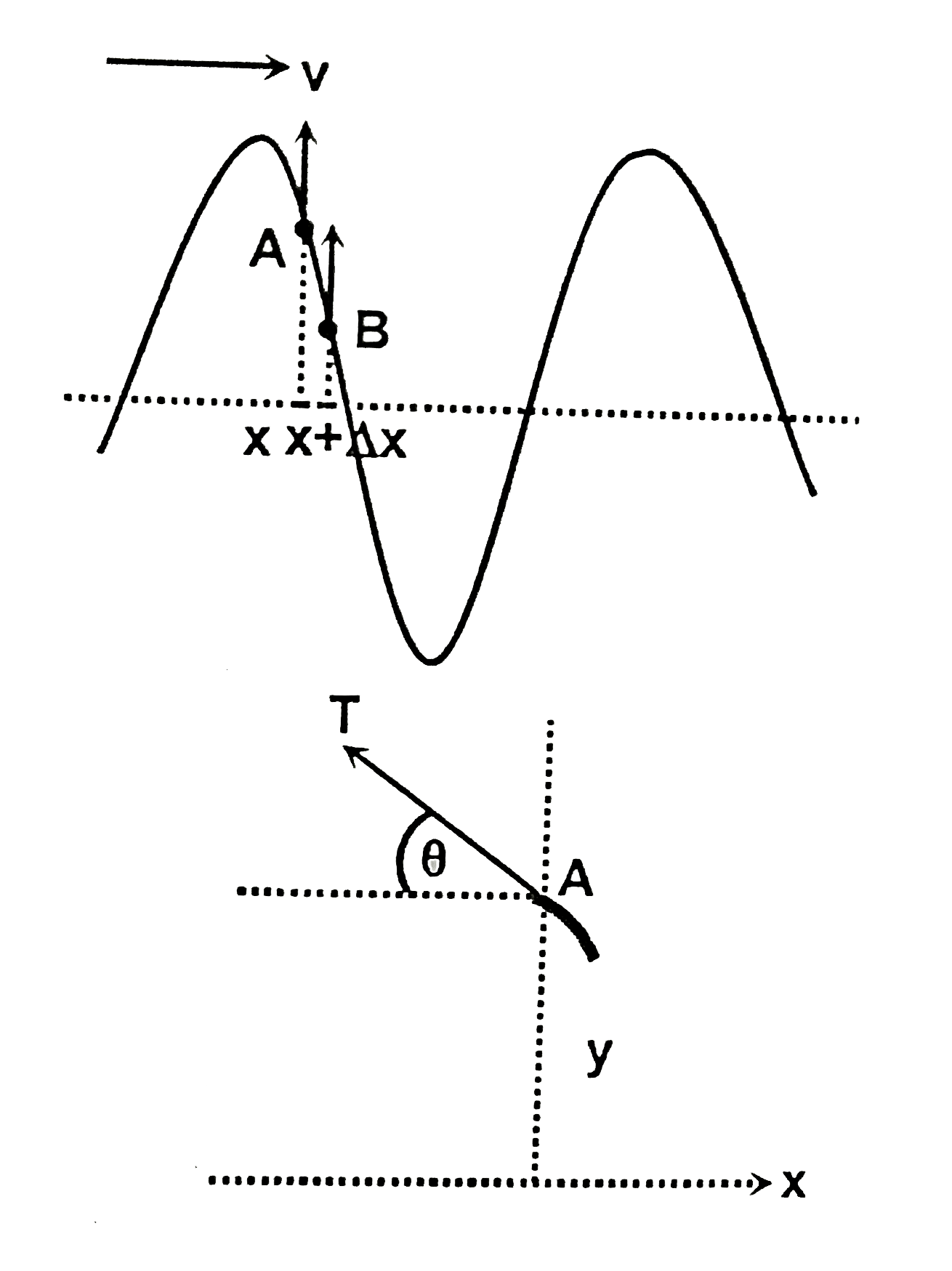
The average power transmitted down the string (i.e. the average energy transfer or sent down it per second) is
View SolutionA transverse wave is travelling on a string. The equation of the wave
View SolutionIn a stationary wave along a string the strain is
View SolutionA wave propagates in a string in the positive x-direction with velocity v. The shape of the string at t=t0 is given by
f(x,t0)=Asin(x2a2). Then the wave equation at any instant t is given by
View SolutionStationary waves are produced in 10 m long stretched string. If the string vibrates in 5 segments and wave velocity 20 m/s then the frequency is :-
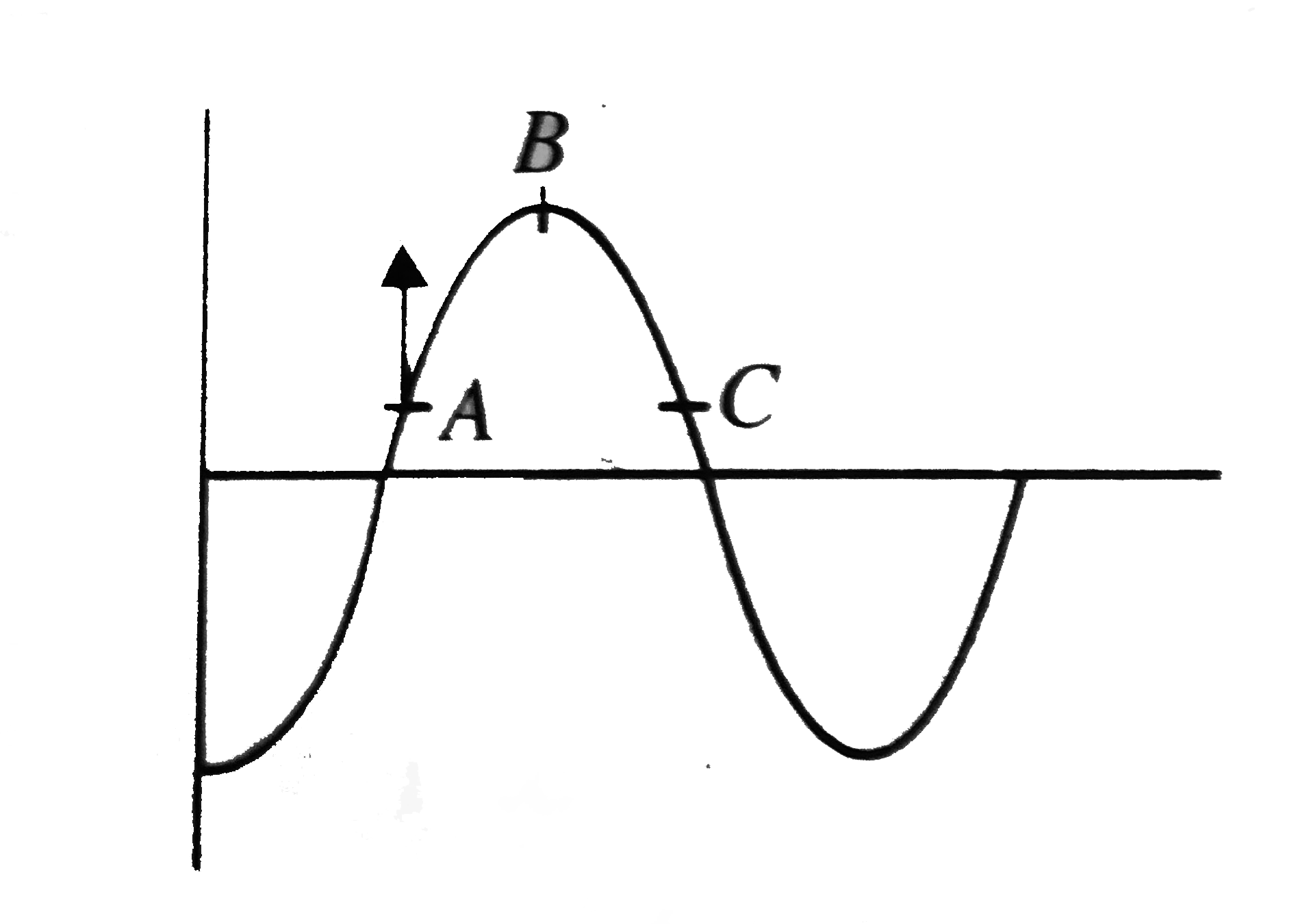
View SolutionA wave is travelling along a string. At an instant shape of the string is as shown in the enclosed figure. At this instant, point A is moving upwards. Which of the following statements are correct?
View SolutionA harmonic wave is travelling along +ve x-axis, on a stretched string. If wavelength of the wave gets doubled, then
View SolutionA travelling wave in a stretched string is described by the equation y=Asin(kx−ωt) the maximum particle velocity is
View SolutionAssertion: In a string wave, during reflection from fix boundary, the reflected wave is inverted.
Reason: The force on string by clamp is in downward direction while string is pulling the clamp is upward direction.
View SolutionA wave y=asin(ωt−kx) on a string meets with another wave producing a node at x=0. Then the equation of the unknown wave is
View Solution
