Magnetic field exist in the space and given as →B=−B0l2x2ˆk, where B0and l positive constants. A particle having positive charge 'q' and mass 'm' is pojected wit speed 'v0' along positive x axis from the origin. What is the maimum distance of the charged particle from the y-axis before it turns back due to the magnetic field. (Ignore any interaction other than magnetic field)
(ml2v03qB0)1/3
(3ml2v02qB0)1/3
(3ml2v0qB0)1/3
(ml2v0qB0)1/3
The correct Answer is:C
To solve the problem, we need to analyze the motion of a charged particle in a magnetic field. The magnetic field is given as:
→B=−B0l2x2^k
where B0 and l are positive constants. The particle has a positive charge q and mass m, and it is projected with an initial speed v0 along the positive x-axis from the origin.
Step 1: Determine the Force on the Charged Particle
The magnetic force acting on a charged particle moving in a magnetic field is given by the Lorentz force equation:
→F=q→v×→B
Here, the velocity vector →v is along the x-axis, which can be represented as:
→v=v0^i
Substituting →v and →B into the Lorentz force equation:
→F=q(v0^i)×(−B0l2x2^k)
Step 2: Calculate the Cross Product
Using the right-hand rule for the cross product, we have:
^i×^k=^j
Thus, the force becomes:
→F=−qB0l2x2v0^j
This indicates that the force acts in the negative y-direction.
Step 3: Write the Equation of Motion
The particle will experience a downward force due to the magnetic field, which will cause it to accelerate in the y-direction. The equation of motion in the y-direction can be written as:
md2ydt2=−qB0l2x2v0
Step 4: Analyze the Motion
Since the particle is moving along the x-axis, we can express x as a function of time:
x=v0t
Substituting this into the force equation gives:
md2ydt2=−qB0l2(v0t)2v0
This simplifies to:
md2ydt2=−qB0v30l2t2
Step 5: Integrate to Find the Position
Integrating once with respect to time:
dydt=−qB0v303ml2t3+C1
Integrating again gives:
y=−qB0v3012ml2t4+C1t+C2
Step 6: Determine Constants of Integration
At t=0, the particle starts at the origin, hence:
y(0)=0⟹C2=0
The initial velocity in the y-direction is zero, so:
dydt(0)=0⟹C1=0
Thus, the equation simplifies to:
y=−qB0v3012ml2t4
Step 7: Find Maximum Distance from the y-axis
The maximum distance from the y-axis occurs when the particle turns back, which is when y reaches its maximum value. This will happen when the particle stops moving in the y-direction, which will occur when the force acting on it becomes zero.
To find the maximum distance, we need to determine the time at which the particle turns back. This can be done by setting the acceleration to zero, which corresponds to the time when the particle reaches its maximum y-displacement.
Final Answer
The maximum distance from the y-axis can be calculated by substituting the time corresponding to the maximum y-displacement back into the equation for x:
xmax=v0tmax
This will yield the maximum distance of the charged particle from the y-axis before it turns back due to the magnetic field.
Similar Questions
From the surface of a round wire of radius a carrying a direct current i, a positive charge q escapes with a velocity v0 perpendicular to the surface. Find what the maximum distance of the electron will be from the axis of the wire before it turns back due to the action of the magnetic field generated by the current.
View SolutionSometimes positive charged particle comes from space towards earth with high velocity. Its deviation due to the magnetic field of earth will be:
View SolutionA particle of mass m and having a positive charge q is projected from origin with speed v0 along the positive X-axis in a magnetic field B = −B0ˆK, where B0 is a positive constant. If the particle passes through (0,y,0), then y is equal to
View SolutionA non-uniform magnetic field →B=B0(1+yd)(−ˆk) is present in the region of space between y=0 and y=d. A particle of mass m and positive charge q has velocity →v=3qB0dmˆi at origin O. Find the angle made by velocity of the particle withe the positive x-axis when it leaves the field. (Ignore any interaction other than magnetic field)
View SolutionA moving charged particle q travelling along the positive X-axis enters a uniform magnetic field B. When will the force acting on q be maximum?
View SolutionA positive charge particle having change q and mass m has velocity →v=v(ˆi+ˆk√2) in the magnetic field B at the origin . Its speed as the function of y is :
View SolutionA particle of charge q and mass m moving with a velocity v along the x-axis enters the region x>0 with uniform magnetic field B along the ˆk direction. The particle will penetrate in this region in the x-direction upto a distance d equal to
View SolutionA magnetic field B=B0ˆj exists in the region a<x<2a and B=−B0ˆj, in the region 2a<x<3a, where B0 is a positive constant. A positive point charge moving with as velocity v=v0ˆi, where v0 is a positive constant, enters the magnetic field at x=a, the trajectory of the charge in this region can be like:

View SolutionA particle of mass m and positive charge q is projected with a speed of v0 in y–direction in the presence of electric and magnetic field are in x–direction. Find the instant of time at which the speed of particle becomes double the initial speed.
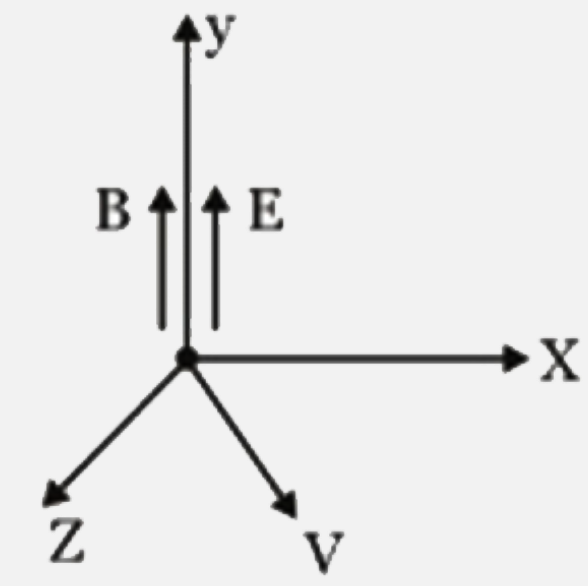
View SolutionThe space has electromagnetic field which is given as →B=−B0ˆk and →E=E0ˆk. A charged particle having mass m and positive charge q is given velocity v0ˆi at origin at t=0sec. The z coordinate of the particle when it passes through the z−axis is (neglect gravity through motion)
View Solution
