A rope AB of linear mass density λ is placed on a quarter vertical fixed disc of radius R as shown in the figure. The surface between the disc and rope is rough such that the rope is just is equilibrium. Gravitational acceleration is g. Choose the correct option (s).

Coefficient of static friction between rope and disc is μ=1
Coefficient of static friction between rope and disc is μ=1√2
Maximum tension in the rope is at the top most point A of the rope
Maximum tension in the rope is λRg(√2−k1)
The correct Answer is:A, D
For equilibrium
λRg∫π20cosθdθ=μλRg∫π20sinθdθ
∴μ=1
At the position of maximum tension in the rope
λRdθgcosθ=μ(λRdθgsinθ)
∴θ=45∘
At any θ
dT=λRdθgcosθ−μλdθgsinθ
∫Tmax0dT=λRg∫π40(cosθ−sinθ)dθ
Tmax=λRg[sinθ+cosθ]π40=λRg[1√2+1√2−1]=λRg(√2−1)

Similar Questions
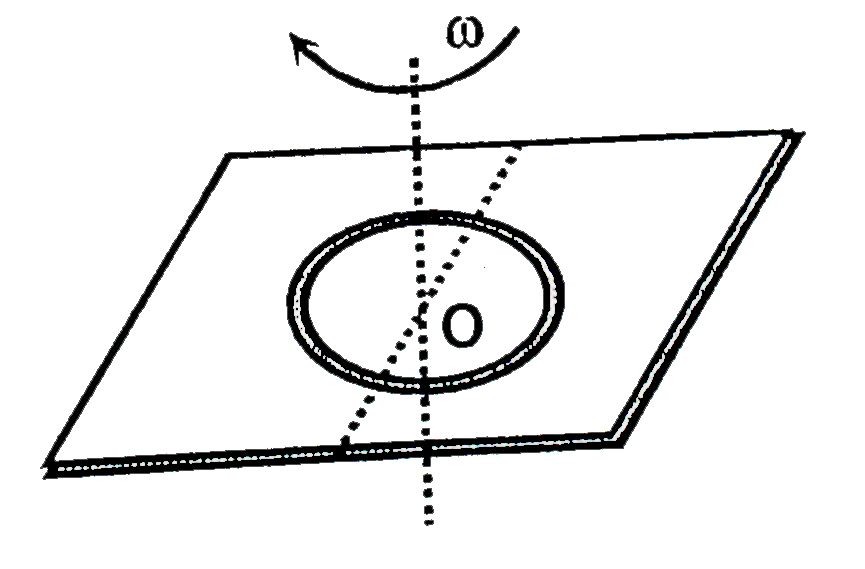
A thin annular disc of outer radius R and R2 inner radius has charge Q uniformly distributed over its surface. The disc rotates about its axis with a constant angular velocity ω. Choose the correct option(s):
View SolutionA solid cylinder of mass M and radius R pure rolls on a rough surface as shown in the figure. Choose the correct alternative (s).

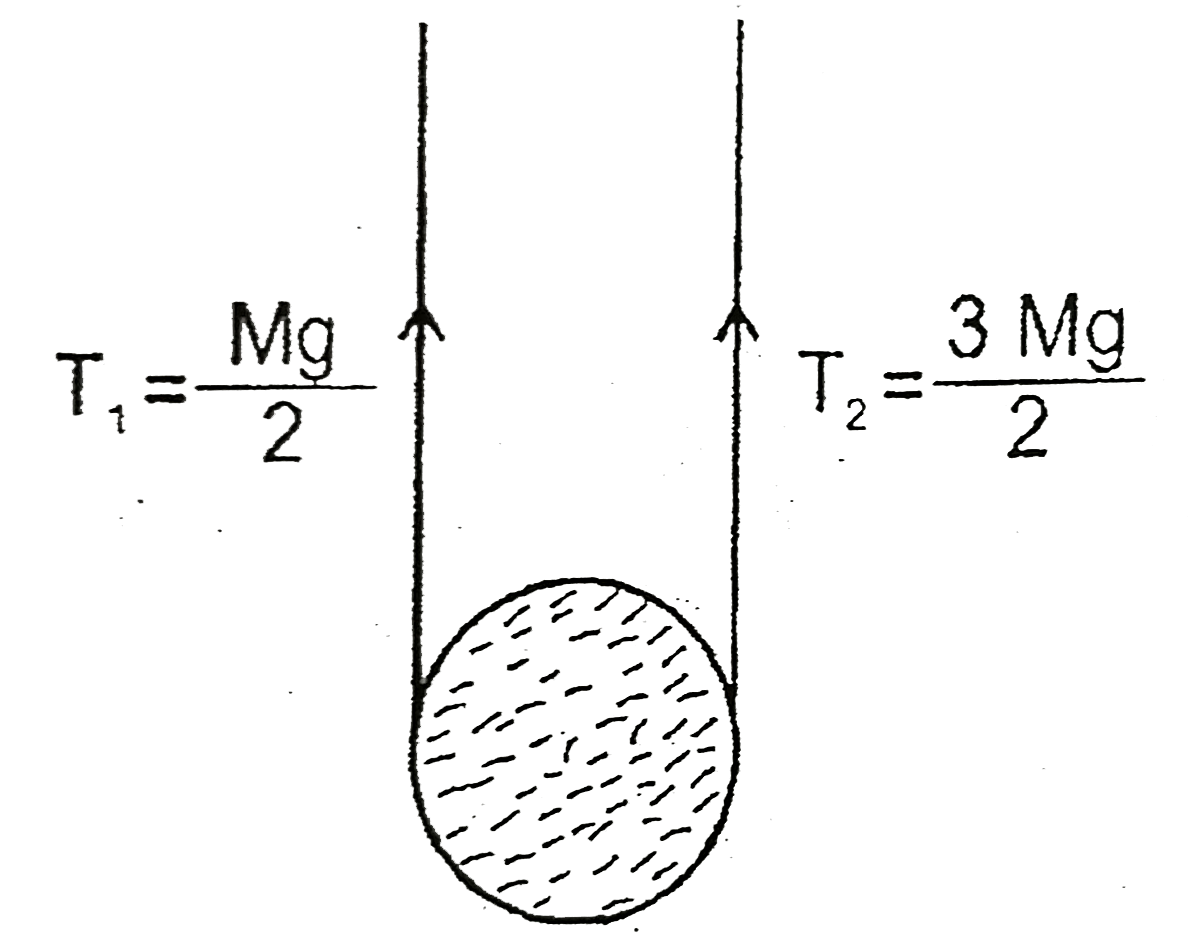
View SolutionA uniform disc of mass M and radius R is liffted using a string as shown in the figure. Then choose incorrect option(s),
View SolutionA particle of mass 'm' is attached to the rim of a uniform disc of mass 'm' and radius R. The disc is rolling wihtout slipping on a stationery horizontal surface as shown in the figure. At a particular instant, the particle is at the topmost position and centre of the disc has speed v0 amd its angular speed is ω. Choose the correct option (s).
View SolutionA disc of radius R and mass M is under pure rolling under the action of a force F applied at the topmost point as shown in figure. There is sufficient friciton between the disc and the horizontal surface. The acceleration is given as

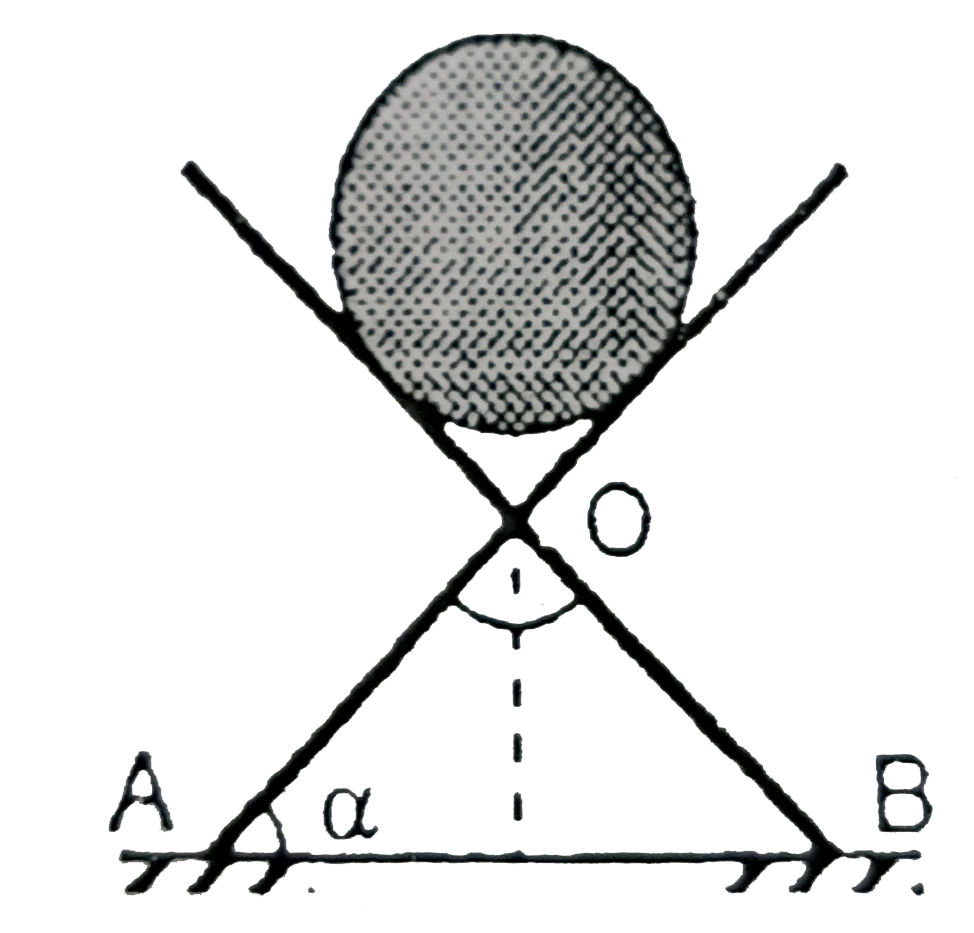
View SolutionA disc of radius a and mass m rests between two light rods, which are smoothly hinged together at point O with their ends A and B resting on a smooth table and are maintained in equilibrium by string AB as shown in the figure. Choose the correct option(s).
View SolutionA spherical hole of radius R/2 is excavated from the asteroid of mass M as shown in the figure the gravitational acceleration at a point on the surface of the asteroid just above the excavation is

View SolutionA uniform ring of mass m is placed on a rough horizontal fixed surface as shown in the figure. The coefficient of friction between the left part of the ring and left part of the horizontal surface is μ1=0.6π and between right half and the surface is μ2=0.2π. At the instant shown, now the ring has been imparted an angular velocity in clockwise sense in the figure shown. At this moment magnitude of acceleration of centre O of the ring (in m/s2) is (take g=10m/s2)
View SolutionA uniform cylinder of mass M and radius R is placed on a rough horizontal board, which in turn is placed on a smooth surface. The coefficient of friction between the board and the cylinder is μ. If the board starts accelerating with constant acceleration a, as shown in the figure, then

Option1
for pure rolling motion of the cylinder, direction of frictional force is forward and its magnitude is M a / 3
Option2
the maximum value of a, so that cyliner performs pure rolling is 3 μ g
Option3
The acceleration of the centre of mass of te cylinder under pure rolling condition for the given a is a / 3
Option4
none of these
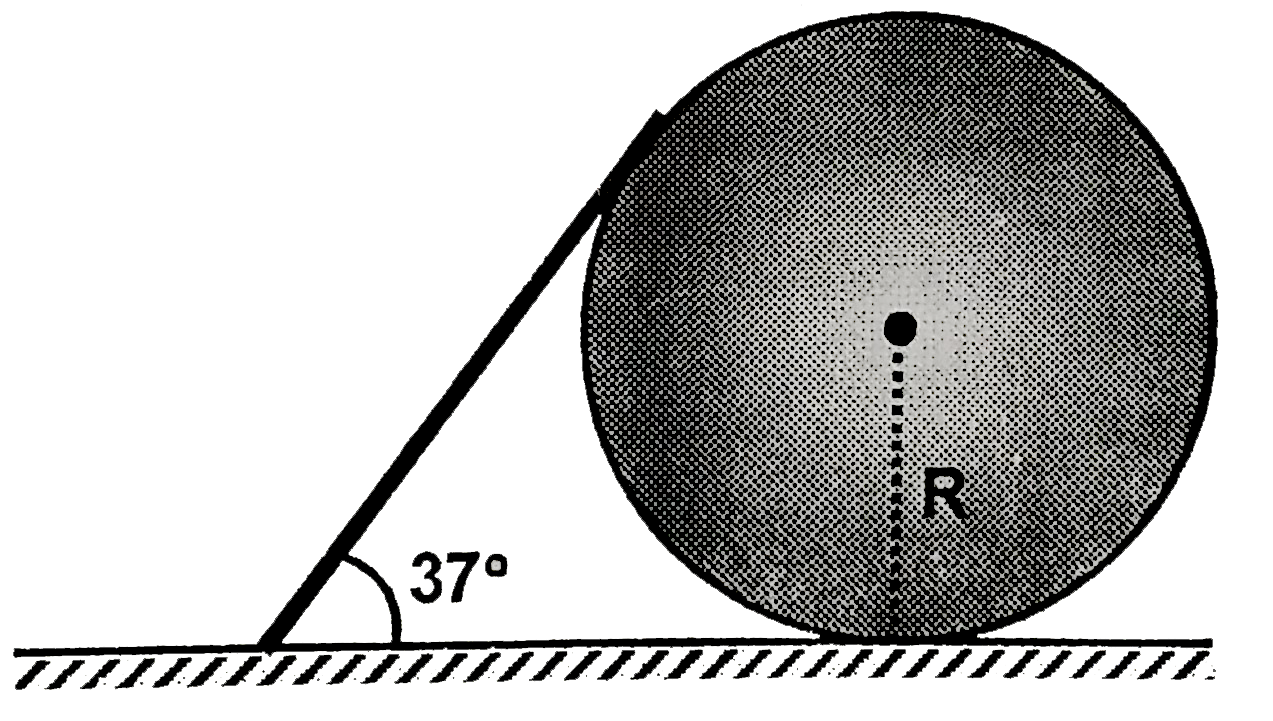
View SolutionA stick o fmas density λ=8kg/m rests on a disc of radius R=20cm as shown in the figure. The stick makes an angle θ=37∘ with the horizontal and is tangent to the disc at its upper end. Friction exists at all point of contact and assume that it is large enough to keep the system at rest. Find the friction force (in Newton) between the ground and the disc. (take g=10m/s2)
View Solution
