There is a disc of mass M and radius R. A pont charge +Q and of mass m is fixed at the centre of the disc. The disc is held on a fixed horizontal rough suface . Another point charged +q is fixed on the surface such that disance between +q and +Q is 4R3. Now the disc is set free. If the disc does not loose contact with the ground at the same intent the relation must hold is M>ηm. Find η. (Use 27Qq256πε0R2=3mg)

The correct Answer is:2
(M+m)g>FEsinθ
>27Qq256πε0R2
(M+m)g>3mg
M>2m→η=2

Similar Questions
A point charge q is placed at a distance d from the centre of a circular disc of radius R. Find electric flux flowing through the disc due to that charge
View SolutionA small hole is made in a disc of mass M and radius R at a distance R/4 from centre. The disc is supported on a horizontal peg through this hole. The moment of inertia of the disc about horizontal peg is
View SolutionA uniform circular disc of mass M and radius R is pivoted at distance x above the centre of mass of the disc, such that the time period of the disc in the vertical plane is infinite. What is the distance between the pivoted point and centre of mass of the disc ?
View SolutionOut of a disc of mass M and radius R a concentric disc of mass m and radius r is removed. The M.I. of the remaining part about the symmetric axis will be :
View SolutionThe moment of inertia of a disc of mass M and radius R about an axis. Which is tangential to sircumference of disc and parallel to its diameter is.
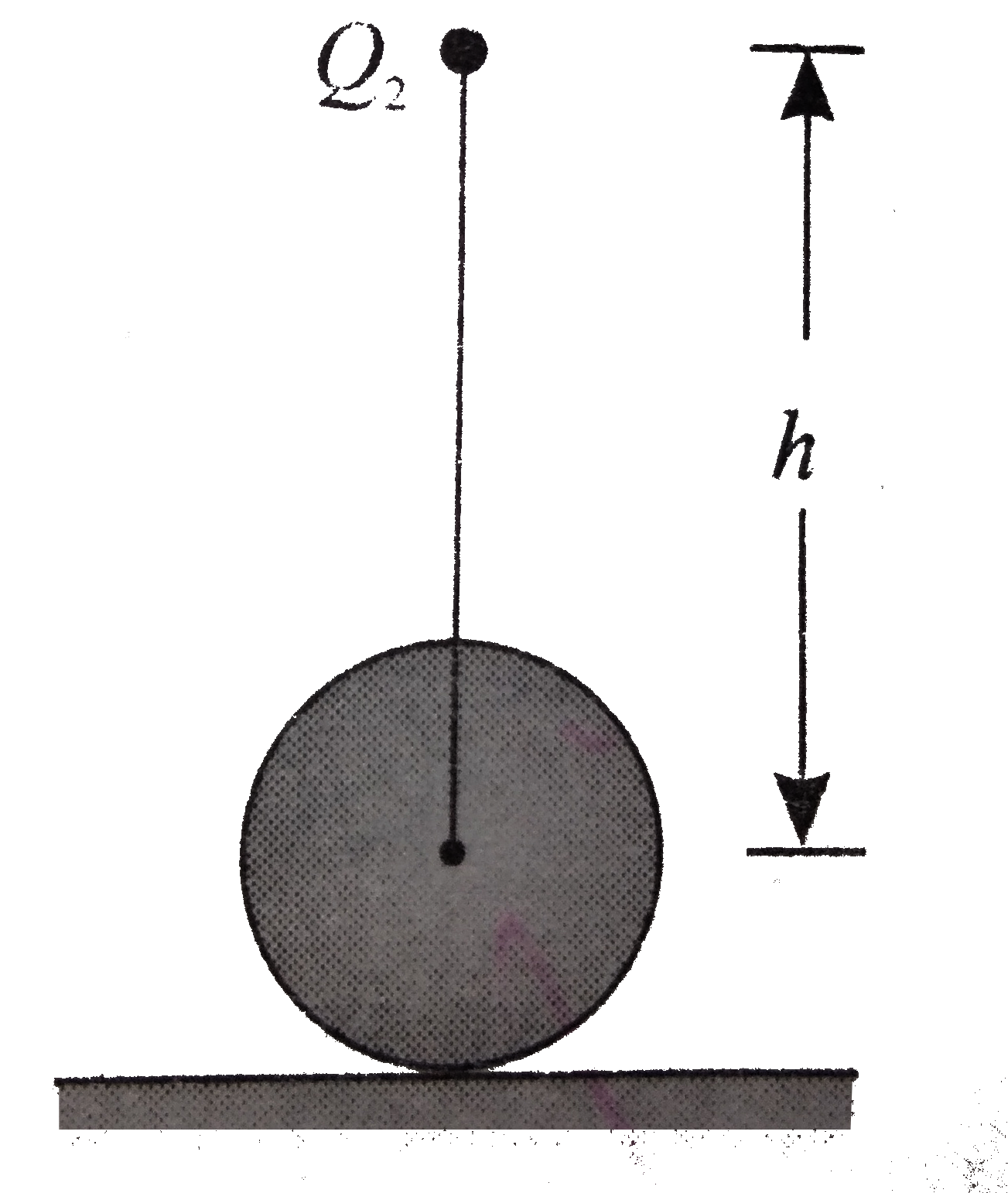
View SolutionA point charge Q1=−125μC is fixed at the center of an insulated disc of mass 1 kg. The disc rests on a rough horizontal plane. Another charge Q2=125μC is fixed verically above the center of the disc at a height h=1m. After the disc is displaced slightly in the horizontall direction (friction is sufficient to prevent slipping), find the period of oscillation of disc.
View SolutionA uniform disc of mass M and radius R is hinged at its centre C . A force F is applied on the disc as shown . At this instant , angular acceleration of the disc is

View SolutionA disc of mass M and radius R rolls on a horizontal surface and then rolls up an inclined plane as shown in the figure. If the velocity of the disc is v, the height to which the disc will rise will be:

View SolutionThe moment of inertia of a uniform semicircular disc of mass M and radius r about a line perpendicular to the plane of the disc through the center is
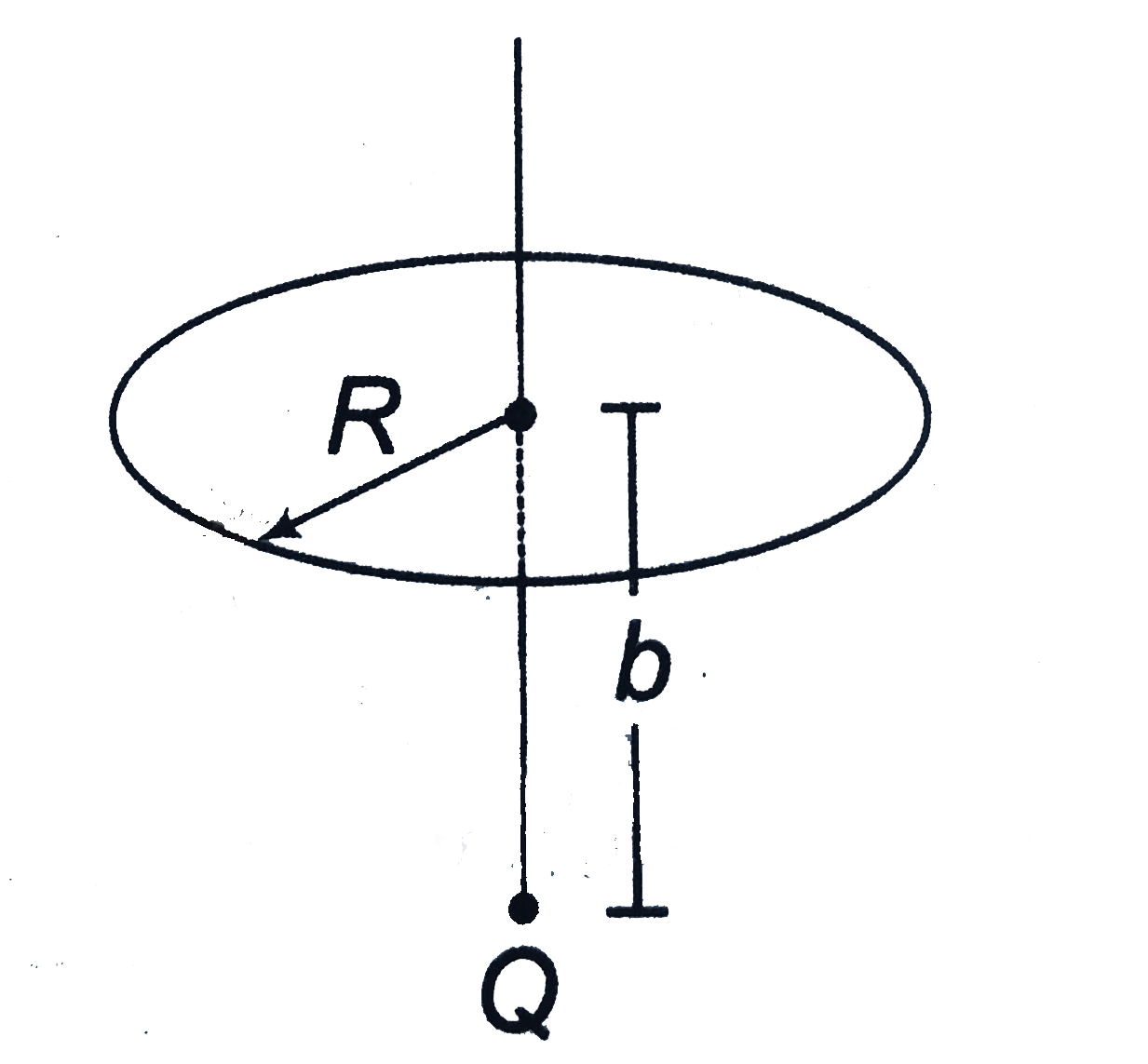
View SolutionA point charge Q is located on the axis of a disc of radius R at a distance b from the plane of the disc (figure). Show that if one-fourth of the electric flux from the charge passes through the disc, then R=√3b.
View Solution
