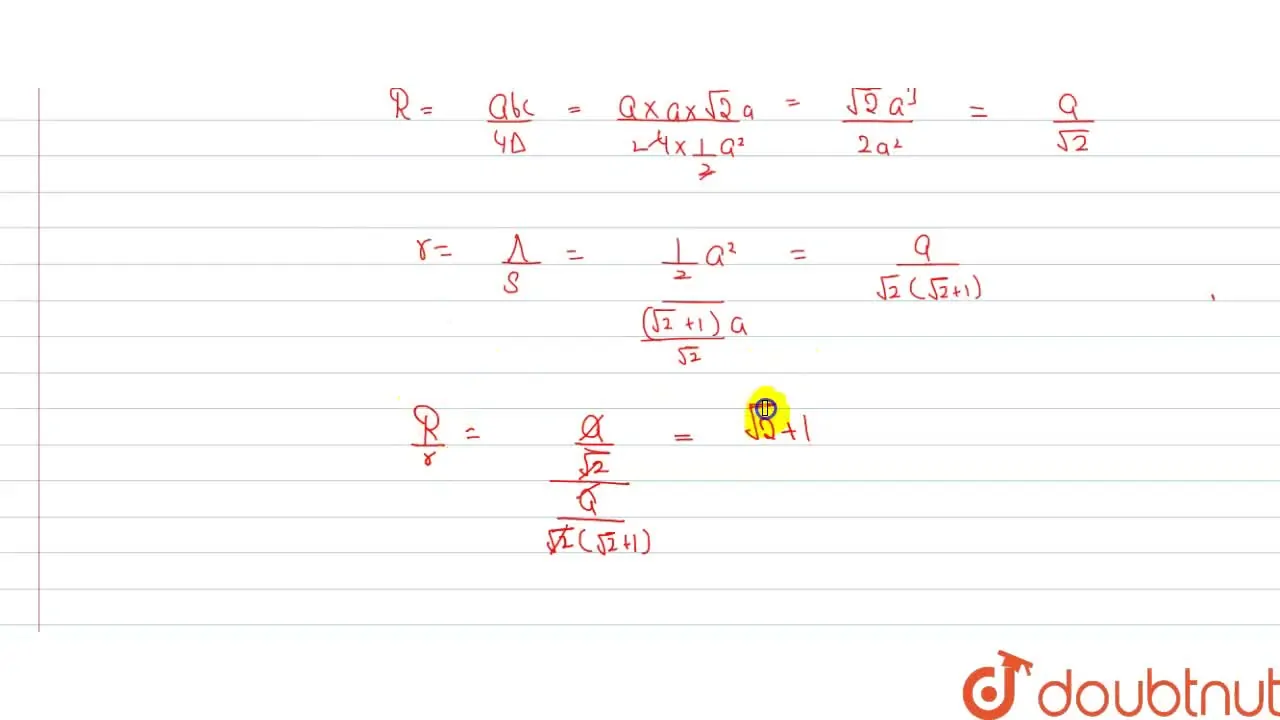
In a right - angled isosceles triangle , the ratio of the circumradius and inradius is

$2 (\sqrt{2} + 1) : 1$

$(s q r T (2) + 1) : 1$

$2 : 1$

$\sqrt{2} : 1$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Answer

Step by step video, text & image solution for In a right - angled isosceles triangle , the ratio of the circumradius and inradius is by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 MATHS SOLUTIONS AND PROPERTIES OF TRIANGLES

Topper's Solved these Questions

BOOK - ALLENCHAPTER - Solutions of Triangle & Binomial TheoremEXERCISE - EXERCISE-II
11videos
Solutions of Triangle & Binomial Theorem
BOOK - ALLENCHAPTER - Solutions of Triangle & Binomial TheoremEXERCISE - EXERCISE (O-1)
11videos
Solutions of Triangle & Binomial Theorem
BOOK - ALLENCHAPTER - Solutions of Triangle & Binomial TheoremEXERCISE - Illustration
28videos
Solutions of Triangle & Binomial Theorem
BOOK - ALLENCHAPTER - SOLUTION AND PROPERTIES OF TRIANGLEEXERCISE - All Questions
106videos
SOLUTION AND PROPERTIES OF TRIANGLE
BOOK - ALLENCHAPTER - TRIGNOMETRIC RATIOS AND IDENTITIESEXERCISE - All Questions
1videos
TRIGNOMETRIC RATIOS AND IDENTITIES

Knowledge Check

Question 1 - Select One
$Δ A B C$ is a right angled isosceles triangle and $∠ B$ is the right angle in the triangle. If AC measures $7 \sqrt{2}$ , then which one of the lengths of AB and BC, respectively?
A7,7
B9,9
C10,10
D11,12