Let PQR be a right - angled isosceles triangle , right angled at P(2,1). If the equation of the line QR is $2 x + y = 3$ , then the equation representing the pair of lines PQ and PR is

A

$3 x^{2} - 3 y^{2} + 8 x y + 20 x + 10 y + 25 = 0$

B

$3 x^{2} = 3 y^{2} + 8 x - 20 x - 10 y + 25 = 0$

C

$3 x^{2} - 3 y^{2} + 8 x y + 10 x + 15 y + 20 = 0$

D

$3 x^{2} - 3 y^{2} - 8 x y - 15 y - 20 = 0$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:2

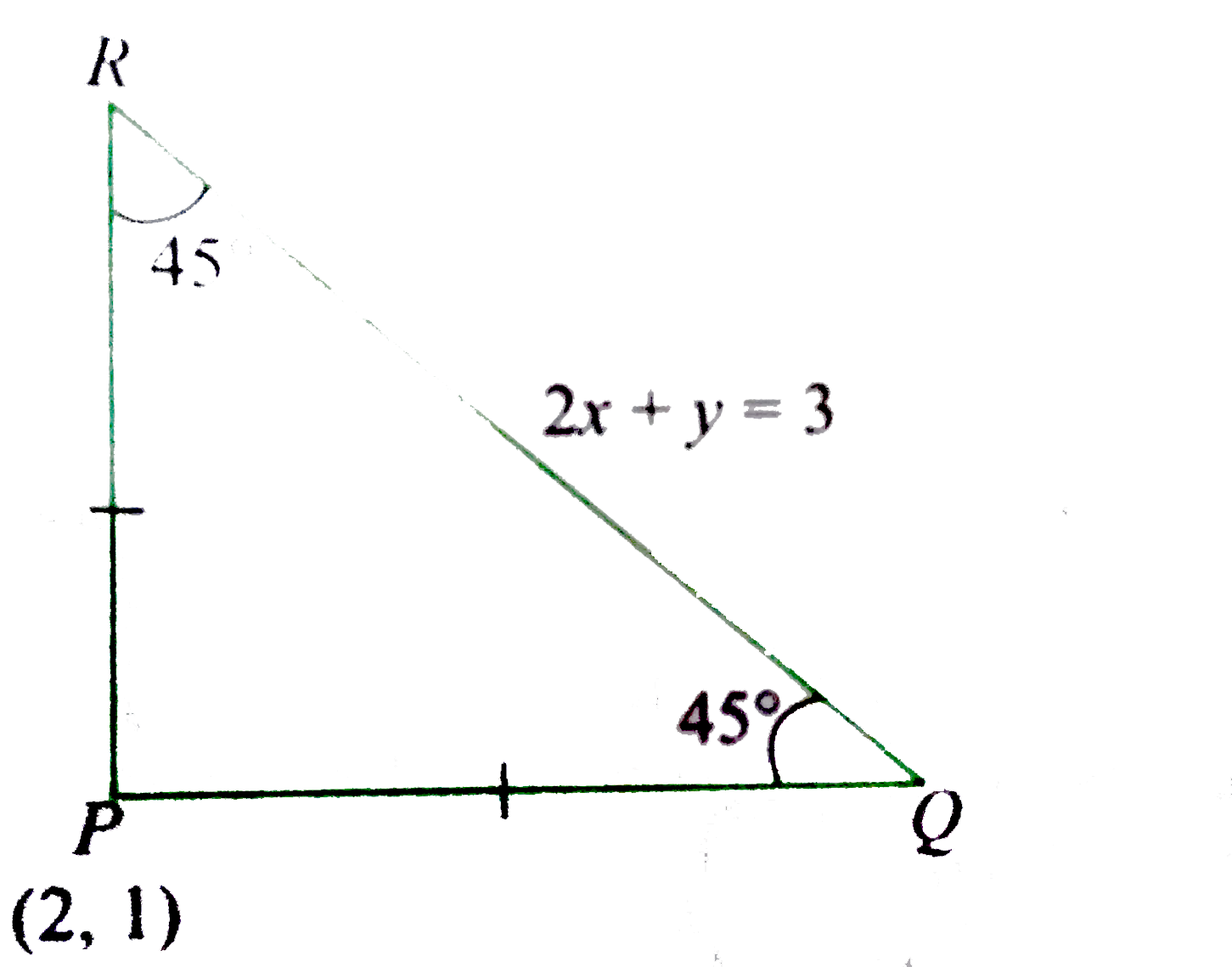
Let m be the slope of PQ . Then ,
${tan 45}^{\circ} = ∣ ∣ ∣ \frac{m - (- 2)}{1 + m (- 2)} ∣ ∣ ∣ = ∣ ∣ ∣ \frac{m + 2}{1 - 2 m} ∣ ∣ ∣$
$∴ m + 2 = 1 - 2 m or - 1 + 2 m = m + 2$
$∴ m = - \frac{1}{3} or m = 3$
Hence , the equation of PQ is
$y - 1 = - \frac{1}{3} (x - 2)$
or $x + 3 y - 5 = 0$ and the equation of PR is
$y - 1 = 3 (x - 2)$
or $3 x - y - 5 = 0$
Hence , the combined equation of PQ and PR is
$(x + 3 y - 5) (3 x - y - 5) = 0$
or $3 x^{2} - 3 y^{2} + 8 x y - 20 x - 10 y + 25 = 0$

Updated on:21/07/2023

|

Class 12 MATHS PAIR OF STRAIGHT LINES

Topper's Solved these Questions

Similar Questions

CENGAGE ENGLISH-PAIR OF STRAIGHT LINES-Single Correct Answer type

Home
Ask Doubt
Profile