The emf of a battery is 2V and its internal resistance is 0.5Ω the maximum power which it can deliver to any external circuit will be
The correct Answer is:(i) straight line (ii) x2(L2−r)2+y2r2=1
To find the maximum power that a battery can deliver to an external circuit, we can use the formula for maximum power transfer, which states:
Pmax=E24r
where:
- Pmax is the maximum power,
- E is the electromotive force (emf) of the battery,
- r is the internal resistance of the battery.
1. Identify the Given Values:
- The emf of the battery, E=2V
- The internal resistance, r=0.5Ω
2. Substitute the Values into the Formula:
Pmax=E24r
Substituting the values we have:
Pmax=(2V)24×0.5Ω
3. Calculate E2:
(2V)2=4V2
4. Calculate the Denominator:
4×0.5Ω=2Ω
5. Calculate Pmax:
Pmax=4V22Ω=2W
6. Conclusion:
The maximum power that the battery can deliver to any external circuit is 2W.
Final Answer:
The maximum power that can be delivered to any external circuit is 2 Watts.
- Pmax is the maximum power,
- E is the electromotive force (emf) of the battery,
- r is the internal resistance of the battery.
- The emf of the battery, E=2V
- The internal resistance, r=0.5Ω
Pmax=E24r
Substituting the values we have:
Pmax=(2V)24×0.5Ω
(2V)2=4V2
4×0.5Ω=2Ω
Pmax=4V22Ω=2W
The maximum power that the battery can deliver to any external circuit is 2W.
The maximum power that can be delivered to any external circuit is 2 Watts.
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:ALLENChapter:CURRENT ELECTRICITYExercise:EXERCISE-IV B
Explore 14 Videos
- CURRENT ELECTRICITYBook:ALLENChapter:CURRENT ELECTRICITYExercise:EXERCISE-V A
Explore 20 Videos
- CURRENT ELECTRICITYBook:ALLENChapter:CURRENT ELECTRICITYExercise:EXERCISE-III
Explore 26 Videos
- CIRCULAR MOTIONBook:ALLENChapter:CIRCULAR MOTIONExercise:EXERCISE (J-A)
Explore 6 Videos
- GEOMETRICAL OPTICSBook:ALLENChapter:GEOMETRICAL OPTICSExercise:subjective
Explore 14 Videos
Similar Questions
A battery of 20 cells is charged by 220 V with a charging current of 15 A . If the emf of each cell is 2 V and internal resistance is 0.1Ω , then the series resistance required to be placed in the circuit will be
View SolutionIn the situation shown each cell has e.m.f. 4V and internal resistance 1Ω. The maximum power that can be delivered to the variable load resistance R is

View SolutionTwo electric bulbs rated 50 W and 100 V are glowing at full power, when used in parallel with a battery of emf 120 V and internal resistance 10 Ω. The maximum number of bulbs that can be connected in the circuit when glowing at full power, is
View SolutionThe potential difference between the terminals of a battery of emf 6.0V and internal resistance1(Ω)drops to 5.8 V when connected across an external resistor. Find the resistance of the external resistor.
View SolutionAn element with emfε and interval resistance r is connected across an external resistance R. The maximum power in external circuit is 9W. The current flowing through the circuit in these conditions is 3A. Then which of the following is // are correct ?
View SolutionIn a potentiometer circuit, the emf of driver cell is 2V and internal resistance is 0.5Ω. The potentiometer wire is 1m long . It is found that a cell of emf 1V and internal resistance 0.5Ω is balanced against 60 cm length of the wire. Calculate the resistance of potentiometer wire.
View Solutionin the circuit shown in the figure, each battery is of 5 V and has an internal resistance of 0.2 Omega What is the reading of the ideal voltmeter V
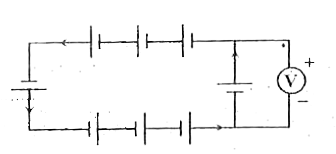
View SolutionTwo identical batteries each of emf E=2 volt and internal resistance r=1 ohm are available t. produce heat in an external resistance by passing a current through it. What is the maximum power that can be developed across an external resistance R using these batteries?
View SolutionThree identical cells, each of emf 2 V and internal resistance 0.2Ω are connected in series to an external resistor of 7.4Ω. Calculate the current in the circuit.
View SolutionA cell of emf 16V and internal resistant 4Ω can deliver maximum power to a load of resistance R where R is equal to
View Solution