A set of n identical resistors, each of resistance RΩ, when connected in series have an effective resistance XΩ and when the resistors are connected in parallel, their effective resistance is YΩ. Find the relation between R, X and Y.
When n identical resistors are connected in series X=R+R+R+..............+R=nR
and r esistors are connected in parallel 1Y=1R+1R+............+1R=1R=nR⇒Y=Rn
⇒X=nR=(RY)R=R2Y∴XY=R2
Similar Questions
Four resistors each of resistance 2 Ω are connected in parallel. What is the effective resistance ?
View SolutionThirteen resistors each of resistance R are connected in the circuit as shown . The effective resistance between A and B is
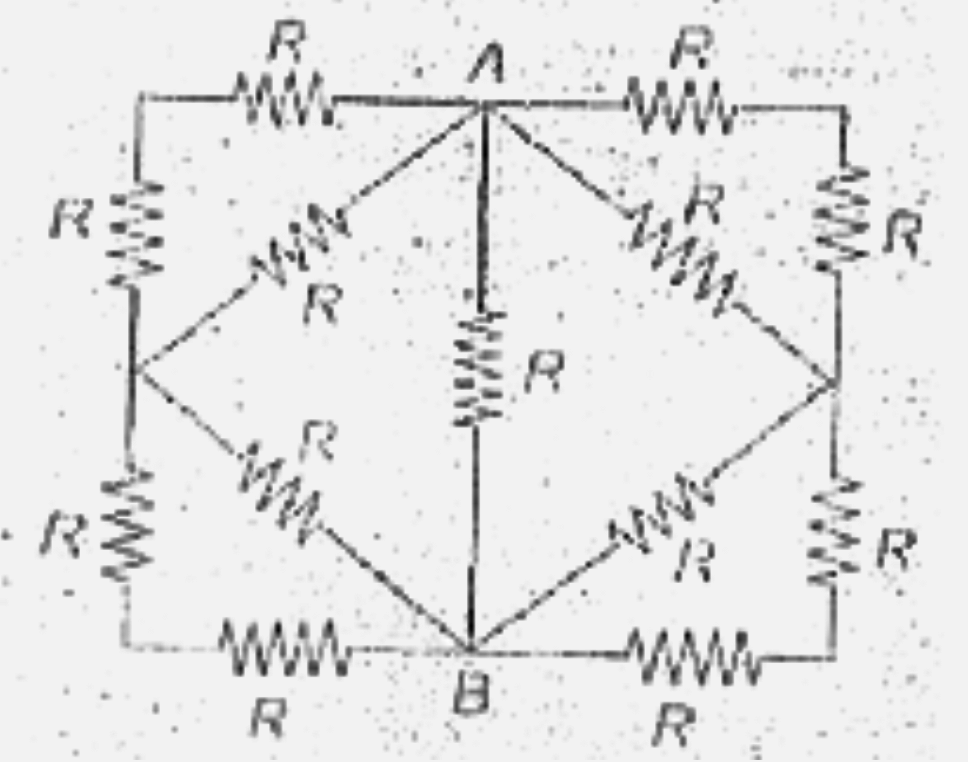
View SolutionTwo resistors having resistance 4 Ω and 6 Ω are connected in parallel. Find their equivalent resistance.
View SolutionIdentical resistance of resistance R are connected as in figure then find out net resistance between x and y.
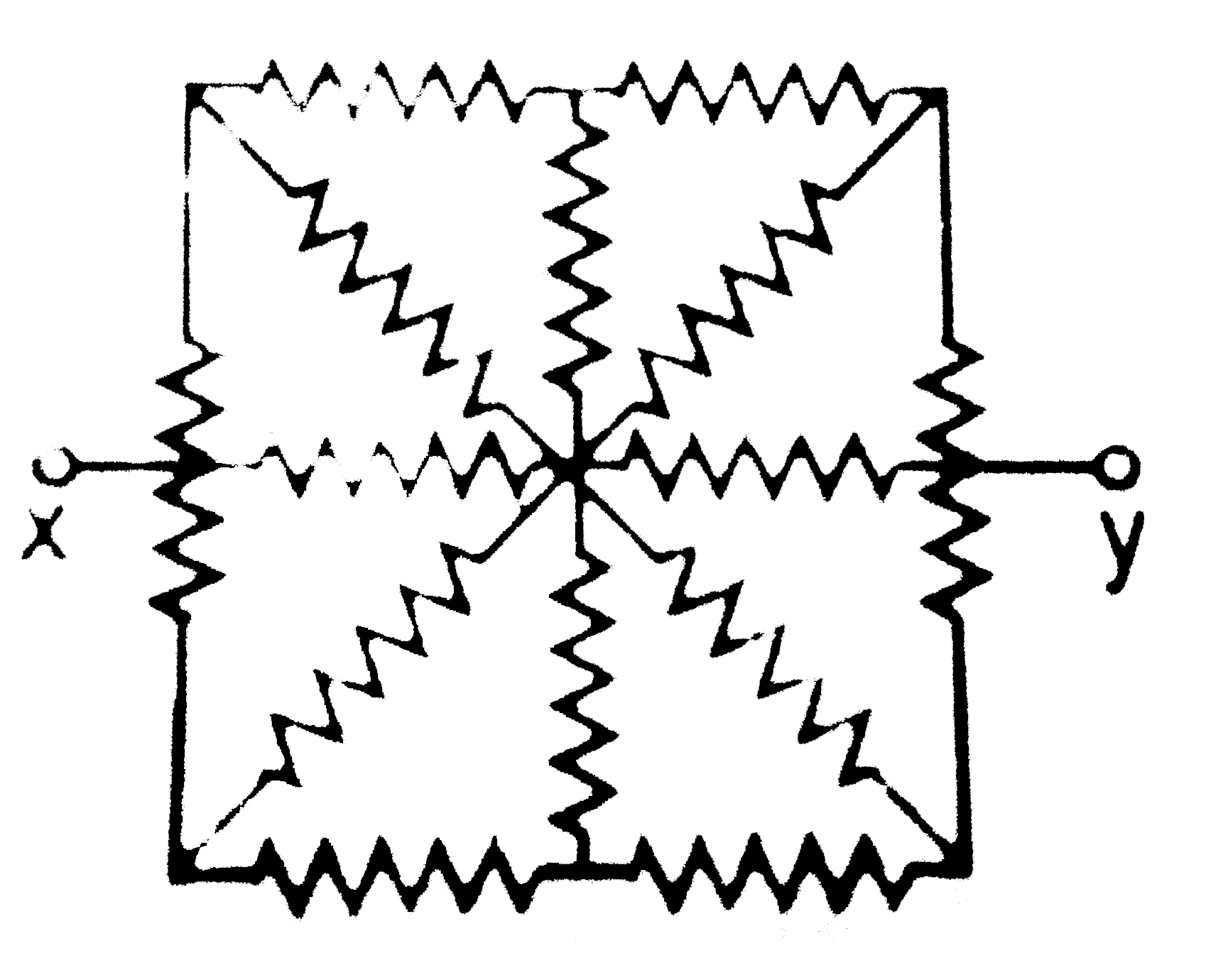
View SolutionTwo resistors of resistances 2Ωand4Ω when connected to a battery will have :
View SolutionThese resistors of 10Ω,15Ωand5Ω are connected in parallel. Find their equivalent resistance.
View SolutionSixteen resistor, each resistane 16Ω are connected in the circuit as shown in fig. the net resistance between A and B is
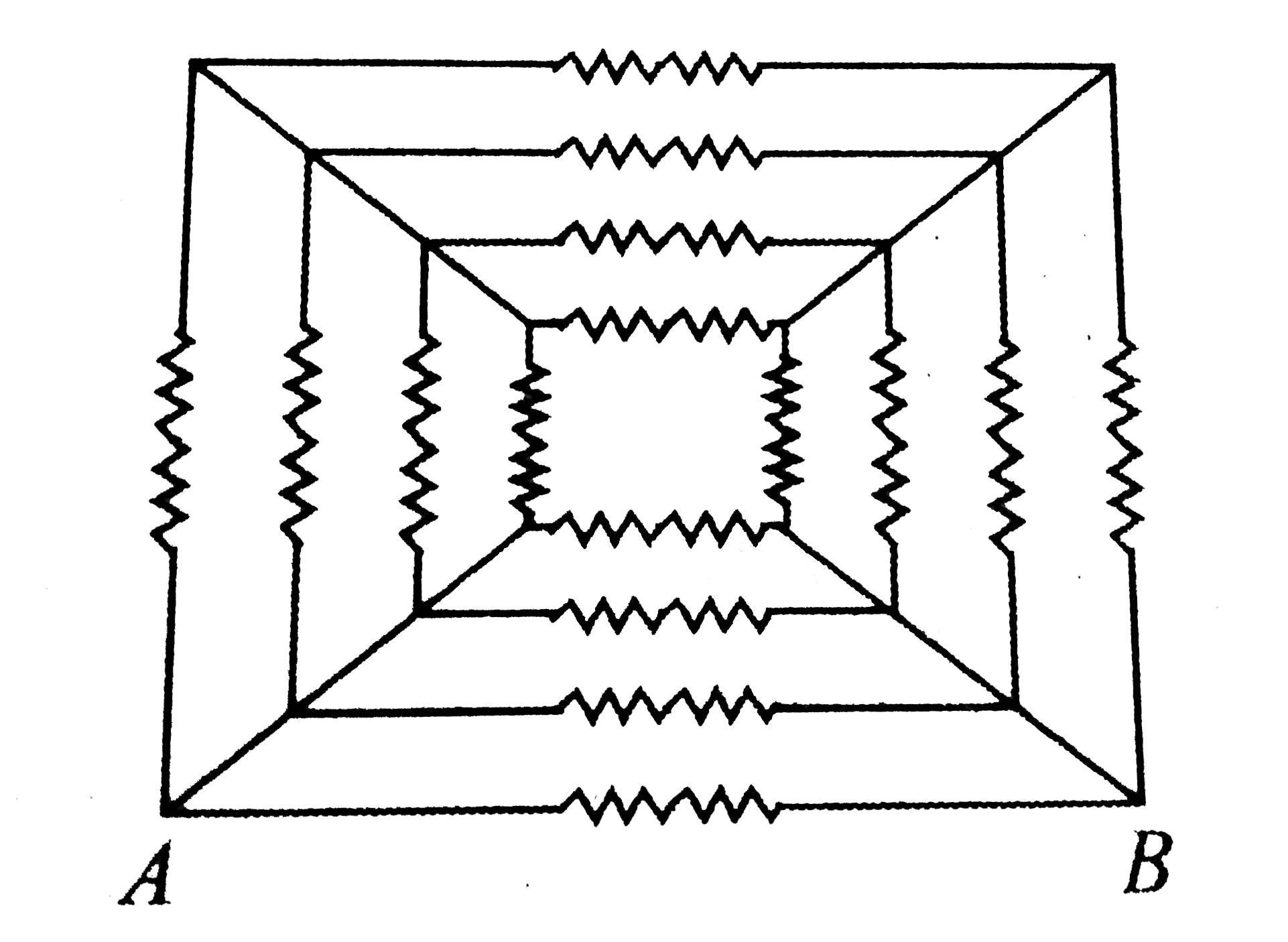
View SolutionFour equal resistors, each of resistance 10Ω, are connected as shown in the circuit diagram. The equivalent resistance between A and B is
View SolutionTwelve resistors each of resistance 1Ω are connected in the circuit shown in figure. Net resistance between point A and H would be
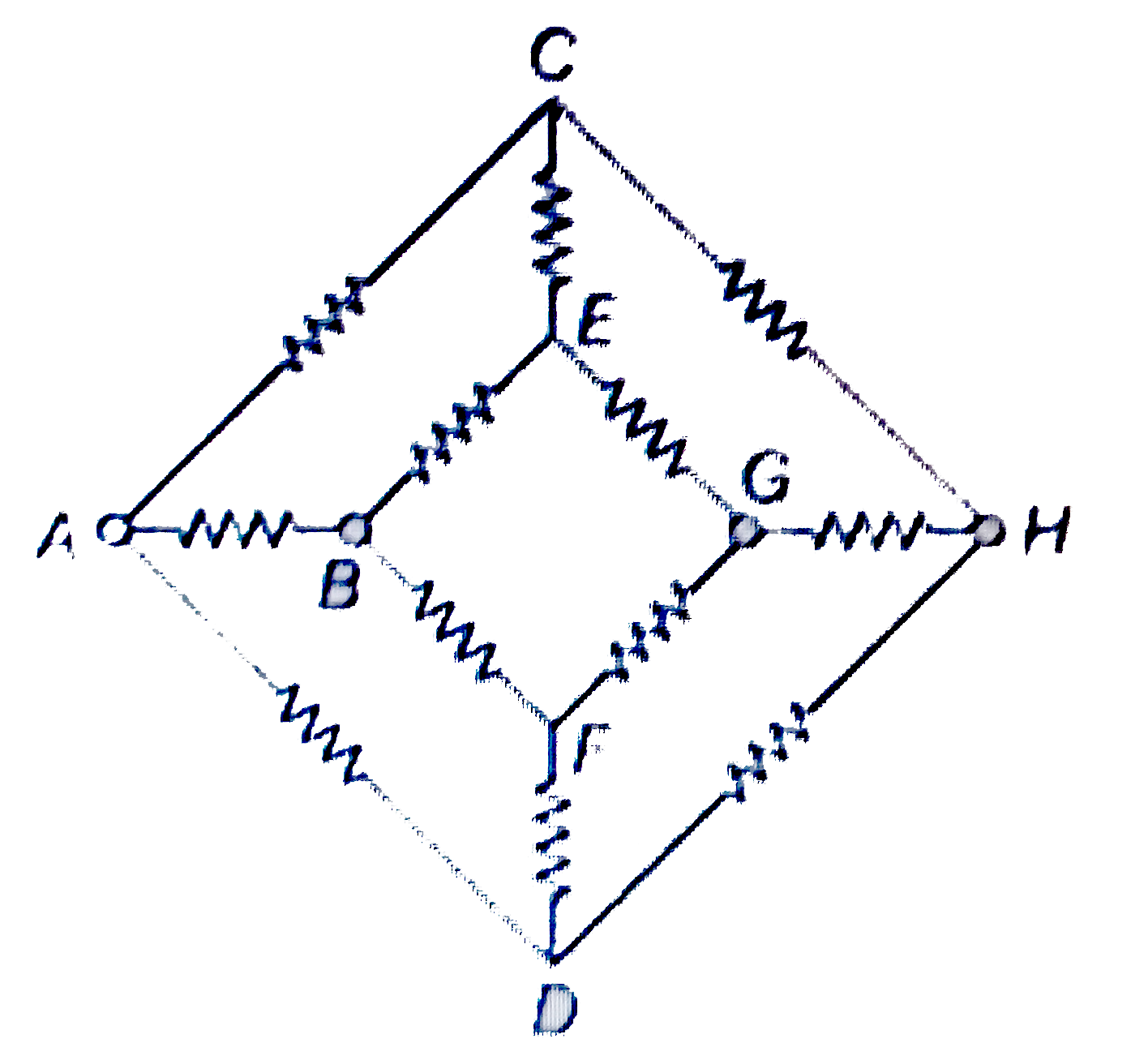
View SolutionWhat resistance must be connected to a 15 Ω resistance to provide an effective resistance of 6Ω ?
View Solution