Two wires of same metal have the same length but their cross-sections are in the ratio 3:1. They are joined in series. The resistance of the thicker wire is 10Ω. The total resistance of the combination will be
The impulse momentum diagram of the ball is dhown in the figure below. Here F, mg, and Deltat represent the average value of the force exerted by the bat, weight of the ball and time interval.
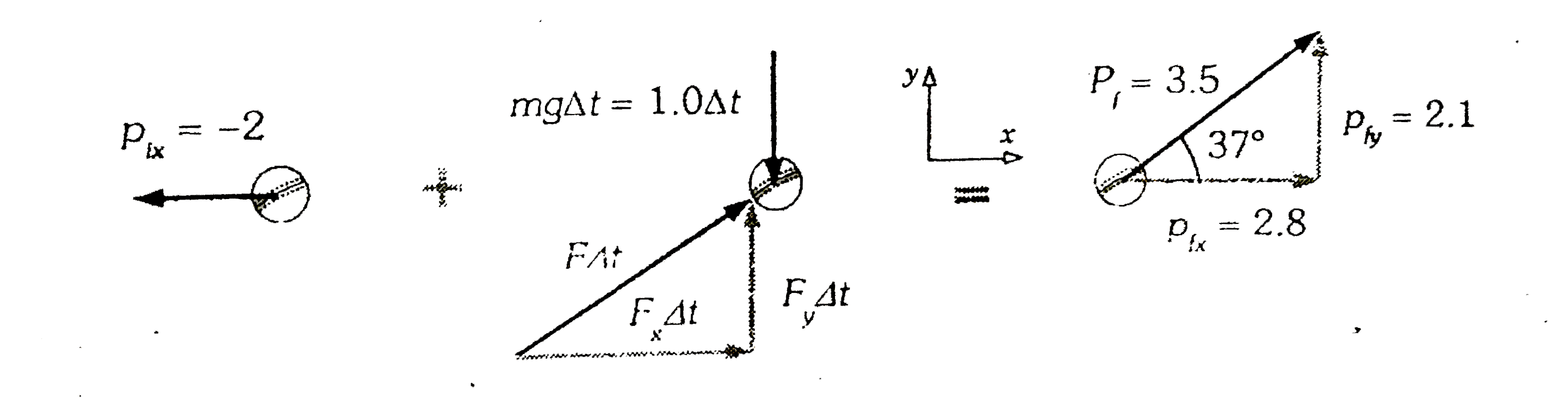
Applying principle of impulse and momentum in x-direction, we have ltbr gt pfx=pix+∑lmp,x→ −2+FxΔt=2.8
Fx=4.8ΔtN...(i)
Applying principle of impulse and momentum in y-direction, we have
pfy=piy+∑Imp.y→ 0.0+FyΔt−1.0Δt=2.1
Fy=(2.1Δt+1.0)N.....(ii)
Applying principle of impulse and momentum in y-direction, we have
(a) Substituting Δt=0.30s, on equations (i) and (ii), we find →F=16ˆi+8ˆjN
(b) Substituting Δt=0.03s, in equations (i) and (ii), we find →F=160ˆi+71ˆjN
Substituting Δt=0.003s, in equations 1 and 2, we find →F=1600ˆi+701ˆjN
(d) It is clear from the above results that as the duration of contact between the ball and the bat decreases, effect of the weight of the ball also decreases as compared with that of the force of the bat and for sufficiently short time interval, it can be neglected.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-III
26 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-IV A
32 Videos
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
Similar Questions
Two wire of the same meta have same length, but their cross-sections are in the rati 3:1 . They are joined in series. The resistance of thicker wire is 10Ω . The total resistance of the combination will be
View SolutionTwo metallic wires of the same material B, have the same length out cross-sectional area is in the ratio 1:2. They are connected (i) in series and (ii) in parallel. Compare the drift velocities of electrons in the two wires in both the cases (i) and (ii) .
View SolutionMasses of 3 wires of same metal are in the ratio 1 : 2 : 3 and their lengths are in the ratio 3 : 2 : 1. The electrical resistances are in ratio
View SolutionTwo wires of the same material and same mass are stretched by the same force. Their lengths are in the ratio 2:3. Their elongation are in the ratio
View SolutionTwo wires of the same materical having equal area of cross-section have L and 2L. Their respective resistances are in the ratio
View SolutionTwo wires of the same dimensions but resistivities ρ1 and ρ2 are connected in series. The equivalent resistivity of the combination is
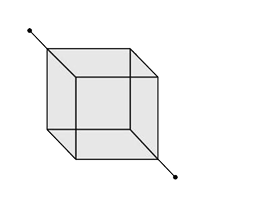
View SolutionTwelve wires of equal length and same cross-section are connected in the form of a cube. If the resistance of each of the wires is R, and the effective resistance between the two diagonal ends is Re, then the ratio RRe is equal to __.
View SolutionWhen a resistance wire is passed through a die the cross-section area decreases by 1%, the change in resistance of the wire is
View SolutionTwo wires of the same material and same length have radii 1 mm and 2 mm respectively. Compare :
their resistances,
View SolutionTwo steel wires of the same radius have their lengths in the ratio of 1:2. If they are stretched by the same force, then the strains produced in the two wires will be in the ratio of
View Solution
