Find the total linear momentum of the electrons in a conductor of length l=1000m carrying a current I=70A.
To find mass center of truncated bodies we can make use of superposition principle that is. If we add the removed portion in the same place we obtain the original body. The idea is illustrated in the following figure.

The removed portion is added to the trucated body keeping their location unchanged relative to the coordinate frame.
Denoting massses of the truncated body, removed portion and the original body by mtb,mrp and mob and location of their mass centres by xtb,xrp and xob, we can write mtbxtb+mrbxrp=mobxob
From the above equation we obtain position coordinate xtb of the mass center of the truncated body.
xtb=mobxob−mrbxrpmtb
Denoting mass per unit area by sigma, we can express the masses mtb,mrp and mob.
Mass of truncated body mtb=σ{π(r2−r24)}=3σπr24
Mass of the removed portion mrp=σπr24
Mass of the original body mob=σπr2
Mass center of the truncated body xtb
Mass center of the removed portion xrp=r2
Mass center of the original body xob=0
Substituting the above values in equation (1), we obtain the mass the center of the truncated body.
xtb=mobxob−mrbxrpmtb=(σπr2)×0−(σπr24)(r2)3σπr24=−r6
Mass center of the truncated body is at point (−r6,0)
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-III
26 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-IV A
32 Videos
- CIRCULAR MOTIONBOOK - ALLENCHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE (J-A)
6 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
Similar Questions
Find the total linear momentum of the electrons in a conductor of length I=1000m carrying a current I = 70A.
View SolutionFind the magnetic field B at the centre of a rectangular loop of length l and width b, carrying a current i.
View SolutionA Long straight conductor carrying a current of 2 A is in parallel to another conductor of length 5 cm. and carrying a current 3A. They are separated by a distance of 10 cm. Calculate (a) B due to first conductor at second conductor (b) the force on the short conductor.
View SolutionFind the magnetic induction due to a straight conductor of length 16cm carrying current of 5A at a distance of 6cm from the midpoint of conductor
View SolutionState Ohm' law and deduce it from the knowledge of drift velocity of free electrons in a conductor carrying current.
View SolutionThe magnetic field at centre of a hexagonal coil of side l carrying a current I is

View SolutionCalculate the uncertainty in the momentum of an electron if it is confined to a linear region of length 1×10−10metre
View SolutionCalculate the uncertainty in the momentum of an electron if it is confined to a linear region of length 1x 10^-8
View SolutionA potential difference of 30V is applied between the ends of a conductor of length 100 m and resistance 0.5Ω . The conductor has a uniform cross-section. Find the total linear momentum of free electrons.
View SolutionTwo long parallel conductors are carrying currents in the same direction as shown in the figure. The upper conductor A carrying a current of 100A is held firmly in position. The lower conductor B carries a current of 50A and free to move up and down. The linear mass density of the lower conductor is 0.01 kg/m.
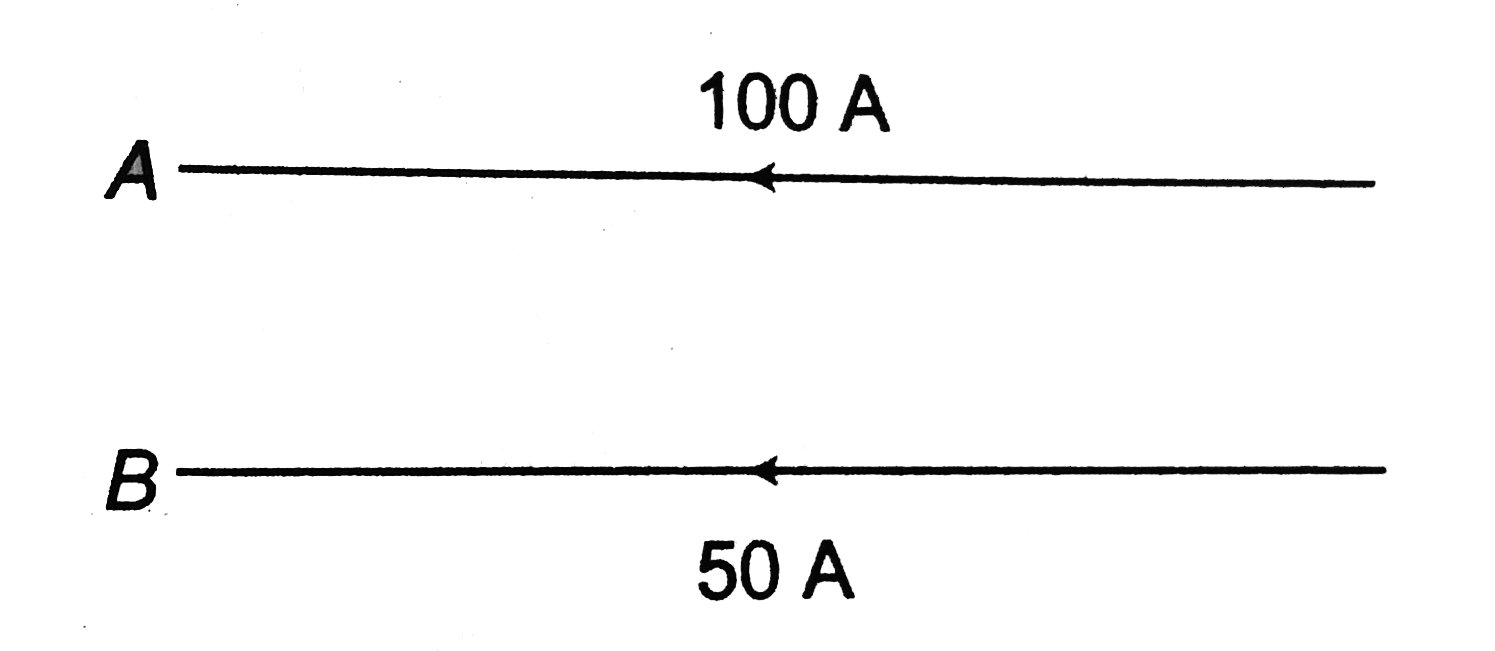
View Solution
