In a reference frame a man A is moving with velocity (3ˆi−4ˆj)ms−1 and another man B is moving with velocity (ˆi+ˆj)ms−1 relative to A. Find the actual velocity of B.
To find the actual velocity of man B, we can follow these steps:
Step 1: Understand the given information
We have:
- Velocity of man A, →VA=3^i−4^j m/s
- Velocity of man B relative to A, →VB/A=^i+^j m/s
Step 2: Use the relative velocity formula
The relative velocity of B with respect to A can be expressed as:
→VB/A=→VB−→VA
where:
- →VB is the actual velocity of B,
- →VA is the velocity of A.
Step 3: Rearrange the equation to find →VB
From the relative velocity equation, we can rearrange it to solve for →VB:
→VB=→VB/A+→VA
Step 4: Substitute the known values
Now, substitute the values of →VB/A and →VA:
→VB=(^i+^j)+(3^i−4^j)
Step 5: Combine the vectors
Now, combine the components:
→VB=(1+3)^i+(1−4)^j
→VB=4^i−3^j
Step 6: Write the final answer
Thus, the actual velocity of man B is:
→VB=4^i−3^j m/s
Summary of the solution:
The actual velocity of man B is 4^i−3^j m/s.
---
We have:
- Velocity of man A, →VA=3^i−4^j m/s
- Velocity of man B relative to A, →VB/A=^i+^j m/s
The relative velocity of B with respect to A can be expressed as:
→VB/A=→VB−→VA
where:
- →VB is the actual velocity of B,
- →VA is the velocity of A.
From the relative velocity equation, we can rearrange it to solve for →VB:
→VB=→VB/A+→VA
Now, substitute the values of →VB/A and →VA:
→VB=(^i+^j)+(3^i−4^j)
Now, combine the components:
→VB=(1+3)^i+(1−4)^j
→VB=4^i−3^j
Thus, the actual velocity of man B is:
→VB=4^i−3^j m/s
The actual velocity of man B is 4^i−3^j m/s.
Topper's Solved these Questions
- MOTION IN A PALNEBook:ALLENChapter:MOTION IN A PALNEExercise:EXERCISE-02
Explore 37 Videos
- MOTION IN A PALNEBook:ALLENChapter:MOTION IN A PALNEExercise:EXERCISE-03
Explore 6 Videos
- KINEMATICS-2DBook:ALLENChapter:KINEMATICS-2DExercise:Exercise (O-2)
Explore 46 Videos
- NEWTON'S LAWS OF MOTION & FRICTIONBook:ALLENChapter:NEWTON'S LAWS OF MOTION & FRICTIONExercise:EXERCISE (JA)
Explore 4 Videos
Similar Questions
A particle of mass m moving with a velocity (3ˆi+2ˆj)ms−1 collides with another body of mass M and finally moves with velocity (−2ˆi+ˆj)ms−1. Then during the collision
View SolutionA particle has an initial velocity (6ˆi+8ˆj)ms−1 and an acceleration of (0.8ˆi+0.6ˆj)ms−2. Its speed after 10s is
View SolutionA man A moves due to East with velocity 6ms−1 and another man B moves in N−30∘E with 6ms−1 . Find the velocity of B w.r.t. A.
View SolutionA small sphere of mass m=1kg is moving with a velocity (4ˆi−ˆj)m/s. it hits a fixed smooth wall and rebounds with velocity (ˆi+3ˆj)m/s. The coefficient of restitution between the sphere and the wall is n/16. Find the value of n.
View SolutionA body of mass 0.8 kg has intial velocity (3ˆi−4ˆj)ms−1 and final velocity (−6ˆj+2ˆk)ms−1. Find change in kinetic energy of the body?
View SolutionConsider a horizontal surface moving vertically upward with velocity 2ms−1. A small ball of mass 2 kg is moving with velocity (2ˆi−2ˆj)ms−1 If the coefficient of restitution and coefficient of friction are 12and13 respectively, find the horizontal velocity (in ms−1) of the ball after the collision.
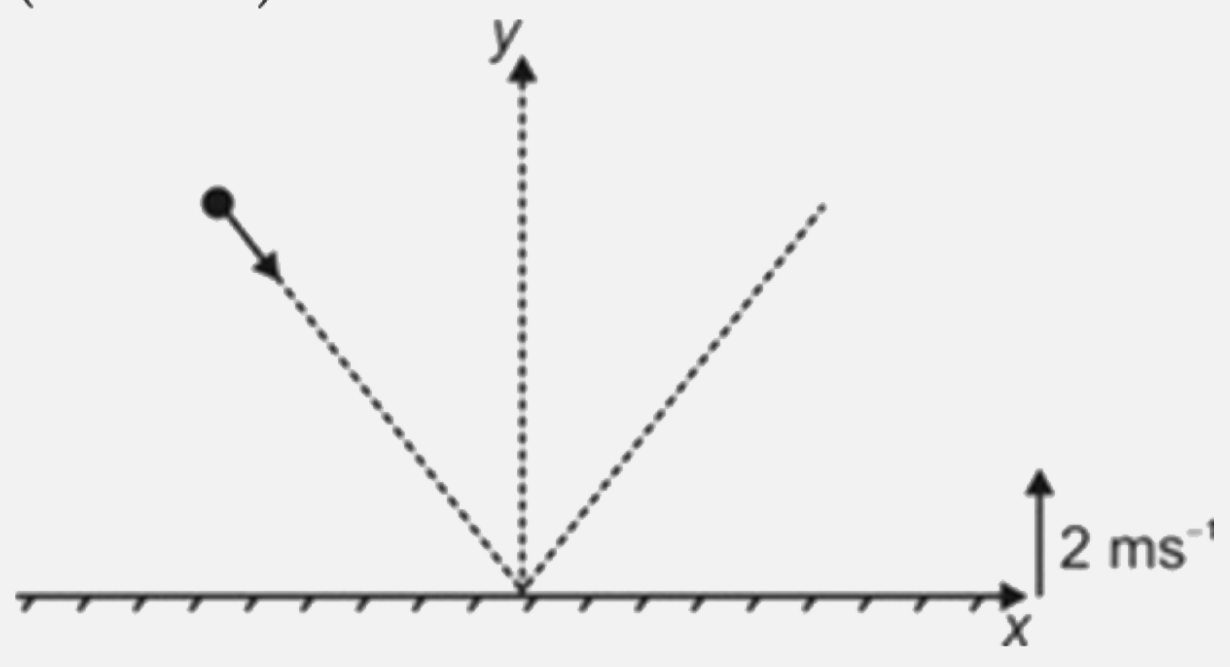
View SolutionA body of mass 3 kg moving with a velocity (2ˆi+3ˆj+3ˆk) m/s collides with another body of mass 4 kg moving with a velocity (3ˆi+2ˆj−3ˆk) m/s. The two bodies stick together after collision. The velocity of the composite body is
View SolutionA small sphere of mass 1kg is moving with a velocity (6ˆi+ˆj)m/s . It hits a fixed smooth wall and rebounds with velocity (4ˆi+ˆj)m/s. The coefficient of restitution between the sphere and the wall is
View SolutionA body of mass 5 kg is moving with velocity of v=(2ˆi+6ˆj)ms−1 at t=0s. After time t=2s, velocity of body is (10ˆi+6ˆj), then change in momentum to body is
View SolutionA body of mass 5 kg is moving with velocity of v=(2ˆi+6ˆj)ms−1 at t=0s. After time t=2s, velocity of body is (10ˆi+6ˆj), then change in momentum to body is
View Solution