Question

A projectile is fired horizontally with velocity of 98 m/s from the top of a hill 490 m high. Find
(a) the time taken by the projectile to reach the ground,
(b) the distance of the point where the particle hits the ground from foot of the hill and
(c) the velocity with which the projectile hits the ground. $(g=9.8m/s^2)$
.

Explanation

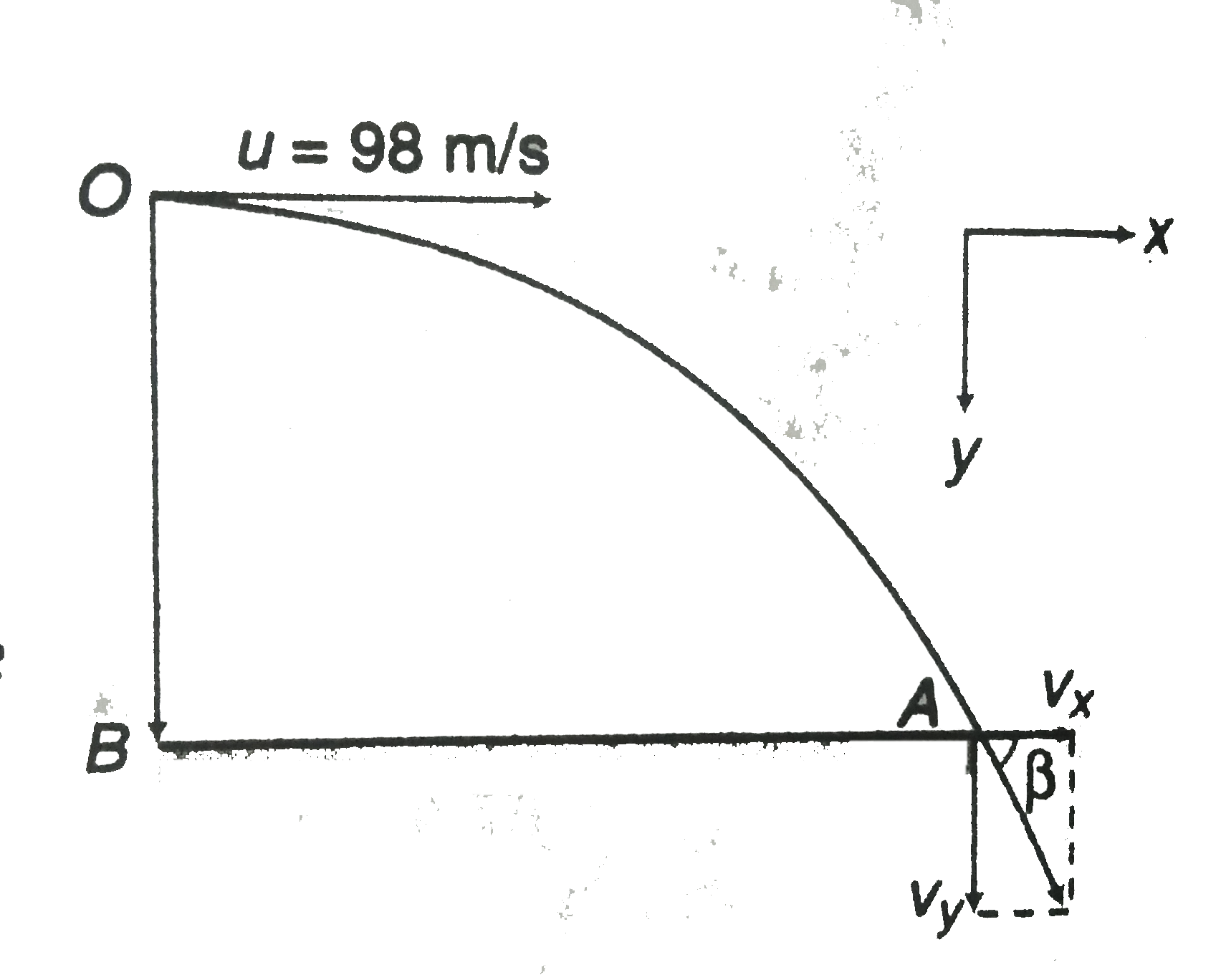
(i) The projectile is fired from the top O of a hill with velocity `u=98ms^(1)` along the horizontal OX. It reaches the target P in vertical distance,
OA=y=490m
As `y=(1)/(2)"gt"^(2)`
`therefore 490=(1)/(2)xx9.8t^(2)`
or `t=sqrt(100)=10s`.
(ii) Distance of the target from the hill is
AP=x=horizontal velocity xxtime=98xx10=980m.
(iii) The horizontal components of velocity v of the projectile at point P is `v_(x)=u=98ms^(-1)`
`v_(x)=u_(y)+gt=0+9.8xx10=98ms^(-1)` and vertical component
`therefore v=sqrt(v_(x)^(2)+v_(y)^(2))=sqrt(98^(2)+98^(2))=98sqrt(2)=138.59ms^(-1)`
Now if the resultant velocity v makes angle `beta` with the horizontal, then
`tan beta=(v_(y))/(v_(x))=(98)/(98)=1` or `beta=45^(@)`