A ray reflected successively from two plane mirrors inclined at a certain angle (<90∘) undergoes a deviation of 300∘ . The number of images observable are:
10
11
12
14
The correct Answer is:B
To solve the problem of finding the number of images formed by two plane mirrors inclined at an angle, we can follow these steps:
1. Understanding the Problem: We have two plane mirrors inclined at an angle θ (which is less than 90∘). A ray of light reflects off these mirrors and undergoes a total deviation of 300∘.
2. Using the Deviation Formula: The formula for the total deviation O when a ray is reflected from two mirrors is given by:
O=360∘−2θ
Here, O is the total deviation, and θ is the angle between the two mirrors.
3. Setting Up the Equation: We know from the problem that the total deviation O is 300∘. Therefore, we can set up the equation:
300∘=360∘−2θ
4. Solving for θ: Rearranging the equation to solve for θ:
2θ=360∘−300∘
2θ=60∘
θ=30∘
5. Calculating the Number of Images: The number of images n formed by two mirrors inclined at an angle θ can be calculated using the formula:
n=360∘θ−1
Substituting θ=30∘:
n=360∘30∘−1
n=12−1
n=11
6. Conclusion: The number of images observable is 11.
Final Answer:
The number of images observable is 11.
O=360∘−2θ
Here, O is the total deviation, and θ is the angle between the two mirrors.
300∘=360∘−2θ
2θ=360∘−300∘
2θ=60∘
θ=30∘
n=360∘θ−1
Substituting θ=30∘:
n=360∘30∘−1
n=12−1
n=11
The number of images observable is 11.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE-3
12 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE-04[A]
23 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE- 01
45 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
Image formed by reflection from a plane mirror is :
View SolutionTwo plane mirrors at an angle such that a ray incident on a mirror undergoes a total deviation of 240∘ after two reflections:
View SolutionAn object is placed asymmetrically between two plane mirrors inclined at an angle of 72∘. The number of images formed is
View SolutionTwo plane mirror are inclined at an angle of 72∘. The number of images of a point object placed between them will be
View SolutionThe total number of images formed by two plane mirrors , inclined at an angle of 15∘ and an objectvitying symmetrically is
View SolutionAn object is placed (i) asymmetrically (ii) symmetrically, between two plane mirrors inclined at an angle of 50∘. Find the number of images formed.
View SolutionAn object is placed between two plane mirrors inclined at an angle to each other. If the number of images formed is 7 then the angle of inclination is -
View SolutionThe total number of image formed by two plane mirrors, inclined at an angle of 112.5∘ and an object lying symmetrically is
View SolutionTwo plane mirrors A and B are aligned parallel to each other as shown in the figure. A light ray is incident at an angle of 30∘ at a point just inside one end of A. The number of times the ray undergoes reflections (including the first one) before it emerges out is
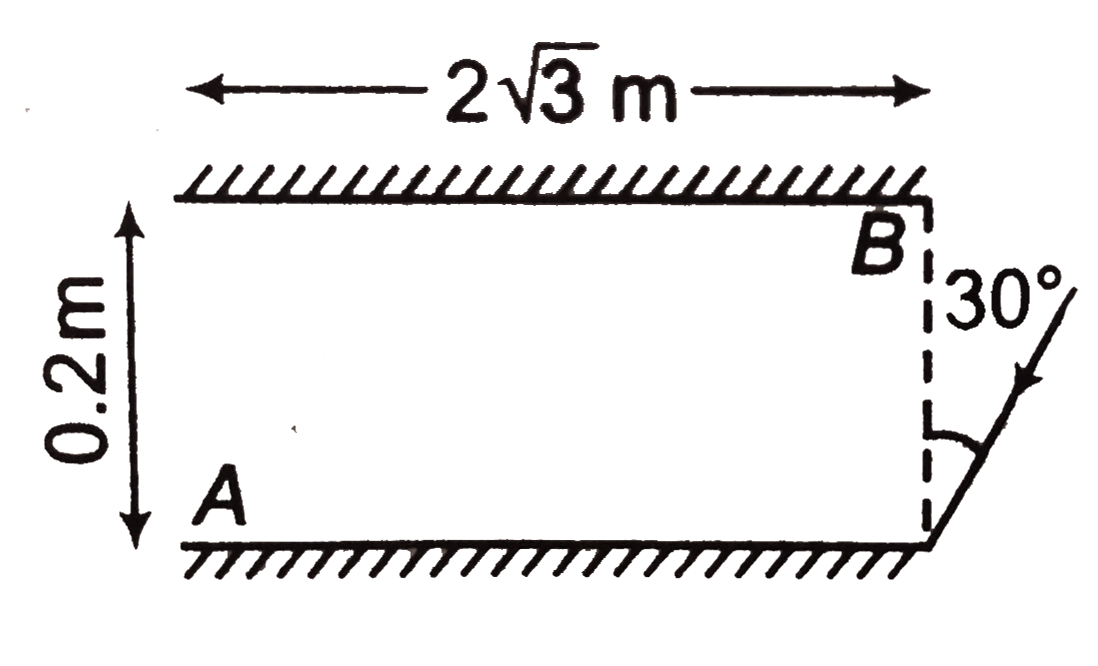
View SolutionTwo plane mirror are inclined to each other at 90∘. A ray of light is incident on one mirror. The ray will undergo a total deviation of
View Solution
