The coefficient of linear expansion 'α' of a rod of length 2 m varies with the distance x from the end of the rod as α=α0+α1x where α0=1.76×10−5^(@)C^(-1)and alpha_(1) = 1.2 xx 10^(-6)m^(-1) ∘C−1. The increase in the length of the rod. When heated through 100∘C is :-
2cm
3.76mm
1.2mm
None of these
The correct Answer is:B
To solve the problem of finding the increase in the length of a rod when heated, we will follow these steps:
Step 1: Understand the Coefficient of Linear Expansion
The coefficient of linear expansion α varies with the distance x from the end of the rod as:
α=α0+α1x
where:
- α0=1.76×10−5∘C−1
- α1=1.2×10−6m−1∘C−1
Step 2: Set Up the Expression for Length Change
The increase in length dL of an infinitesimal element dx of the rod when the temperature changes by ΔT is given by:
dL=α⋅dx⋅ΔT
Substituting the expression for α:
dL=(α0+α1x)⋅dx⋅ΔT
Step 3: Integrate Over the Length of the Rod
To find the total increase in length L when the entire rod is heated, we need to integrate from x=0 to x=L:
ΔL=∫L0(α0+α1x)dx⋅ΔT
where L=2m and ΔT=100∘C.
Step 4: Calculate the Integral
The integral can be split into two parts:
ΔL=ΔT(∫L0α0dx+∫L0α1xdx)
Calculating the first integral:
∫L0α0dx=α0⋅L
Calculating the second integral:
∫L0α1xdx=α1⋅L22
Thus, we have:
ΔL=ΔT(α0L+α1L22)
Step 5: Substitute the Values
Substituting the known values:
ΔL=100(1.76×10−5⋅2+1.2×10−6⋅222)
Calculating each term:
1. 1.76×10−5⋅2=3.52×10−5
2. 1.2×10−6⋅42=2.4×10−6
Now, adding these:
ΔL=100(3.52×10−5+2.4×10−6)
Calculating the sum:
3.52×10−5+2.4×10−6=3.76×10−5
Step 6: Final Calculation
Now, multiplying by 100:
ΔL=100⋅3.76×10−5=3.76×10−3m
Converting to millimeters:
ΔL=3.76mm
Conclusion
The increase in the length of the rod when heated through 100∘C is 3.76mm.
---
The coefficient of linear expansion α varies with the distance x from the end of the rod as:
α=α0+α1x
where:
- α0=1.76×10−5∘C−1
- α1=1.2×10−6m−1∘C−1
The increase in length dL of an infinitesimal element dx of the rod when the temperature changes by ΔT is given by:
dL=α⋅dx⋅ΔT
Substituting the expression for α:
dL=(α0+α1x)⋅dx⋅ΔT
To find the total increase in length L when the entire rod is heated, we need to integrate from x=0 to x=L:
ΔL=∫L0(α0+α1x)dx⋅ΔT
where L=2m and ΔT=100∘C.
The integral can be split into two parts:
ΔL=ΔT(∫L0α0dx+∫L0α1xdx)
Calculating the first integral:
∫L0α0dx=α0⋅L
Calculating the second integral:
∫L0α1xdx=α1⋅L22
Thus, we have:
ΔL=ΔT(α0L+α1L22)
Substituting the known values:
ΔL=100(1.76×10−5⋅2+1.2×10−6⋅222)
Calculating each term:
1. 1.76×10−5⋅2=3.52×10−5
2. 1.2×10−6⋅42=2.4×10−6
ΔL=100(3.52×10−5+2.4×10−6)
Calculating the sum:
3.52×10−5+2.4×10−6=3.76×10−5
Now, multiplying by 100:
ΔL=100⋅3.76×10−5=3.76×10−3m
Converting to millimeters:
ΔL=3.76mm
The increase in the length of the rod when heated through 100∘C is 3.76mm.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE -02
82 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE -03
10 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - SOME WORKED OUT EXAMPLES
83 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
What should be the length of steel and copper rods at 0∘C that the length of steel rod is 5 cm longer than copper at all termperature? Given αCu=1.7×105.∘C−1 and αsteel=1.1×105.∘C−1.
View SolutionThe coefficient of linear expansion 'α' of the material of a rod of length l0 varies with absolute temperature as α=aT−bT2 where a & b are constant. The linear expansion of the rod when heated from T1 to T2=2T1 is :-
View SolutionWhat must be the lengths of steel and copper rods at 0∘C for the difference in their lengths to be 10 cm at any common temperature?
(αsteel=1.2×10−5.∘K−1andαcopper=1.8×10−5.∘K−1)
View SolutionWhen a strip made or iron (α1) and copper (α2),(α2>α1) is heated
View SolutionA rod has variable co-efficient of linear expansion α=x5000. If length of the rod is 1 m . Determine increase in length of the rod in (cm) on increasing temperature of the rod by 100∘C.
_S01_121_Q01.png)
View SolutionA copper rod of 88 cm and an aluminium rod of unknown length have their increase in length independent of increase in temperature. The length of aluminium rod is (αcu=1.7×10−5K−1 and αAl=2.2×10−5K−1)
View SolutionFind aC and α of the smooth rod of mass m and length l.
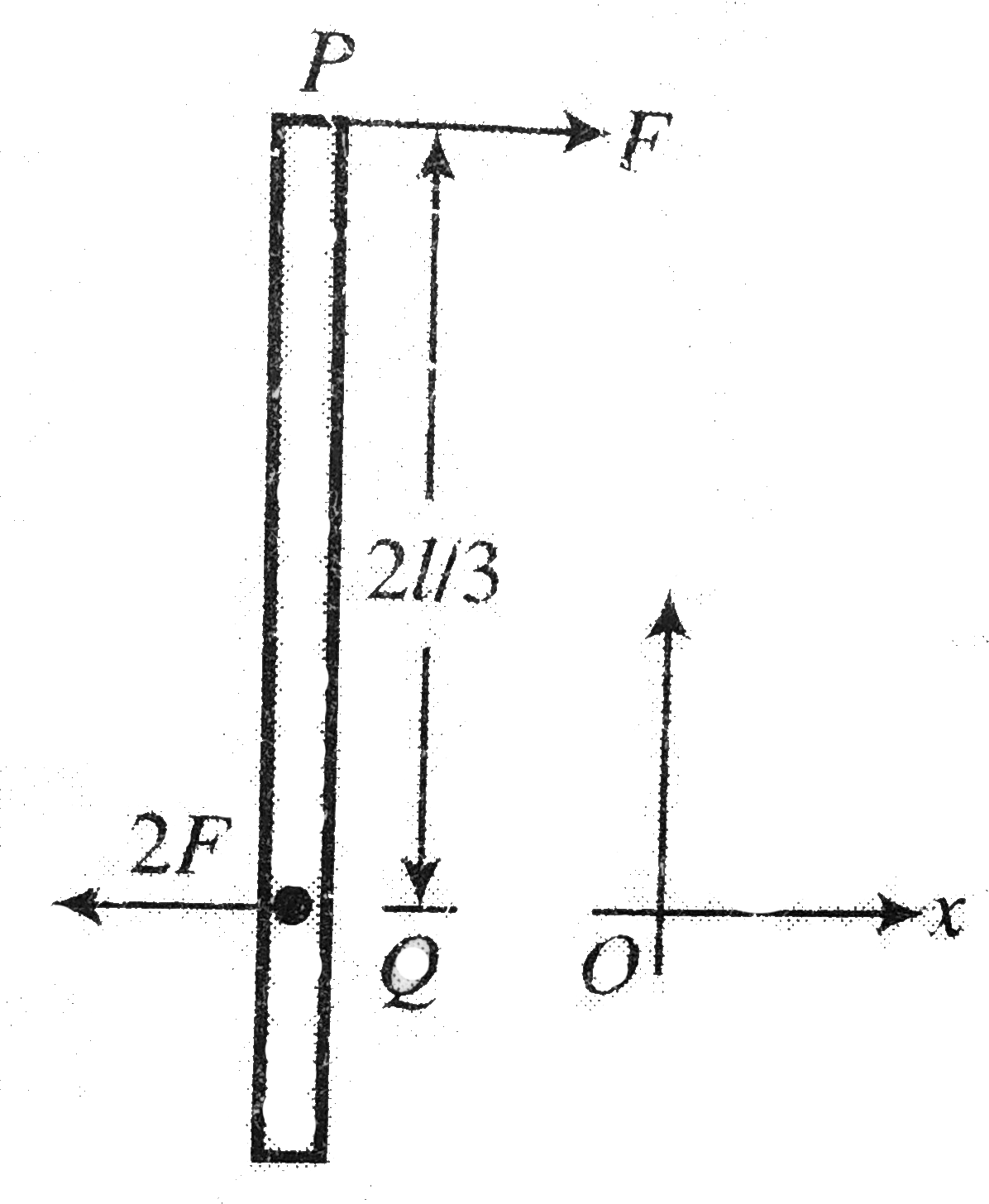
View SolutionThe design of some physical instrument requires that there be a constant difference in length of 10 cm between an iron rod and a copper cylinder laid side by side at all temperature find their lengths
(αFe=11×106.∘C−1,αCu=17×10−6.∘C−1)
View SolutionA rod of length 2m rests on smooth horizontal floor. If the rod is heated from 0∘C to 20∘C. Find the longitudinal strain developed ? (α=5×10−5/.∘C)
View SolutionThe coefficient of linear expansion of crystal in one direction is α1 and that in every direction perpendicular to it is α2. The coefficient of cubical expansion is
View Solution
