The temperatures T1andT(2) of two heat reservoirs in an ideal carnot engine are 1500∘Cand500∘C. Which of these (a) increasing T1by100∘C or (b) decreasing T2by100∘C would result in greater improvement of the efficiency of the engine?
To solve the problem, we need to calculate the efficiency of the Carnot engine in two scenarios: (a) when we increase T1 by 100∘C and (b) when we decrease T2 by 100∘C. The efficiency of a Carnot engine is given by the formula:
η=1−T2T1
where T1 and T2 are the absolute temperatures of the hot and cold reservoirs, respectively, measured in Kelvin.
Step 1: Convert temperatures from Celsius to Kelvin
1. Convert T1 and T2 to Kelvin:
- T1=1500∘C=1500+273=1773K
- T2=500∘C=500+273=773K
Step 2: Calculate initial efficiency
2. Calculate the initial efficiency η:
η=1−T2T1=1−7731773
- Calculate 7731773:
7731773≈0.436
- Therefore, the initial efficiency is:
η≈1−0.436=0.564or56.4%
Step 3: Case (a) - Increase T1 by 100∘C
3. Calculate new T1:
- New T1 after increasing by 100∘C:
T′1=1500+100=1600∘C=1600+273=1873K
4. Calculate new efficiency η′:
η′=1−T2T′1=1−7731873
- Calculate 7731873:
7731873≈0.412
- Therefore, the new efficiency is:
η′≈1−0.412=0.588or58.8%
Step 4: Case (b) - Decrease T2 by 100∘C
5. Calculate new T2:
- New T2 after decreasing by 100∘C:
T′2=500−100=400∘C=400+273=673K
6. Calculate new efficiency η′′:
η′′=1−T′2T1=1−6731773
- Calculate 6731773:
6731773≈0.379
- Therefore, the new efficiency is:
η′′≈1−0.379=0.621or62.1%
Step 5: Compare efficiencies
7. Compare the improvements in efficiency:
- Increase in efficiency from case (a):
Δηa=η′−η=0.588−0.564=0.024or2.4%
- Increase in efficiency from case (b):
Δηb=η′′−η=0.621−0.564=0.057or5.7%
Conclusion
Since Δηb>Δηa, decreasing T2 by 100∘C results in a greater improvement in the efficiency of the engine.
Final Answer
(b) Decreasing T2 by 100∘C results in a greater improvement of the efficiency of the engine.
---
1. Convert T1 and T2 to Kelvin:
- T1=1500∘C=1500+273=1773K
- T2=500∘C=500+273=773K
2. Calculate the initial efficiency η:
η=1−T2T1=1−7731773
- Calculate 7731773:
7731773≈0.436
- Therefore, the initial efficiency is:
η≈1−0.436=0.564or56.4%
3. Calculate new T1:
- New T1 after increasing by 100∘C:
T′1=1500+100=1600∘C=1600+273=1873K
4. Calculate new efficiency η′:
η′=1−T2T′1=1−7731873
- Calculate 7731873:
7731873≈0.412
- Therefore, the new efficiency is:
η′≈1−0.412=0.588or58.8%
5. Calculate new T2:
- New T2 after decreasing by 100∘C:
T′2=500−100=400∘C=400+273=673K
6. Calculate new efficiency η′′:
η′′=1−T′2T1=1−6731773
- Calculate 6731773:
6731773≈0.379
- Therefore, the new efficiency is:
η′′≈1−0.379=0.621or62.1%
7. Compare the improvements in efficiency:
- Increase in efficiency from case (a):
Δηa=η′−η=0.588−0.564=0.024or2.4%
- Increase in efficiency from case (b):
Δηb=η′′−η=0.621−0.564=0.057or5.7%
Since Δηb>Δηa, decreasing T2 by 100∘C results in a greater improvement in the efficiency of the engine.
(b) Decreasing T2 by 100∘C results in a greater improvement of the efficiency of the engine.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - SOME WORKED OUT EXAMPLES
83 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE -01
65 Videos
- CURRENT ELECTRICITYBOOK - ALLENCHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
An ideal Carnot's engine works between 227∘C and 57∘C. The efficiency of the engine will be
View SolutionIn a Carnot engine when T2=0∘C and T1=200∘C its efficiency is η1 and when T1=0∘C and T2=−200∘C. Its efficiency is η2, then what is η1/η2?
View SolutionThe efficiency of a Carnot engine operating between temperatures of 100∘C and −23∘C will be
View SolutionWhat percentage T1 is of T2 for a 10% efficiency of a heat engine?
View SolutionTwo ideal Carnot engines operate in cascade (all heat given up by one engine is used by the other engine to produce work) between temperatures, T1andT2. The temperature of the hot reservoir of the first engine is T1 and the temperature of the cold reservoir of the second engine is T2. T is temperature of the sink of first engine which is also the source for the second engine. How is T related to T1andT2, if both the engines perform equal amount of work?
View SolutionCalculate the efficiency of a carnot engine working between the two temperatures: 30∘Cand100∘C
View SolutionA Carnot's engine is made to work between 200∘C and 0∘C first and then between 0∘C and −200∘C. The ratio of efficiencies of the engine in the two cases is
View SolutionA Carnot engine works between 120∘C and 30∘C. Calculate the efficiency. If the power produced by the engine is 400W, calculate the heat abosorbed from the source.
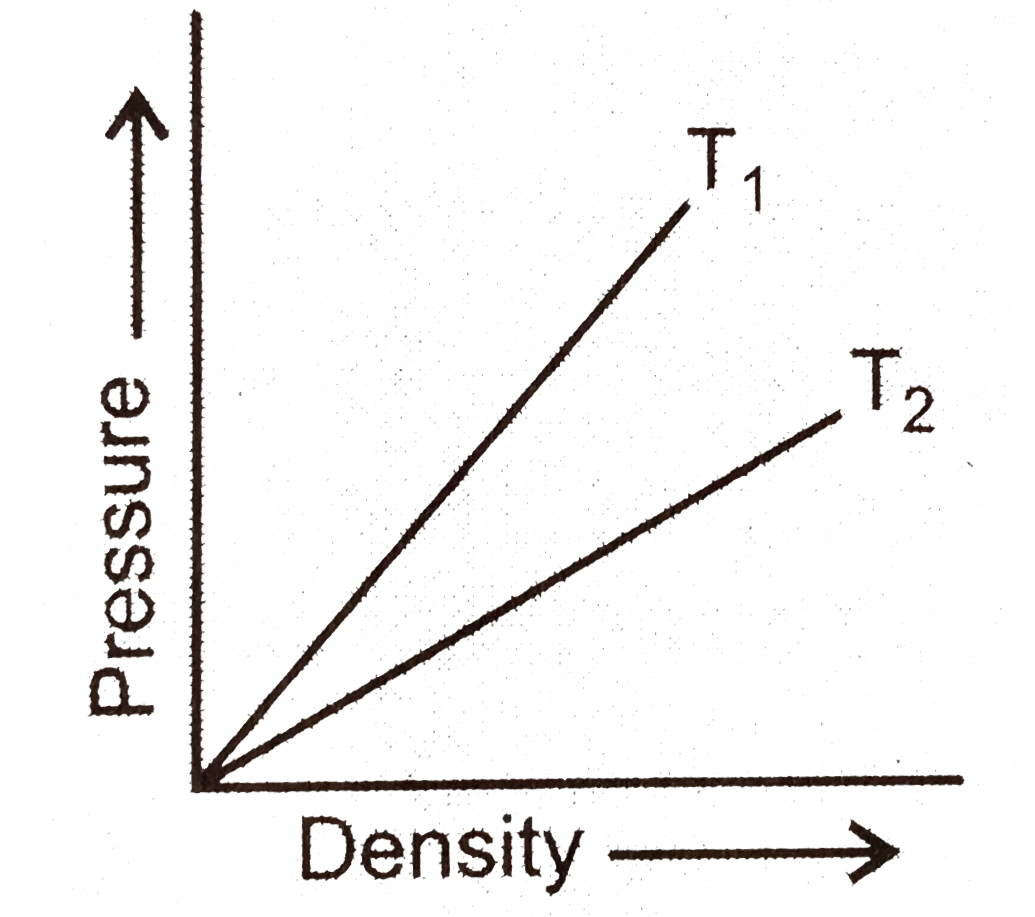
View SolutionFigure shows graphs of pressure versus density for an ideal gas at two temperatures T1 and T2 Which is correct ?
 .
.
View SolutionCarnot engine takes one thousand kilo calories of heat from a reservoir at 827∘C and exhausts it to a sink at 27∘C. How, much work does it perform? What is the efficiency of the engine?
View Solution
