A particle A moves along a circle of radius R=50cm so that its radius vector r relative to the point O(figure) rotates with the constant angular velocity ω=0.40rad/s. Find the modulus of the velocity of the particle, and the modulus and direction of its total acceleration.

v=0.4m/s,a=0.4m/s2
v=0.32m/s,a=0.32m/s2
v=0.32m/s,a=0.4m/s2
v=0.4m/s,a=0.32m/s2
The correct Answer is:D
Angular velocity ω about centre =2ω
=2×0.40=0.30rad/sec
v=ωR
=0.80×12=0.40m/s
a=v2R=0.40×0.40×10050=0.32cm/s2
Topper's Solved these Questions
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-02
57 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-03
6 Videos
- KINEMATICSBOOK - ALLENCHAPTER - KINEMATICSEXERCISE - EXERCISE-2
89 Videos
- ERROR AND MEASUREMENTBOOK - ALLENCHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLENCHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
Knowledge Check
A particle moves along the circumference of a circle of radius r when its completes one rotation.
ADistance travelled is zero and displacement is 2πrBdistance and displacement are zeroCdistance is 2πr but displacement is zeroDdistance and displacement are 2πr each
A particle moves with constant angular velocity in a circle. During the motion its
View SolutionA particle moves in a circle of radius 5 cm with constant speed and time period 0.2πs. The acceleration of the particle is
View SolutionA particle moves along a circle of radius R with a constant angular speed ω . Its displacement (only magnitude) in time t will be
View SolutionA particle moves in a circle with constant angular velocity ω about a point P on its circumference. The angular velocity of the particle about the centre C of the circle is
View SolutionA particle moves in a circle of radius 4 m with a linear velocity of 20ms−1. Find the angular velocity.
View SolutionThe rod of length l=1m rotates with an angular velocity ω=2rads−1 an the point P moves with velocity v=1ms−1 and acceleration a=1ms−2. Find the velocity and acceleration of Q.
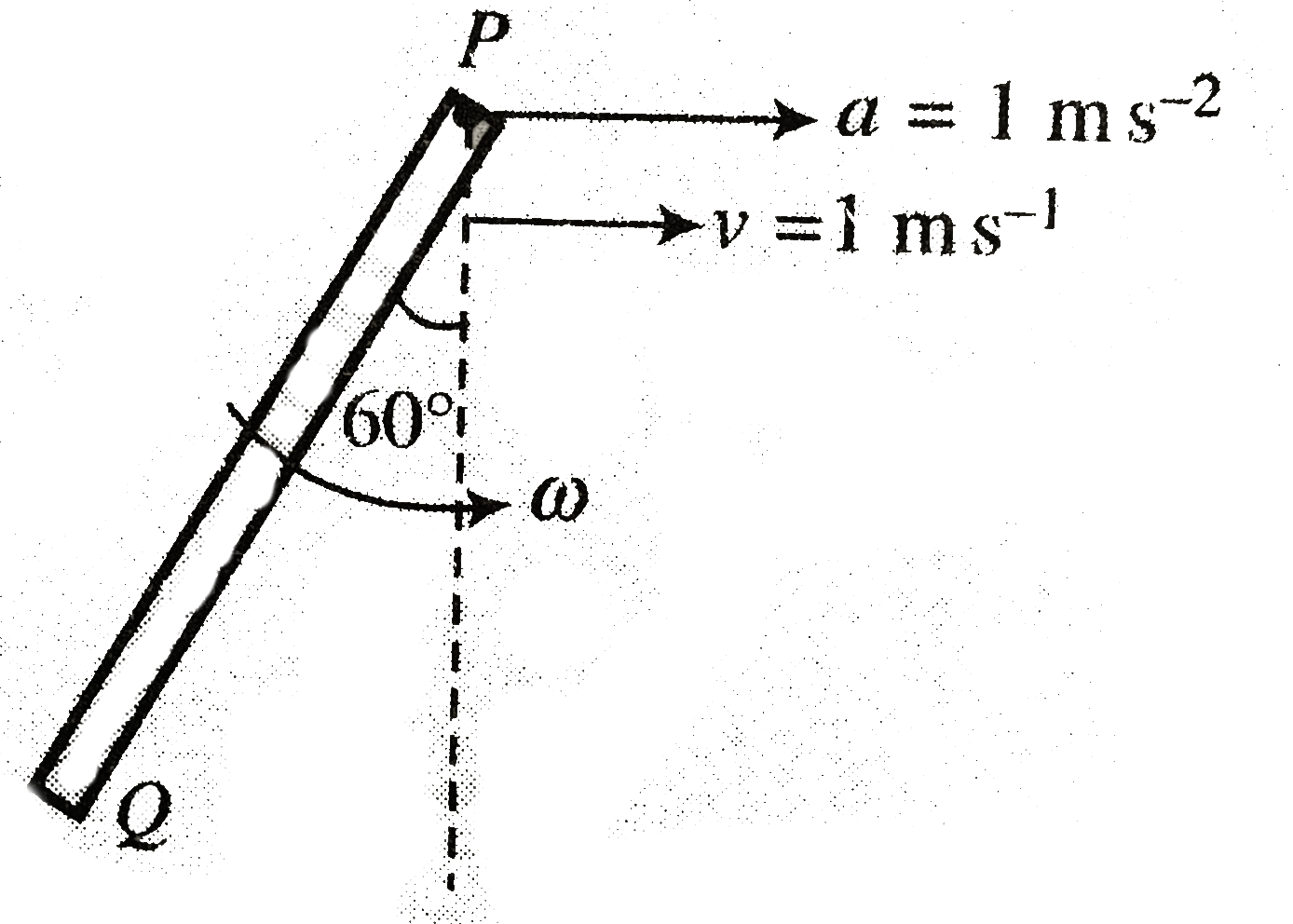
View SolutionA particle travels along the arc of a circle of radius r. Its speed depends on the distance travelled l as v=a√l where 'a' is a constant. The angle α between the vectors of net acceleration and the velocity of the particle is
View Solution
