A cricket ball of mass 160g is moving horizontally directly towards a batsman. Its speed just before it hits the bat is 30m/s. It leaves the bat at 40m/s at 90∘ to its original direction. Find the magnitude of the impulse (in N−s) imparted to the ball.
4.8
8
6.4
10
The correct Answer is:B
To solve the problem of finding the magnitude of the impulse imparted to the cricket ball, we will follow these steps:
1. Identify the Mass of the Ball:
The mass of the cricket ball is given as m=160g.
Convert this to kilograms:
m=1601000=0.16kg
2. Identify the Initial and Final Velocities:
The initial velocity of the ball before it hits the bat is vi=30m/s (horizontally).
The final velocity after it leaves the bat is vf=40m/s (perpendicular to the original direction).
3. Calculate the Initial Momentum:
The initial momentum pi is given by:
pi=m⋅vi=0.16kg⋅30m/s=4.8kg m/s
4. Calculate the Final Momentum:
The final momentum pf is given by:
pf=m⋅vf=0.16kg⋅40m/s=6.4kg m/s
5. Determine the Change in Momentum:
The change in momentum Δp is given by:
Δp=pf−pi
Since the initial and final velocities are perpendicular to each other, we can treat them as vectors and use the Pythagorean theorem to find the magnitude of the change in momentum:
Δp=√(pf)2+(pi)2=√(6.4)2+(4.8)2
Calculate:
Δp=√40.96+23.04=√64=8kg m/s
6. Impulse Imparted to the Ball:
The impulse J imparted to the ball is equal to the change in momentum:
J=Δp=8N s
Final Answer:
The magnitude of the impulse imparted to the ball is 8N s.
The mass of the cricket ball is given as m=160g.
Convert this to kilograms:
m=1601000=0.16kg
The initial velocity of the ball before it hits the bat is vi=30m/s (horizontally).
The final velocity after it leaves the bat is vf=40m/s (perpendicular to the original direction).
The initial momentum pi is given by:
pi=m⋅vi=0.16kg⋅30m/s=4.8kg m/s
The final momentum pf is given by:
pf=m⋅vf=0.16kg⋅40m/s=6.4kg m/s
The change in momentum Δp is given by:
Δp=pf−pi
Since the initial and final velocities are perpendicular to each other, we can treat them as vectors and use the Pythagorean theorem to find the magnitude of the change in momentum:
Δp=√(pf)2+(pi)2=√(6.4)2+(4.8)2
Calculate:
Δp=√40.96+23.04=√64=8kg m/s
The impulse J imparted to the ball is equal to the change in momentum:
J=Δp=8N s
The magnitude of the impulse imparted to the ball is 8N s.
Topper's Solved these Questions
- TEST PAPERBook:ALLENChapter:TEST PAPERExercise:PHYSICS
Explore 4 Videos
- TEST PAPERBook:ALLENChapter:TEST PAPERExercise:PHYSICS
Explore 4 Videos
- SIMPLE HARMONIC MOTIONBook:ALLENChapter:SIMPLE HARMONIC MOTIONExercise:Example
Explore 1 Video
- TEST PAPER 1Book:ALLENChapter:TEST PAPER 1Exercise:PHYSICS
Explore 11 Videos
Similar Questions
A bullet of mass 10−3kg strikes an obstacle and moves at 60∘ to its original direction. If its speed also changes from 20m/s to 10m/s. Find the magnitude of impulse acting on the bullet.
View SolutionA cricket ball of mass m is hitted at the angle 45∘ to the horizontal with velcoity v its kinetic energy at the topmost point is
View Solution
Knowledge Check
A ball of mass m travelling horiozntally with velocity v strikes a massive vertical wall and rebounds back along its original direction with no change in speed. What is the magnitude of the impulse delivered by the wall to the ball?
A0B12mvCmvD2mv
A particle of mass m strikes a wall with speed v at an angle 30∘ with the wall elastically as shown in the figure. The magnitude of impulse imparted to the ball by the wall is
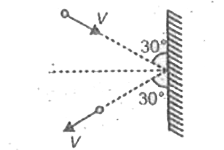
View SolutionA cricket ball of mass 150 kg is moving with a velocity of 12m/s and is hit by a bat so that ball is turned back with a velocity of 20m/s . The force of the blow acts for 0.01 s on the ball . Find the average force exerted by the bat on the ball.
View SolutionA cricket baal of mass 0.25 kg with speed 10 m/s collides with a bat and returns with same speed with in 0.01 s. The force acted on bat is
View SolutionA cricket ball of mass 150 g moving at a speed of 25 ms−1 is brought to rest by a player in 0.03 s. Find the average force applied by the player.
View SolutionA ball of mass 2 kg is projected horizontally with a velocity 20m/s from a building of height 15m. The speed with which body hits the ground is :
View SolutionA 5 kg body is fired vertically up with a speed of 200 m/s. Just before it hits the ground, its speed is 150 m/s. Over the entire trip,
View SolutionA ball of mass M moving with speed v collides perfectly inelastically with another ball of mass m at rest. The magnitude of impulse imparted to the first ball is
View Solution