Figure shows the interference pattern obtained ina double-slit experiment using light of wavelength 600 m. 1, 2, 3, 4 and 5 are maked on fiv fringes.
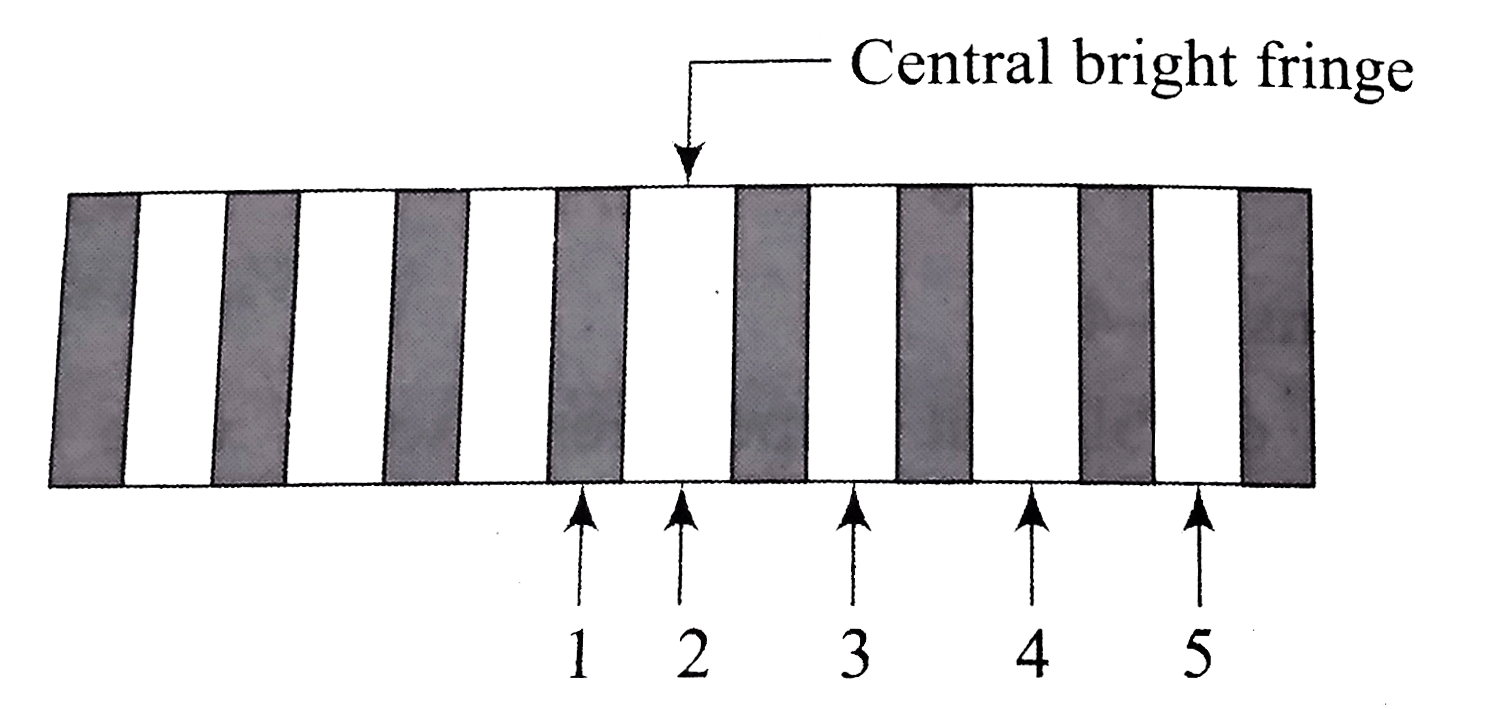
Let ΔXA and ΔXc represent path difference between waves interfering at 1 and 3, respectively. Then (|ΔXc|−|ΔXA|) is equal to
0
300 mn
600 nm
900 nm
The correct Answer is:B
CBF⇒ path difference =0
ΔXA→At 1
dark fringe having path difference =λ2
ΔXC→At 3,
bright fringes path difference =λ
∴(|ΔXC|−|ΔXA|)=(λ−λ2)=λ2=6002nm=300nm
Topper's Solved these Questions
- WAVE OPTICSBOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 4 A (Conceptual subjective Exercise)
9 Videos
- WAVE OPTICSBOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 4 B (Conceptual subjective Exercise)
8 Videos
- WAVE OPTICSBOOK - ALLENCHAPTER - WAVE OPTICSEXERCISE - Exercise 2 (Brain Teasures)
22 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLENCHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (J-A)
7 Videos
Similar Questions
_E01_080_Q01.png)
The figure shows the interfernece pattern obtained in double slit experiment using light of wavelength 600 nm.
Q. The third order bright fringe is
View Solution_E01_082_Q01.png)
The figure shows the interfernece pattern obtained in double slit experiment using light of wavelength 600 nm.
Q. Let ΔXA and ΔXC represent path differences between waves interering at 1 and 3 respectively then (|ΔXC|−(|ΔXA|) is equal to
View Solution_E01_081_Q01.png)
The figure shows the interfernece pattern obtained in double slit experiment using light of wavelength 600 nm.
Q. Which fringe results from a phase difference of 4π between the light waves incidenting from two slits?
View SolutionIn the adjacent figure 'P' is any interior point of the equilateral triangle ABC is side length 2 unit . If xa,xbandxc represent the distance of P from the sides BC, CA and AB respectively then xa+xb+xc is equal to
View SolutionThe base and the altitude of a triangle are (3x−4y) and (6x+5y) respectively. Find its area.
View SolutionIn a double slit experiment, at a certain point on the screen the path difference between the two interfering waves is 13rd of a wavelength. The ratio of the intensity of light at that point to that at the centre of a bright fringe is:
View SolutionIn a triangle ABC, if A is (1,2) and the equations of the medians through B and c are x+y=5 and x=4 respectively then B must be:
View SolutionIn a ΔABC the equation of the side BC is 2x−y=3 and its circumcentre and orthocentre are (2,4)and(1,2) respectively .
Circumradius of ΔABC is
View SolutionIn Young.s double slit interference experiment the wavelength of light used is 6000A0. If the path difference between waves reaching a point P on the screen is 1.5 microns, then at that point P
View SolutionThe area (in square units) of the triangle bounded by x = 4 and the lines y2−x2+2x=1 is equal to
View Solution
