The escape velocity for a planet from the surface is ve. A particle starts from rest at a large distance from the planet, reaches the planet only under gravitational attraction, and passes through a smooth tunned through its centre. Its speed at the centre of the planet will be
√1.5ve
ve√2
ve
zero
The correct Answer is:A
From mechanical energy conservation 0+0=12mv2−3GMm2R⇒v=√3GMR=√1.5ve
Topper's Solved these Questions
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 1 (Check your Grasp)
28 Videos
- GRAVITATIONBOOK - ALLENCHAPTER - GRAVITATIONEXERCISE - Exercise 2 (Brain Teasers)
27 Videos
- GEOMETRICAL OPTICSBOOK - ALLENCHAPTER - GEOMETRICAL OPTICSEXERCISE - subjective
14 Videos
- KINEMATICS-2DBOOK - ALLENCHAPTER - KINEMATICS-2DEXERCISE - Exercise (O-2)
46 Videos
Similar Questions
The escape velocity for a planet is ve. A particle starts from rest at a large distance from the planet, reaches the planet only under gravitational attraction, and passes through a smooth tunnel through its centre. Its speed at the centre of the planet will be
View Solution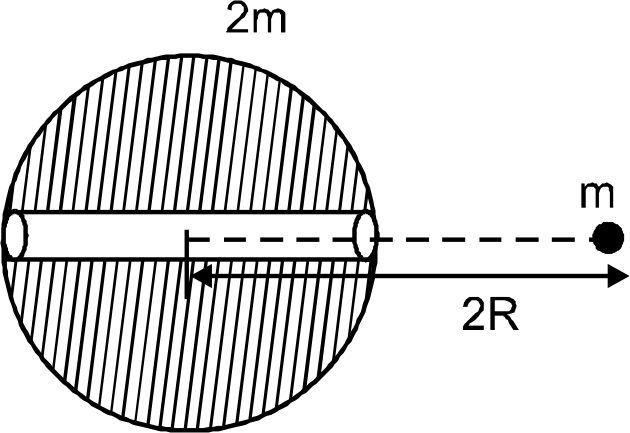
A solid spherical planet of mass 2m and radius 'R' has a very small tunnel along its diameter. A small cosmic particle of mass m is at a distance 2R from the centre of the planet as shown. Both are initially at rest, and due to gravitational attraction, both start moving toward each other. After some time, the cosmic particle passes through the centre of the planet. (Assume the planet and the cosmic particle are isolated from other planets)
View SolutionIf the angular velocity of a planet about its own axis is halved, the distance of geostationary satellite of this planet from the centre of the centre of the planet will become
View SolutionEscape velocity from a planet is ve. If its mass is increased to 16 times and its radius is increased to 4 times, then the new escape velocity would be
View SolutionThe escape velocity from the surface of the earth is Ve. The escape velcotiy from the surface of a planet whose mass and radius are three times those of the earth, will be
View SolutionA point P lies on the axis of a fixed ring of mass M and radius a, at a distance a from its centre C. A small particle starts from P and reaches C under gravitational attraction only. Its speed at C will be.
View SolutionA point P lies on the axis of a fixed ring of mass M and radius a, at a distance a from its centre C. A small particle starts from P and reaches C under gravitational attraction only. Its speed at C will be.
View SolutionStatement I: In free space a uniform spherical planet of mass M has a smooth narrow tunnel along its diameter. This planet and another superdense small particle of mass M start approaching towards each other from rest under action of their gravitational forces. When the particle passes through the centre of the planet, sum of kinetic energies of both the bodies is maximum.
Statement II: When the resultant of all forces acting on a particle or a particle like object (initially at rest) is constant in direction, the kinetic energy of the particle keeps on increasing.
View SolutionThe escape Velocity from the earth is 11.2Km/s. The escape Velocity from a planet having twice the radius and the same mean density as the earth, is :
View SolutionA particle is thrown with escape velocity ve from the surface of earth. Calculate its velocity at height 3 R :-
View Solution
