What is the direction of electric field at a point on the equitorial line of electric dipole ? Solution in Punjabi

Video Solution

Text Solution

Verified by Experts

Electric Potential at any point due to an Electric Dipole : Consider an electric dipole AB having charge - 9 at point A and charge + q at point B and 2a is its dipole length. Let O be the centre of the dipole and P be any point at a distance from its centre, where electric potential due to the dipole is to be determined. Let $∠ P O B = θ$ .
The potential at point P due to charge -q,
and the potential at point P due to charge +q,
$V_{2} = \frac{1}{4 π \in_{0}} . \frac{q}{P B}$
Therefore, net potential at point P due to the dipole,
$V_{2} = V_{1} + V_{2} = - \frac{1}{4 π \in_{0}} . \frac{q}{P A} + \frac{1}{4 π \in_{0}} . \frac{q}{P B}$
or $V = \frac{1}{4 π \in_{0}} . q [\frac{1}{P B} - \frac{1}{P A}] ... ... (i)$

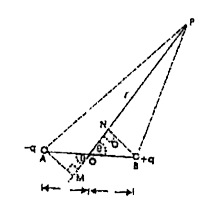
To find PB and PA, draw BN perpendicular to OP and AM perpendicular to PQ after producing it. From right angled AAMO, we have
$\cos θ = \frac{O M}{O A} = \frac{O M}{a} or O M = a \cos θ$
In case the length of the dipole is very small as compared to distance r, then $P A \approx P M = P O + O M = r \div a \cos$
Similarly, it can be obtained that
$P B = r - a \cos θ$
In the equation (1), substituting for PA and PB, we have
$V = \frac{1}{4 π \in_{0}} . q [\frac{1}{r - a \cos θ} - \frac{1}{r + a \cos θ}]$
$= \frac{1}{4 π \in_{0}} . q [\frac{r + a \cos θ - r + a \cos θ}{r^{2} - a^{2} \cos^{2} θ}]$
$= \frac{1}{4 π \in_{0}} . q [\frac{2 a \cos θ}{r^{2} - a^{2} \cos^{2} θ}]$
Since q (20) = P, the electric dipole moment of the dipole, the above equation becomes
$V = \frac{1}{4 π \in_{0}} . \frac{P \cos θ}{(r^{2} - a^{2} \cos^{2} θ)} ... ... (2)$
The equation (2) gives electric potential due to the dipole at a distance r from its centre in a direction making an angle with the dipole.
Special cases : Let us find the electric potential due to the dipole in the following two cases :
When point P lies on the axial line of the dipole :
In such a case, $θ = 0^{\circ}$ and ${\cos 0}^{\circ} = 1$
Therefore, the equation (2) gives
$V = \frac{1}{4 π \in_{0}} . \frac{P {\cos 0}^{\circ}}{(r^{2} - a^{2} \cos^{2} 0^{\circ})}$
$V = \frac{1}{4 π \in_{0}} . \frac{P (1)}{(r^{2} - a^{2})}$
$V = \frac{1}{4 π \in_{0}} . \frac{P}{(r^{2} - a^{2})}$
When point P lies on the equitorial line of the dipole :
In such a case, theta=90^(@) $. and$ cos90^(@)=0
From equation (2)
y=0

|

Updated on:21/07/2023

Class 12 PHYSICS ELECTROSTATIC POTENTIAL

Topper's Solved these Questions

Similar Questions

BETTER CHOICE PUBLICATION-ELECTROSTATIC POTENTIAL-LONG ANSWERTYPE QUESTIONS (5/6 Marks Questions)

Home
Ask Doubt
Profile