The time period of a body undergoing simple harmonic motion is given by , where p is the pressure, D is density and S is surface tension. The values of a, b and c respectively are
The correct Answer is:D
To solve the problem, we need to determine the values of a, b, and c in the equation T=paDbSc, where T is the time period, p is the pressure, D is the density, and S is the surface tension. We will use dimensional analysis to find these values.
Step 1: Write down the dimensions of each physical quantity.
1. Time period T: The dimension of time is given by:
(T)=T1
2. Pressure p: Pressure is defined as force per unit area. The dimension of force is MLT−2 and area is L2. Therefore, the dimension of pressure is:
(p)=MLT−2L2=ML−1T−2
3. Density D: Density is mass per unit volume. The dimension of volume is L3. Therefore, the dimension of density is:
(D)=ML3=ML−3
4. Surface tension S: Surface tension is defined as force per unit length. Therefore, the dimension of surface tension is:
(S)=MLT−2L=ML−1T−2
Step 2: Substitute the dimensions into the equation.
Now we substitute the dimensions into the equation T=paDbSc:
(T)=(p)a(D)b(S)c
Substituting the dimensions we found:
T1=(ML−1T−2)a(ML−3)b(ML−1T−2)c
Step 3: Expand the right-hand side.
Expanding the right-hand side gives:
T1=Ma+b+cL−a−3b−cT−2a−2c
Step 4: Set up equations by equating dimensions.
Now, we equate the powers of M, L, and T from both sides:
1. For M:
a+b+c=0(Equation 1)
2. For L:
−a−3b−c=0(Equation 2)
3. For T:
−2a−2c=1(Equation 3)
Step 5: Solve the equations.
From Equation 1:
c=−a−b
Substituting c into Equation 2:
−a−3b−(−a−b)=0⟹−a−3b+a+b=0⟹−2b=0⟹b=0
Now substituting b=0 back into Equation 1:
a+0+c=0⟹c=−a
Substituting b=0 into Equation 3:
−2a−2(−a)=1⟹−2a+2a=1⟹0=1 (not possible)
We need to re-evaluate our equations.
From Equation 3:
−2a−2c=1⟹c=−a−12
Substituting c into Equation 1:
a+b−a−12=0⟹b=12
Now substituting b=12 into Equation 1:
a+12+c=0⟹c=−a−12
Final values:
After solving the equations, we find:
- a=−32
- b=12
- c=1
Conclusion:
Thus, the values of a, b, and c are:
−32,12,1
(T)=T1
(p)=MLT−2L2=ML−1T−2
(D)=ML3=ML−3
(S)=MLT−2L=ML−1T−2
(T)=(p)a(D)b(S)c
Substituting the dimensions we found:
T1=(ML−1T−2)a(ML−3)b(ML−1T−2)c
T1=Ma+b+cL−a−3b−cT−2a−2c
a+b+c=0(Equation 1)
−a−3b−c=0(Equation 2)
−2a−2c=1(Equation 3)
c=−a−b
−a−3b−(−a−b)=0⟹−a−3b+a+b=0⟹−2b=0⟹b=0
a+0+c=0⟹c=−a
−2a−2(−a)=1⟹−2a+2a=1⟹0=1 (not possible)
−2a−2c=1⟹c=−a−12
a+b−a−12=0⟹b=12
a+12+c=0⟹c=−a−12
After solving the equations, we find:
- a=−32
- b=12
- c=1
Thus, the values of a, b, and c are:
−32,12,1
Topper's Solved these Questions
Similar Questions
The x-t graph of a particle undergoing simple harmonic motion is shown below. The acceleration of the particle at t=23s is

View SolutionA gas bubble, from an explosion under water, oscillates with a period proportional to PadbEc, where P is the static pressure , d is the density and E is the total energy of the explosion. Find the values of a,b and c.
View Solution
Knowledge Check
The period T of a soap bubble under SHM is given by T=PaDbSc, where P is pressure, D, is density and S is surface tension. Then the values of a,b and c are
A−32,12,1B−1,−2,3C13,−32,−1/2D1,2,32For the equation F=Aavbdc where F is force, A is area, v si velocity and d is density with the dimensional anaysis gives the following values for the exponents.
A1,2,1B2,1,1C1,1,2D0,1,1,A gas bubble, from an explosion under water, oscillates with a period proportional to PadbEc, where P is the static pressure , d is the density and E is the total energy of the explosion. Find the values of a,b and c.
Aa=32,b=−13,c=12Ba=−56,b=13,c=12Ca=−56,b=12,c=13Da=32,b=−13,c=12
A gas bubble from an explosion under water oscillates with a period T proportional to PadbEc, where P is the pressure, d is density of water and E is the total energy of the explosion. Find the value of a,b and c.
View SolutionA gas bubble , from an explosion under water , oscillates with a period T proportional in PaDbEc , where p is the static pressure , d is the density of water and E is the total energy of the explosion . Find the value ofa,b andc .
View SolutionIf the period 'T' of a drop under surface tension 's' is given by T=√darbSc where d is the density , r is the radius of the drop. If a=1,c=−1 then the value of b is
View SolutionThe x−t graph of a particle undergoing simple harmonic motion is shown below. The accelertion of the particle at t=4/3s is
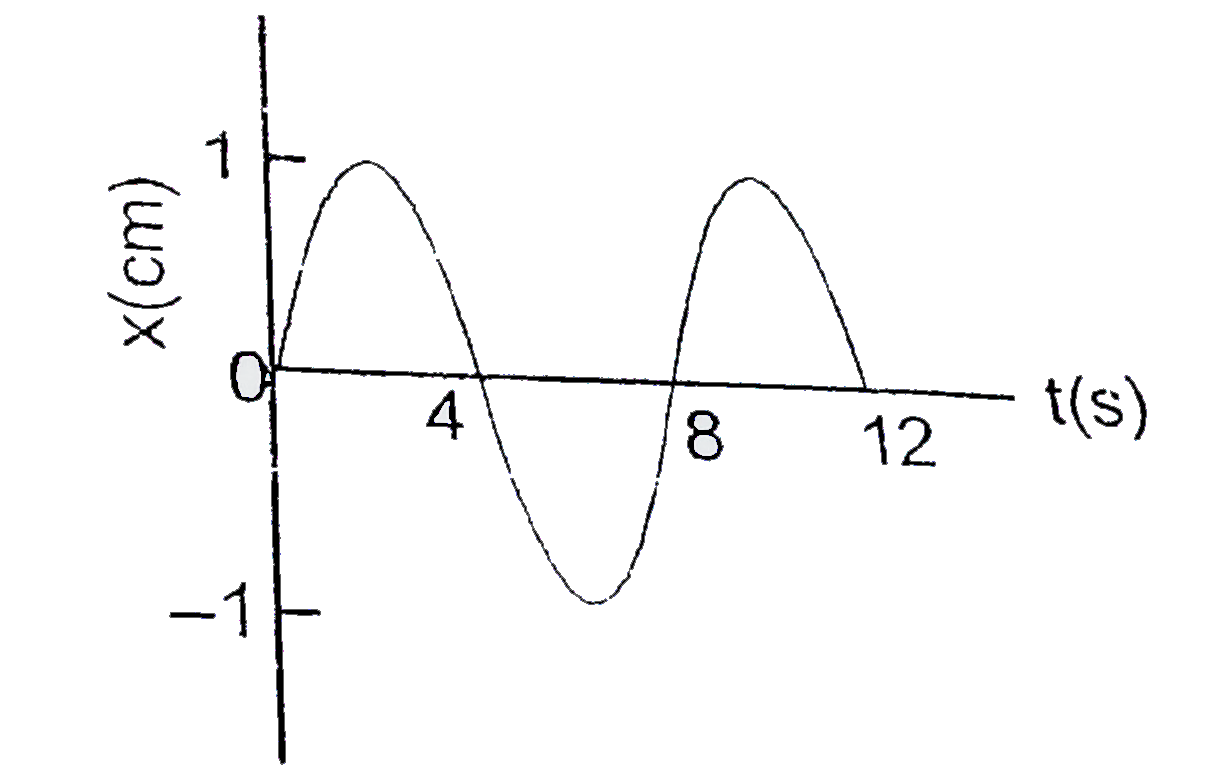
View SolutionThe x-t graph of a particle undergoing simple harmonic motion is shown below. The acceleration of the particle of t=4/3s is
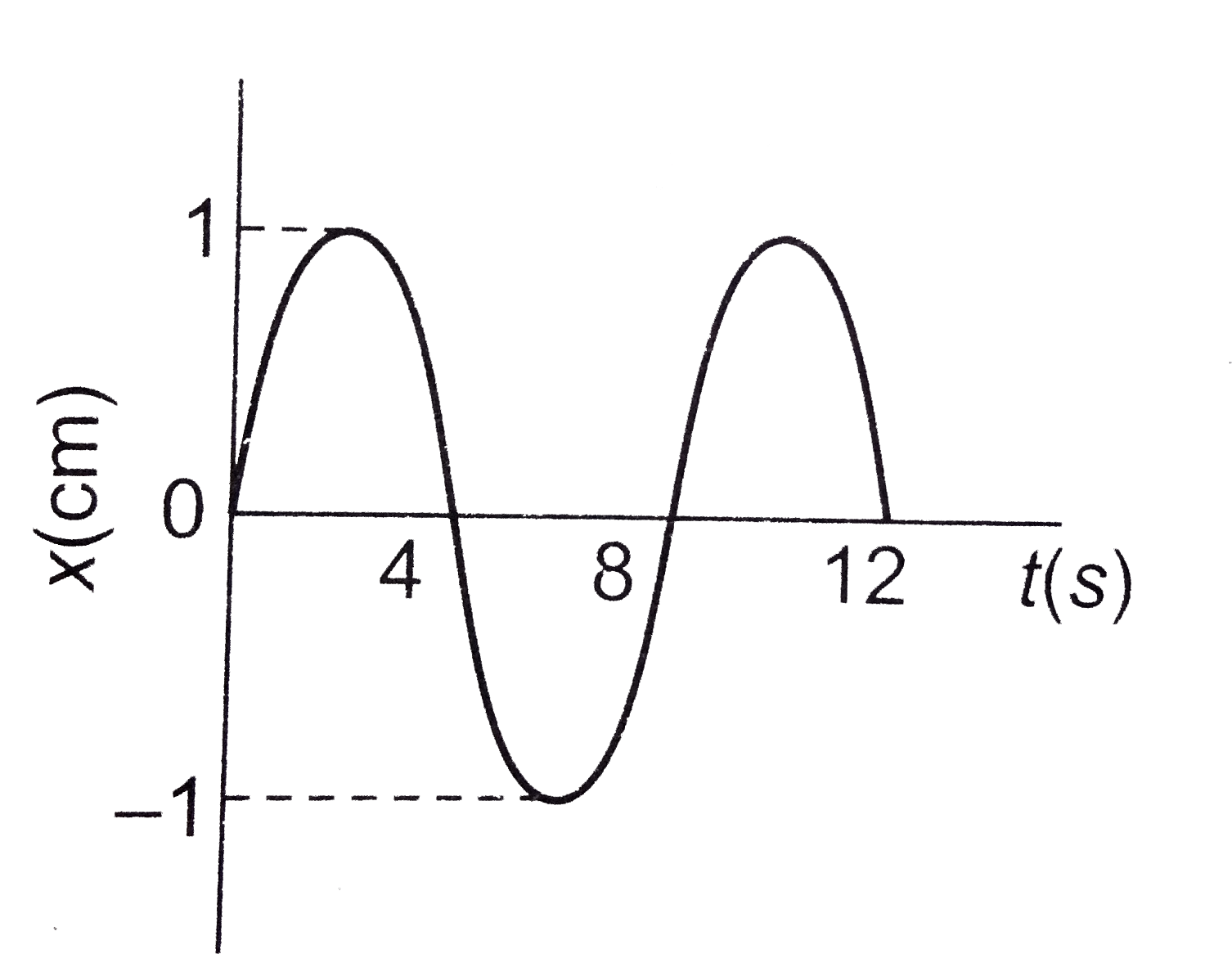
View Solution
