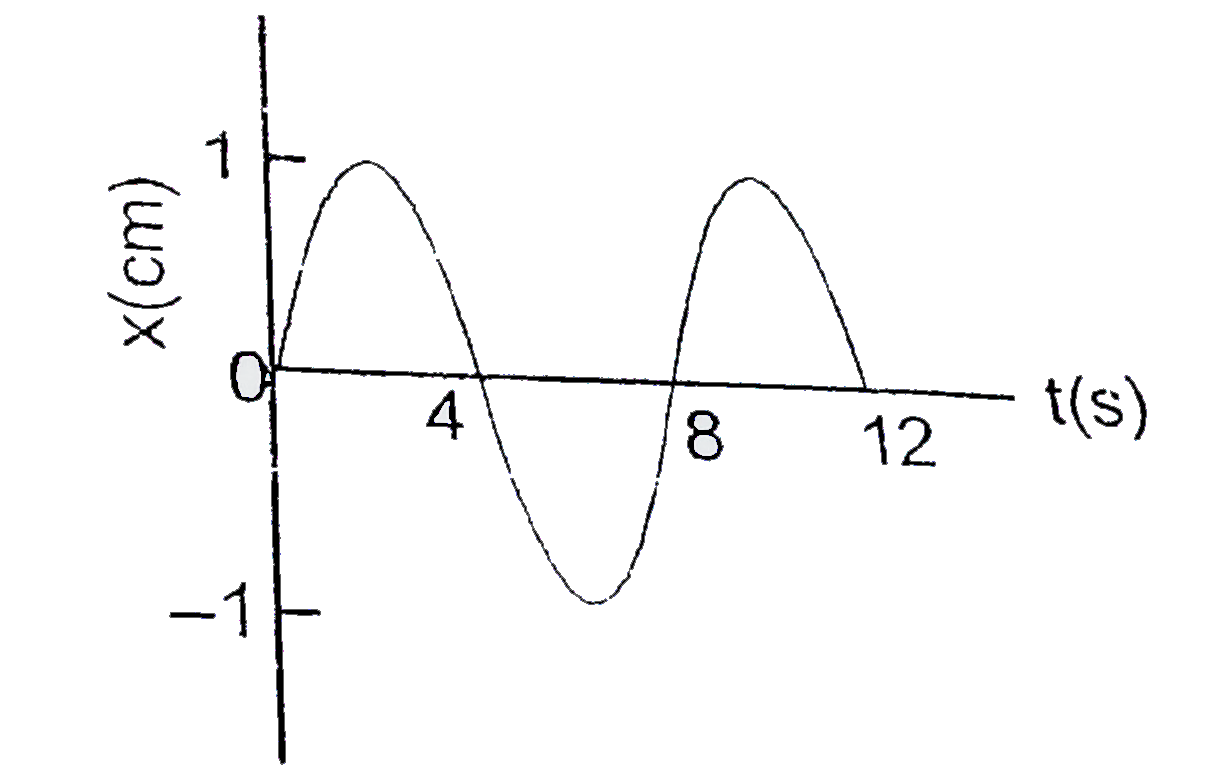
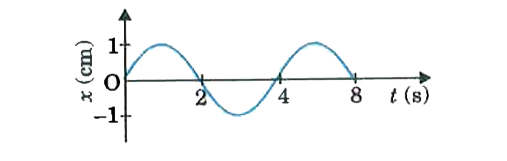
The x−t graph of a particle undergoing simple harmonic motion is shown below. The accelertion of the particle at t=4/3s is
A
√332π2cm/s2
B
−π232cm/s2
C
π232cm/s2
D
−√332π2cm/s2
Video Solution
Text Solution
The correct Answer is:D
From graph
T=8 second, A=1cm,x=Asinωt.=1sin(2π)8t.
a=−ω2x=−(2π8)2sin(2π8)tcm/s2
At, t=43 second, a=−(2π8)2sinπ3=−√3π232cm/s2
|
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 3, PART - II
17 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - IV
8 Videos
- SEMICONDUCTORSBOOK - RESONANCECHAPTER - SEMICONDUCTORSEXERCISE - Exercise 3
88 Videos
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - FST-3
30 Videos
Similar Questions
Knowledge Check
The x-t graph of a particle undergoing simple harmonic motion is shown below. The acceleration of the particle of t=4/3s is
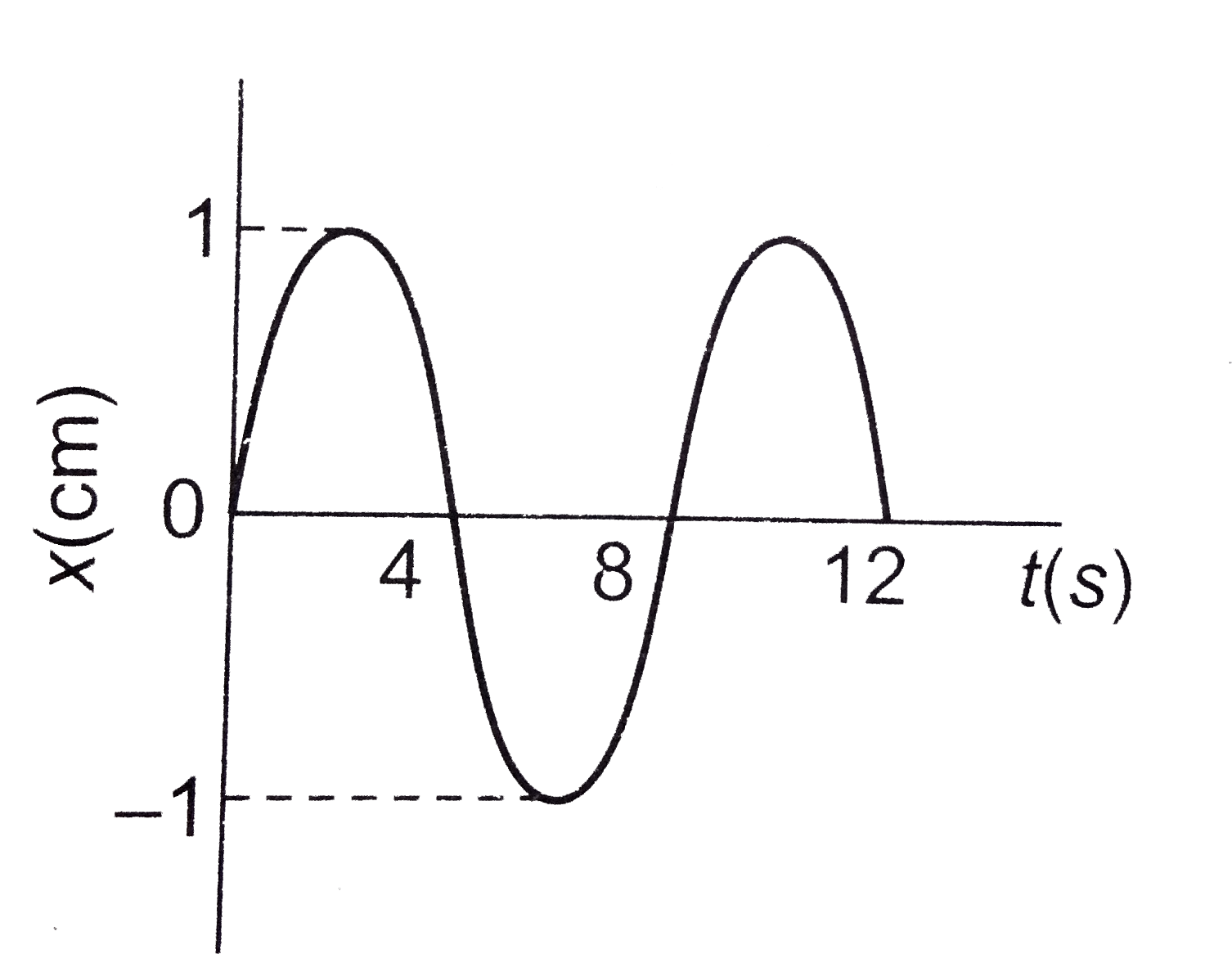 A√332π2cm/s2B−π232cm/s2Cπ232cm/s2D−√332π2cm/s2
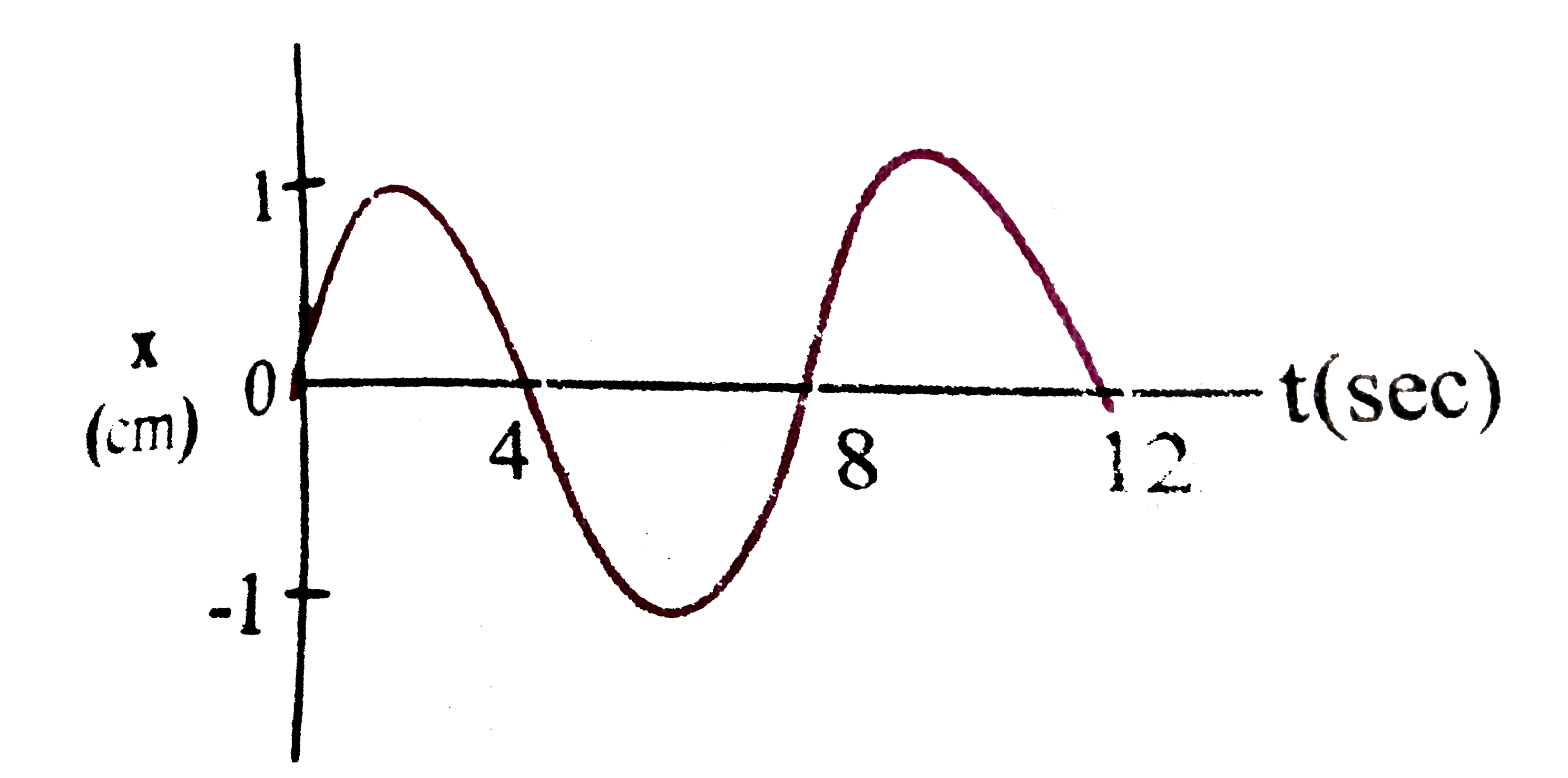
A√332π2cm/s2B−π232cm/s2Cπ232cm/s2D−√332π2cm/s2The x−t graph of a particle undergoing simple harmonic motion is shown in figure. Acceleration of particle at t=4/3s is
 A√332π2cm/s2B−π232cm/s2Cπ232cm/s2D−√332π2cm/s2
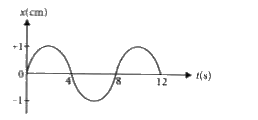
A√332π2cm/s2B−π232cm/s2Cπ232cm/s2D−√332π2cm/s2The x-t graph of a particle undergoing SHM is as shown in figure. The acceleration of the particle at t=2/3 is
 A√332π2cms−2B−π232cms−2Cπ232cms−2D−√332π2cms−2
A√332π2cms−2B−π232cms−2Cπ232cms−2D−√332π2cms−2
The displacement vs time graph of a particle undergoing S.H>M. Is shown below . The acceleration of the particle at t=2/3s is
View SolutionThe velocity of a particle executing simple harmonic motion is
View SolutionWhile a particle executes linear simple harmonic motion
View SolutionThe phase (at a time t) of a particle in simple harmonic motion tells
View SolutionIf a particle is executing simple harmonic motion, then acceleration of particle
View Solution

