A monochromatic beam of light is incident at the interface of two materials of refractive index n1 and n2 as shown . If n1>n2 and θC is the critical angle then which of the following statements is NOT true ?
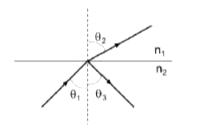
θ1=θ3 for all value of θ1
cosθ2 is imagimary for θ1>θc
cosθ2=0 for θ1=θc
cosθ3 is imaginary for θ1=θc
The correct Answer is:D
n1>n2
this means light is going from rarer to denser medium so θ2 will always be less than θ1
n2sinθ1=n1sinθ2
so cos(θ2) will never be imaginary and also θ2 can .t be 90∘
In question incorrect options are askd
∴(B,C,D)
Topper's Solved these Questions
Similar Questions
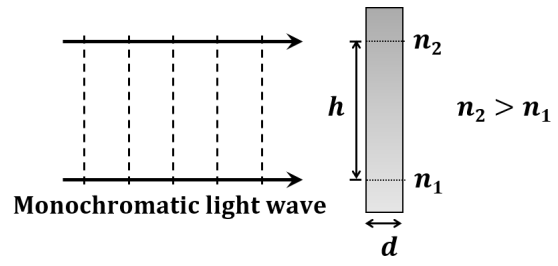
A monochromatic light wave is incident normally on a glass slab of thickness d, as shown in the figureThe refractive index of the slab increases linearly from n1 to n2 over the height h. Which of the following statement(s) is (are) true about the light wave emerging out of the slab?
View SolutionA monochromatic light is incident on the plane interface AB between two media of refractive indices μ1 and (μ2>μ1) at an angle of incidence θ as shown in Fig.
The angle θ is infinitesimally greater than the critical angle for the two media so that total internal reflection takes place. Now, if a transparent slab DEFG of uniform thickness and of refractive index μ3 is introduced on the interface (as shown in the figure), show that for any value of μ3 all light will ultimately be reflected back into medium II.

View Solution
Knowledge Check
A monochromatic beam of light of wavelength λ and frequency v goes from vacum to a medium of refractive index n. How do the wavelength and frequency of light change ?
Awavelength becomes λn and frequency nvBwavelength becomes nλ and frequency becomes vnCwavelength and frequency do not changeDwavelength becomes λn and frequency does not changeIn the diagram shown, light is incident on the interface between media 1 (refractive index n1) and 2 (refractive index n2) at angle slightly greater than the critical angle, and is totally reflected. The light is then also totally reflected at the interface between media 1 and 3 (refractive index n3), after which it travels in a direction opposite to its initial direction, The media must have a refractive indices such that.
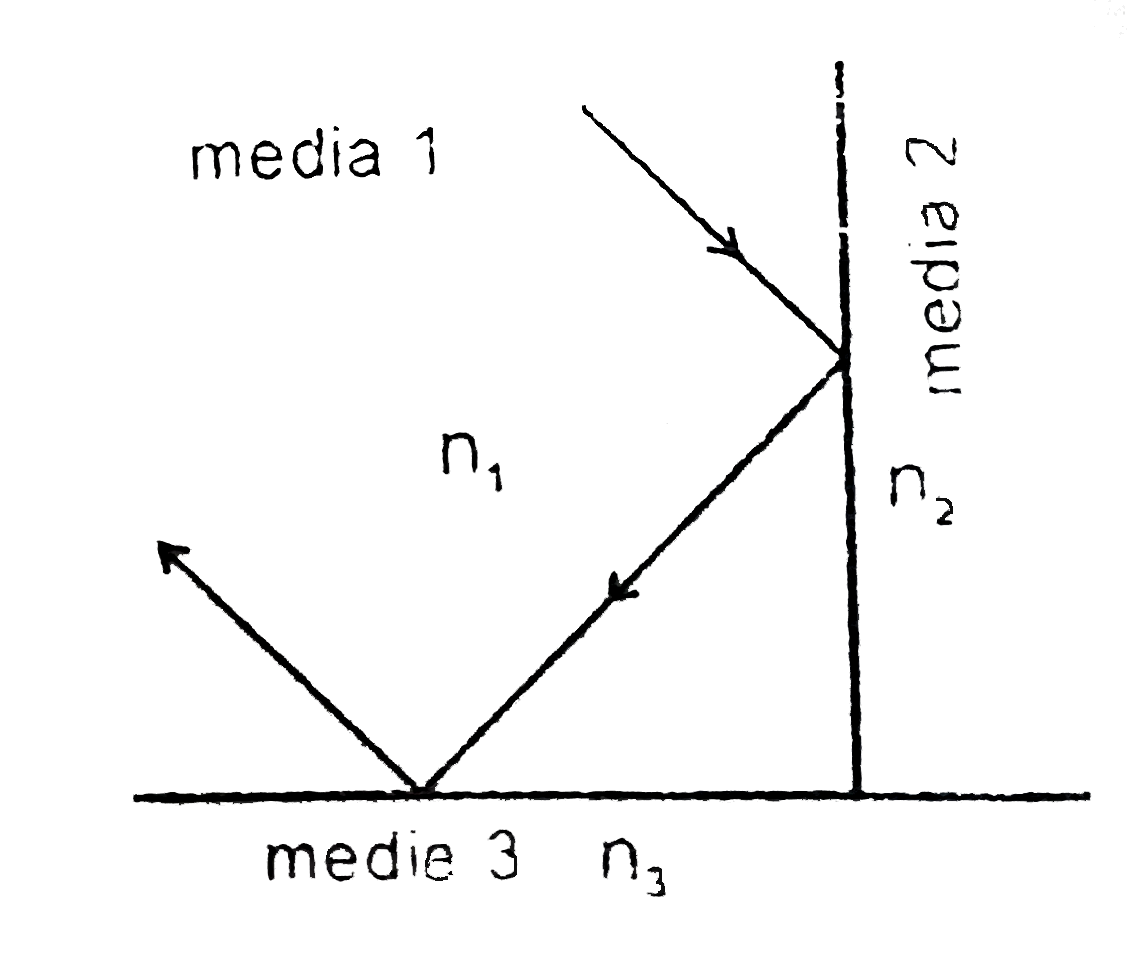 .An1<n2<n3Bn21−n23>n22Cn21−n22<n23Dn21−n22>n23
.An1<n2<n3Bn21−n23>n22Cn21−n22<n23Dn21−n22>n23A beam of light propagating in medium A with index of refraction n(A) passes across an interface into medium B with index of refraction n(B). If v(A) and v(B) are the speeds of light in A and B respectively. Then which of the following is true?
A(a) v(A)>v(B) and n(A)>n(B)B(b) v(A)>v(B) and n(A)<n(B)C(c) v(A)<v(B) and n(A)>n(B)D(d) v(A)<v(B) and n(A)<n(B)
A ray of light is incident on the left vertical face of glass cube of refractive index n2, as shown in figure. The plane of incidence is the plane of the page, and the cube is surrounded by liquid (refractive index=n1). What is the largest angle of incidence θ1 for which total internal reflection occurs at the top surfaces?

View SolutionUnpolarised light is incident on a plane surface of glass of glass of refractive index n at angle i. If the reflected light gets totally polarised, write the relation between the angle i and refractive index n.
View Solution(a) In Fig. 32-19a, a beam of monochromatic light reflects and refracts at point A on the interface between material 1 with index of refraction n1 = 1.33 and material 2 with index of refraction n2=1.77. The incident beam makes an angle of 50∘) with the interface. What is the angle of reflection at poinl A ? What is the angle of refraction there?
View SolutionA transparent slab of thickness d has a refractive index n(z) that increases with z. Here z is the vertical distance inside the slab, measured from the top. The slab is placed between two media with uniform refractive indices n1andn2(>n1), as shown in the figure. A ray of light is incident with angle θi, from medium 1 and emerges in medium 2 with refraction and θf, with a lateral displacement l.
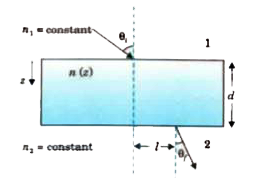
Which of the following statement(s) is (are) true ?
View SolutionTwo optical media of refractive indices n1 and n2 contain x and y waves of the same colour in the same thickness. Then their relative refractive index n1/n2
View Solution
