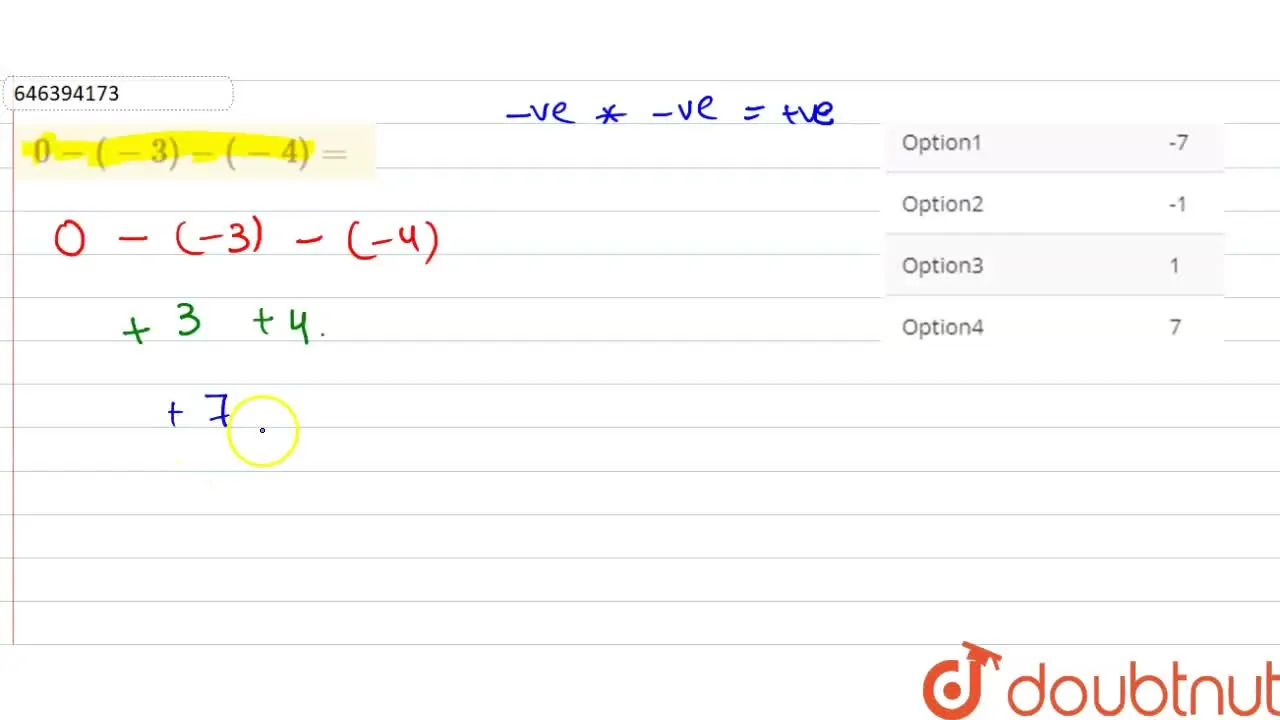
0−(−3)−(−4)=
-7
-1
1
7
Step by step video & image solution for 0-(-3)-(-4)= by Maths experts to help you in doubts & scoring excellent marks in Class 6 exams.
Related Playlists
Similar Questions
If A×B={(−2,3),(−2,4),(0,3),(0,4),(3,3),(3,4)}, find A and B.
View SolutionPlot the points A(0,4),B(−3,0),C(0,−4),D(3,0) and name the figure ABCD
View Solution(0.000729)−3/4×(0.09)−3/4=
View SolutionIf A=⎡⎢⎣033−30−4−340⎤⎥⎦andB=⎡⎢⎣xyz⎤⎥⎦, find the mastrix B'(AB)
View SolutionWhat is the value of (0.7)3−(0.4)3(0.7)2+0.7×0.4+(0.4)2 ?
View SolutionThe range of f(x)=cot^(-1)(log_(1/2)(x^(4)-2x^(2)+3)) is ( (pi)/(2) (3 pi)/(4) 0 (3 pi)/(4) pi) (0 (3 pi)/(4)
View SolutionIf the equationax2+2bx−3c=0 has non real roots and < a+b then c is always1)<0(2)>0(3)≥0(4)zero
View Solution(34)0= ?
View SolutionLet A+2B=⎡⎢⎣32−3104312⎤⎥⎦and−A−B=⎡⎢⎣103−141321⎤⎥⎦. Find A and B.
View SolutionFind the radius of the sphere through the points (0,5,0),(4,3,0),(4,0,3)and(0,4,3)
View Solution